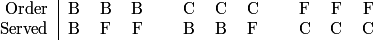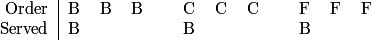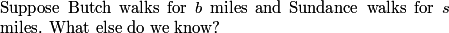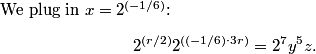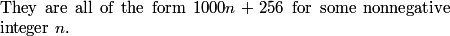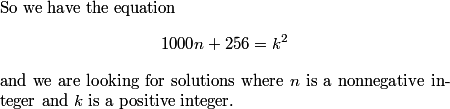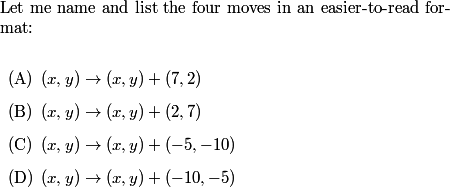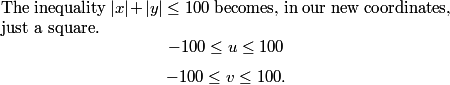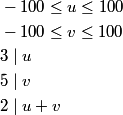Art of Problem Solving
AoPS Online AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

New classes are starting soon - secure your spot today!

2012 AIME I Math Jam

Go back to the Math Jam Archive

AoPS instructors discuss all 15 problems of the 2012 AIME I.

Copyright © 2024 AoPS Incorporated. This page is copyrighted material. You can view and print this page for your own use, but you cannot share the contents of this file with others.

Display all student messages • Show few student messages • Hide student messages

Facilitator: Dave Patrick

DPatrick 2012-03-17 19:00:17
Welcome to the 2012 AIME I Math Jam!
DPatrick 2012-03-17 19:00:22
I'm Dave Patrick, and I'll be leading our discussion tonight.
DPatrick 2012-03-17 19:00:30
Before we get started I would like to take a brief moment to explain our virtual classroom to those who have not previously participated in a Math Jam or one of our online classes.
DPatrick 2012-03-17 19:00:37
The classroom is moderated, meaning that students can type into the classroom, but these comments will not go directly into the room.  These comments go to the instructors, who may choose to share your comments with the room.
DPatrick 2012-03-17 19:00:46
This helps keep the class organized and on track.  This also means that only well-written comments will be dropped into the classroom, so please take time writing responses that are complete and easy to read.
DPatrick 2012-03-17 19:00:57
As usual for our Math Jams, there are a lot of students here!  As I said, only a relatively small fraction of the well-written comments will be passed to the entire group.  Please do not take it personally if your comments do not get posted, and please do not complain about it.  I expect this Math Jam to be much larger than our typical class, so please be patient with me---there are quite a few of you here tonight!!
DPatrick 2012-03-17 19:01:11
Also, we won't be going through the math quite as thoroughly as we do in our classes -- I can't teach all the necessary material for every problem as we go.  Another difference between tonight and our regular online classes is that it is very unlikely that we'll be able to answer every single question you ask.  We usually do in our classes, but we have a large number of students tonight!  So, please go ahead and ask questions, but also please understand if we aren't able to answer them all!
DPatrick 2012-03-17 19:01:25
We do have two teaching assistants with us tonight to help answer your questions: Carl Lian (CatalystOfNostalgia) and David Wise (MathWise).
DPatrick 2012-03-17 19:01:37
They can answer questions by whispering to you or by opening a window with you to chat 1-on-1.  However, due to the large size of the session tonight, they may not be able to get to you right away (or at all).  Repeating your question over and over is more likely to annoy us than to get it answered faster, so please, just ask your question once and be patient, and please understand that we may not be able to answer all the questions tonight.
ksun48 2012-03-17 19:01:57
Are we doing #15?
strategist 2012-03-17 19:01:57
Are we going to go through all 15 questions?
DPatrick 2012-03-17 19:02:04
Yes, we're going to do all 15 problems, in orer.
DPatrick 2012-03-17 19:02:05
...order.
DPatrick 2012-03-17 19:02:13
Please also remember that the purpose of this Math Jam is to work through the solutions to AIME problems, and not to merely present the answers.  "Working through the solutions" includes discussing problem-solving tactics.  Also on occasion we may stop to prove things that you wouldn't necessary need to prove while doing the contest.  So please, when a question is posted, do not simply respond with the final answer.  That's not why we're here.  We're going to work through the problems step-by-step, and comments that skip key steps or jump ahead in the problem, without providing explanation or motivation, won't be acknowledged.
DPatrick 2012-03-17 19:02:42
Again, if you have any questions, feel free to ask.  One of our assistants will try to help you as soon as they can.
DPatrick 2012-03-17 19:02:47
With that, let's get started!
DPatrick 2012-03-17 19:02:55
DPatrick 2012-03-17 19:03:02
(Note that I'll always put the current problem statement up at the top of the window.  You can resize the region at the top of the window by dragging the gray horizontal bar.)
EricMathPath09 2012-03-17 19:03:19
Use the law of divisibility by 4.
DPatrick 2012-03-17 19:03:25
Right.  How we can tell if a 3-digit number is a multiple of 4?
aopsmomisawesome 2012-03-17 19:03:39
ignore the first digit
FalconPower 2012-03-17 19:03:40
last two digits divisble by 4
fireonice 2012-03-17 19:03:40
if the last two digits are divisible by 4
binmu 2012-03-17 19:03:40
last two digits divisible by 4
strategist 2012-03-17 19:03:44
two cases: b is odd or b is even
strategist 2012-03-17 19:03:44
cases where b is odd, b is even
DPatrick 2012-03-17 19:04:06
We only need to look at the last two digits.  And it's possible to divide this in the cases where b is even and where b is odd.
19oshawott98 2012-03-17 19:04:19
b odd=a/c being 2 or 6
Iggy Iguana 2012-03-17 19:04:29
if b even: 4 and 8
aopsmomisawesome 2012-03-17 19:04:35
If b is odd, we can last digithave 2 or 6; if b is even, our last digit can be 4 or 8 (no zero)
DPatrick 2012-03-17 19:04:46
Yes.  The last two digits must be e0, e4, e8, o2, or o6, where "e" is any even digit and "o" is any odd digit.
DPatrick 2012-03-17 19:04:51
But we can throw away e0, because we can't have the last digit be 0.
DPatrick 2012-03-17 19:04:57
So the possibilities are e4, e8, o2, and o6.
Relativity1618 2012-03-17 19:05:23
for each be there are 2 possible values of a and c
Iggy Iguana 2012-03-17 19:05:23
so there are 2 choices for each
thecmd999 2012-03-17 19:05:23
so for every b, there are 2*2=4 different a and c; since there are 10 possible b's, the answer is 4*10=40
DPatrick 2012-03-17 19:05:32
For any possible middle digit b, there are 2 choices for a and 2 choices for c that work.  (If b is even, 4 and 8 work; if b is odd, 2 and 6 work.)
DPatrick 2012-03-17 19:05:44
So, for each of the 10 choices of middle digit b, we have 2 choices for a and 2 choices for c.
strategist 2012-03-17 19:05:52
then there are 2 * 2 * 10
Relativity1618 2012-03-17 19:06:01
2*2*10=40
va2010 2012-03-17 19:06:01
so 2*2*10=40
DPatrick 2012-03-17 19:06:04
googol.plex 2012-03-17 19:06:22
Do you have to put it with the 0 in front?
eccfcco15 2012-03-17 19:06:22
why 040 instead of 40
DPatrick 2012-03-17 19:06:40
I put that to get you in the habit of not forgetting to bubble in the first 0 (on the AIME answer form when you're taking the contest)!
DPatrick 2012-03-17 19:06:54
strategist 2012-03-17 19:07:18
836 - 715 = 121
19oshawott98 2012-03-17 19:07:18
836-715
leecchh 2012-03-17 19:07:20
The toal increase is 836-715=121
DPatrick 2012-03-17 19:07:28
Right.  We see that the new sequence's sum is 836 - 715 = 121 greater.
DPatrick 2012-03-17 19:07:37
On the other hand, we get to the new sequence by adding 1, 3, 5, etc.
ABCDE 2012-03-17 19:07:55
The sum of the first k odd numbers is k^2
19oshawott98 2012-03-17 19:07:55
1+3+5...+21=121
va2010 2012-03-17 19:07:55
121 means 1+3......+21
mcdonalds106_7 2012-03-17 19:07:55
so their must be 11 terms since 11^2=121
DPatrick 2012-03-17 19:08:08
DPatrick 2012-03-17 19:08:24
DPatrick 2012-03-17 19:08:33
DPatrick 2012-03-17 19:08:38
This means that the sequence has 11 terms.
jeff10 2012-03-17 19:09:04
Then do 715/11=65, so that's the middle term.
19oshawott98 2012-03-17 19:09:04
715/11=65
Iggy Iguana 2012-03-17 19:09:04
so the middle integer is 715/11=65
19oshawott98 2012-03-17 19:09:04
65 is middle term because 715/11=65
prezcoin 2012-03-17 19:09:04
first + middle + last terms = 3 * middle term
DPatrick 2012-03-17 19:09:19
Exactly.  We finish by using a couple of facts about arithmetic series.
DPatrick 2012-03-17 19:09:26
DPatrick 2012-03-17 19:09:42
Since the sum of the sequence is 715 and there are 11 terms, the average term is 715/11 = 65.
DPatrick 2012-03-17 19:10:02
The middle term equals the average term, 65.
KeepingItReal 2012-03-17 19:10:09
first + last term = 130
DPatrick 2012-03-17 19:10:12
The first and last terms sum to twice the average term, or 2*65 = 130.
andrewjjiang97 2012-03-17 19:10:23
65+130=195
DPatrick 2012-03-17 19:10:27
DPatrick 2012-03-17 19:10:49
DPatrick 2012-03-17 19:11:06
This problem is mostly a matter of carefully keeping track of our choices as we construct a possible serving.
Iggy Iguana 2012-03-17 19:11:27
there are 9 choices of who gets correct meal
andrewjjiang97 2012-03-17 19:11:27
calculate for one person
KeepingItReal 2012-03-17 19:11:27
9 ways to choose the person with correct order.
mcdonalds106_7 2012-03-17 19:11:27
It is symmetric for the 9 people, so assume one person has his own meal and multiply by 9 at the end
DPatrick 2012-03-17 19:11:33
Right.  We need to pick one person to receive their correct order.  There are 9 choices.
DPatrick 2012-03-17 19:11:50
Without loss of generality, we can suppose it's any person.  We'll count how many ways we can finish the serving, then multiply by 9 (for the 9 choices of which person gets their order) to get our final answer.
DPatrick 2012-03-17 19:12:01
So I made a little chart to help me keep track of things:
DPatrick 2012-03-17 19:12:05
DPatrick 2012-03-17 19:12:16
Notice I've arbitrarily got one beef person getting their correct meal.
DPatrick 2012-03-17 19:12:18
Now what>?
andrewjjiang97 2012-03-17 19:12:33
The other two B's can go under CC, CF, or FF
fireonice 2012-03-17 19:12:33
the other two Bs can get both C, both F, or one C and one F
jubjubdaboss 2012-03-17 19:12:33
there are three cases for the other two B's: F and C, 2 F's, or 2C's
DPatrick 2012-03-17 19:12:44
Right.  The other two Bs can go to 2 of the C people, 2 of the F people, or one each to a C and an F.
FalconPower 2012-03-17 19:12:52
casework
19oshawott98 2012-03-17 19:12:52
case work?
joshxiong 2012-03-17 19:12:52
Casework.
ahaanomegas 2012-03-17 19:12:52
Casework
DPatrick 2012-03-17 19:12:57
Now we have casework. :)
DPatrick 2012-03-17 19:13:06
If they go to 2 C people, how many choices are there?
va2010 2012-03-17 19:13:37
3C2=3
Hydroxide 2012-03-17 19:13:37
3
joshxiong 2012-03-17 19:13:37
3C2=3
alligator112 2012-03-17 19:13:37
3 choices for the 2C, only 1 way for the rest
keelan32 2012-03-17 19:13:37
3 choices for the two C
DPatrick 2012-03-17 19:13:45
There are C(3,2) = 3 choices of two C people.  (Or, think of choosing one of the 3 C people to NOT get beef.)
DPatrick 2012-03-17 19:13:52
Suppose without loss of generality that it's the first two:
DPatrick 2012-03-17 19:13:54
DPatrick 2012-03-17 19:14:02
In how many ways can we finish from here?
lucylai 2012-03-17 19:14:19
the remaining beef people must receive fish
alcra 2012-03-17 19:14:20
Now the others have only 1 choice.
alligator112 2012-03-17 19:14:20
B, B, and C must get F, the 3 F's must get C
DPatrick 2012-03-17 19:14:26
The finish is actually forced: the three fishes must go to the three-remaining non-fish people, and the chickens to the fish people:
DPatrick 2012-03-17 19:14:37
DPatrick 2012-03-17 19:14:48
So there are 3 possibilities in the "2 B's go to 2 C's case".
Iggy Iguana 2012-03-17 19:15:03
this is also the case with both under f
va2010 2012-03-17 19:15:03
the 2 F people is symmetrical
fractals 2012-03-17 19:15:03
2C's and 2F's are the same by symmetry.
mcdonalds106_7 2012-03-17 19:15:03
same for 2Bs go to 2Fs case
DPatrick 2012-03-17 19:15:24
Good -- by symmetry, the "2 B's to 2 F's" case is the same as the previous case.  So there are 3 possibilities in this case too.
esiegel 2012-03-17 19:15:44
but what about... C AND F?
mcdonalds106_7 2012-03-17 19:15:44
now the hard case- one F and one C
NextEinstein 2012-03-17 19:15:44
then 1 to C, 1 to F
DPatrick 2012-03-17 19:15:50
How about the "1 B to each a C and an F"?
DPatrick 2012-03-17 19:16:05
First, how many choices for where the two remaining B's go?
lucylai 2012-03-17 19:16:24
3*3=9
Hydroxide 2012-03-17 19:16:24
3*3
alcra 2012-03-17 19:16:24
Of the C people, one must get a B, and of the F people, one must get a B, so 9 choices here.
aopsmomisawesome 2012-03-17 19:16:24
3x3=9
othuum0149 2012-03-17 19:16:24
3*3=9
DPatrick 2012-03-17 19:16:29
We have 3 choices for which C, and 3 choices for which F, so there are 3*3 = 9 ways to assign the two B's.
DPatrick 2012-03-17 19:16:35
Now we're at this point:
DPatrick 2012-03-17 19:16:38
DPatrick 2012-03-17 19:16:58
DPatrick 2012-03-17 19:17:05
In how many ways can we finish?
19oshawott98 2012-03-17 19:17:51
f and c must go to the remaining Bs
mathswimmer 2012-03-17 19:17:51
C must fill up the Fish, and then 2 choices for which B to go to
alcra 2012-03-17 19:17:51
Two, since the B people need one C one F
DPatrick 2012-03-17 19:17:59
It's clear that the two other C people must receive fish, and the two other F people must receive chicken:
DPatrick 2012-03-17 19:18:03
DPatrick 2012-03-17 19:18:13
So that leaves to decide which B person remaining gets C and which gets F.  That's 2 possibilities.
DPatrick 2012-03-17 19:18:43
So there are 9*2 = 18 possibilities in this case.  (9 choices for the 2 Bs then 2 choices to finish)
lucylai 2012-03-17 19:18:57
total is 9(3+3+18)=216
joshxiong 2012-03-17 19:18:57
9*(3+3+3*3*2)=216
AlcumusGuy 2012-03-17 19:18:57
add them up and multiply by 9
DPatrick 2012-03-17 19:19:02
Adding the cases gives 3+3+18 = 24 ways to finish after we choose the diner who gets their correct order.
DPatrick 2012-03-17 19:19:11
DPatrick 2012-03-17 19:19:29
(remember that the 9 came from the 9 choices of which diner got their order correct.)
DPatrick 2012-03-17 19:20:04
And now what I'm sure was everyone's favorite...
DPatrick 2012-03-17 19:20:07
DPatrick 2012-03-17 19:20:32
Of course, with this problem, the first step is reading it over and over to be sure that you understand what's going on.
DPatrick 2012-03-17 19:20:41
...and then read it again to be SURE you got everything.
DPatrick 2012-03-17 19:20:47
What facts can we learn from the problem statement?
mastermang02 2012-03-17 19:21:21
Horse mile time=10 minutes, Butch mile time=15 minutes, Sundance mile time=24 minutes:bow:
jaw 2012-03-17 19:21:21
When one is walking, the other is riding.
andrewjjiang97 2012-03-17 19:21:21
sparky goes 10 min mile, butch 15, sundance 24
DPatrick 2012-03-17 19:21:26
It takes Butch 15 minutes to walk between mileposts.
DPatrick 2012-03-17 19:21:32
It takes Sundance 24 minutes to walk between mileposts.
DPatrick 2012-03-17 19:21:37
It takes either of them 10 minutes to ride the horse between mileposts.
DPatrick 2012-03-17 19:21:44
Between any two posts, exactly one of them will be walking and one of them will be riding the horse.
DPatrick 2012-03-17 19:22:06
Now you could just work out what happens by brute force, but let's look for a slick solution.
ProblemSolver1026 2012-03-17 19:22:33
Set a system of equations.
mathgiraffe 2012-03-17 19:22:33
let the number of miles Butch rides the horse be a and the number of miles Sundance rides be b.
DPatrick 2012-03-17 19:22:42
I like this idea, but I have better variables. :)
DPatrick 2012-03-17 19:22:49
fractals 2012-03-17 19:23:07
b + s = n
Mary_Posa 2012-03-17 19:23:07
b+s=n
esiegel 2012-03-17 19:23:07
s+b = n
mathgiraffe 2012-03-17 19:23:07
b+s is the total distance
DPatrick 2012-03-17 19:23:18
alligator112 2012-03-17 19:23:32
butch rides s miles, sundance rides b miles
FalconPower 2012-03-17 19:23:32
butch rides for s miles and sundance rides for b
aopsmomisawesome 2012-03-17 19:23:32
butch rides for s miles and sundance rides for b miles
DPatrick 2012-03-17 19:23:50
jubjubdaboss 2012-03-17 19:24:17
we can write equations for the time it takes them
DPatrick 2012-03-17 19:24:40
Exactly.  And we know that the time it takes them to travel the n miles is equal.  So we write expressions for how long it takes each of them to travel the n miles, and set them equal.
alligator112 2012-03-17 19:25:06
15b +10s = 10b + 24s
Iggy Iguana 2012-03-17 19:25:06
15b+10s=24s+10b
superpi83 2012-03-17 19:25:06
Butch: 15b+10s; Sundance: 10b+24s
DPatrick 2012-03-17 19:25:34
DPatrick 2012-03-17 19:25:45
DPatrick 2012-03-17 19:25:50
scgorantla 2012-03-17 19:26:02
therfore 5b=14s
centrino 2012-03-17 19:26:02
5b=14s
cstbear 2012-03-17 19:26:02
so we have 5b=14s
lucylai 2012-03-17 19:26:02
5b=14s
joshxiong 2012-03-17 19:26:02
Thus 5b=14s.
DPatrick 2012-03-17 19:26:11
googol.plex 2012-03-17 19:26:28
b and s are integers and they are as small as possible, so b=14 and s=5
fractals 2012-03-17 19:26:28
b = 14, s = 5 to get the smallest
lucylai 2012-03-17 19:26:28
least possible values are b=14 and s=5
fireonice 2012-03-17 19:26:28
they meet for the first time at 19 miles
centrino 2012-03-17 19:26:28
First time they meet would be when b=14, s=5
DPatrick 2012-03-17 19:26:41
Right.  The smallest solution in positive integers is b=14 and s=5.  (Since 5 and 14 are relatively prime.)
DPatrick 2012-03-17 19:26:49
That is, they travel 19 miles, where Butch walks 14 and Sundance walks 5.
mcdonalds106_7 2012-03-17 19:26:59
now we plug it in
fractals 2012-03-17 19:27:02
So n = 19 and t = 260
DPatrick 2012-03-17 19:27:09
DPatrick 2012-03-17 19:27:22
algebra1337 2012-03-17 19:27:35
but how do we know that they will meet first at 19 miles, and not 38 or something?
DPatrick 2012-03-17 19:27:46
That is an excellent question.  How can we be sure that our solution to the equation is actually possible?  That is, how can we be sure that the horse-swapping occurs correctly so that this 19-mile trek is actually achievable under the travel rules laid out in the problem?
lucylai 2012-03-17 19:28:09
bash!!!
va2010 2012-03-17 19:28:09
Brute Force!
FalconPower 2012-03-17 19:28:09
BASH IT?!?!?
scgorantla 2012-03-17 19:28:09
by plugging it back in
m1sterzer0 2012-03-17 19:28:09
you bash it out anyway to check your answer :p
DPatrick 2012-03-17 19:28:20
We can actually check it rather quickly.  (You might have also solved the problem in the first place using the method that I'm about to describe as our "check".)
DPatrick 2012-03-17 19:28:31
Note that, on any mile, if Sundance rides and Butch walks, Sundance gains 5 minutes on Butch (as it only takes him 10 minutes to ride versus Butch's 15 minutes to walk).
DPatrick 2012-03-17 19:28:45
On the other hand, if Butch rides and Sundance walks, then Butch gains 14 minutes on Sundance.
DPatrick 2012-03-17 19:28:54
And at each milepost, whichever of them is behind (in time) gets to ride.
DPatrick 2012-03-17 19:29:05
So we can quickly check how many minutes Sundance arrives before Butch at each milepost (where a negative number means that Butch arrives before Sundance by that many minutes):
DPatrick 2012-03-17 19:29:08
0, 5, -9, -4, 1, -13, -8, -3, 2, -12, -7, -2, 3, -11, -6, -1, 4, -10, -5, 0.
DPatrick 2012-03-17 19:29:24
At the start and at each negative number, S is behind, so S rides and B walks, and S gains 5 minutes.
At each positive number, S is ahead, so S walks and B rides, and S loses 14 minutes.
DPatrick 2012-03-17 19:29:36
We indeed check and see that after 19 miles, S and B are back even with each other.
proglote 2012-03-17 19:30:05
can we use a graph to solve it, too?
DPatrick 2012-03-17 19:30:10
Certainly.
DPatrick 2012-03-17 19:30:23
DPatrick 2012-03-17 19:30:50
Like a lot of AIME problems, this is actually pretty easy once you decode the problem statement.
DPatrick 2012-03-17 19:30:59
What are we really counting here?
richard4912 2012-03-17 19:31:28
binary numbers that are one apart
deepankar 2012-03-17 19:31:28
pairs of consecutive numbers
geyser 2012-03-17 19:31:28
the groups of numbers that are 1 apart
mcdonalds106_7 2012-03-17 19:31:28
number of consecutive integers that use 5 zeroes and 8 ones
DPatrick 2012-03-17 19:31:33
Right.  We're counting numbers in B to which we can add 1 and still get a number of B.
DPatrick 2012-03-17 19:31:43
When we add 1 to a binary number, when do we not change the number of 0s and 1s (assuming we have the leading zeros like in this problem)?
Mr.117 2012-03-17 19:32:07
when it is 01 to 10
algebra1337 2012-03-17 19:32:07
when the last two digits are 01
kelleyzhao 2012-03-17 19:32:07
If the number ends with 01
Hydroxide 2012-03-17 19:32:07
when the last two digits is 01
AwesomeToad 2012-03-17 19:32:07
____01 -> ______10
thecmd999 2012-03-17 19:32:07
when the last two digits are 01
DPatrick 2012-03-17 19:32:17
Right.  Let's step through this to be sure.
DPatrick 2012-03-17 19:32:23
If the number ends in a 0, then adding 1 just changes the last 0 to a 1, so that doesn't work (we get one additional 1 and one fewer 0).
DPatrick 2012-03-17 19:32:32
If the number ends in 01, we're good!  The 01 becomes 10 in the new number, and nothing else changes.
DPatrick 2012-03-17 19:32:41
If the number ends in more than one 1, what happens?
Relativity1618 2012-03-17 19:33:02
ends in 00
CantonMathGuy 2012-03-17 19:33:02
they all become 0s
scgorantla 2012-03-17 19:33:02
more zeroes appear
infinity1 2012-03-17 19:33:02
at least two ones become one one
himym83 2012-03-17 19:33:02
number of 1s go down
ImAPerson 2012-03-17 19:33:02
they will turn to 0 when 1 is added
DPatrick 2012-03-17 19:33:20
Right.  After we carry the addition, those "more than one 1" all become 0 and the rightmost 0 becomes a 1.
DPatrick 2012-03-17 19:33:34
But that's a net loss of more than one 1s and a gain of just one 0.  So that doesn't work either.
va2010 2012-03-17 19:33:42
so it has to end with 01
DPatrick 2012-03-17 19:33:50
Right.  We've learned that we just need to count the numbers in B that end in 01.
joshxiong 2012-03-17 19:34:07
So we need to count the number of ways to arrange 4 ones and 7 zeroes.
scgorantla 2012-03-17 19:34:07
that makes 7 ones and 4 zeroes left
DPatrick 2012-03-17 19:34:11
The other eleven digits are 4 zeros and 7 ones, in any order.
lucylai 2012-03-17 19:34:24
answer is just 11C4=330
Relativity1618 2012-03-17 19:34:24
11C4=330
Lalagato 2012-03-17 19:34:24
11C4 = 330
negativebplusorminus 2012-03-17 19:34:24
C(11,4)=330
DPatrick 2012-03-17 19:34:46
DPatrick 2012-03-17 19:35:13
On to by far my least favorite question...
DPatrick 2012-03-17 19:35:17
DPatrick 2012-03-17 19:35:31
How do we proceed?
centrino 2012-03-17 19:35:47
Substitue z^13 for w in second equation
himym83 2012-03-17 19:35:47
substitute z in for w
fractals 2012-03-17 19:35:47
z^143 = z by substitution
alligator112 2012-03-17 19:35:47
z^143 = z
jubjubdaboss 2012-03-17 19:35:47
z=(z^13)^11
DPatrick 2012-03-17 19:36:02
va2010 2012-03-17 19:36:16
z^142=1
FalconPower 2012-03-17 19:36:17
z^142=1
esiegel 2012-03-17 19:36:17
z^142 = 1
alcra 2012-03-17 19:36:17
1=z^142
DPatrick 2012-03-17 19:36:23
superpi83 2012-03-17 19:36:56
so z=cis(2k*pi/142)
djmathman 2012-03-17 19:36:56
All roots of unity can be written as cis(pin/71) for some n.  Hence 71 is our answer.
scgorantla 2012-03-17 19:36:56
so 2pi(i)/142
CantonMathGuy 2012-03-17 19:36:56
imaginary part = sin (pi * x / 71)
DPatrick 2012-03-17 19:37:00
DPatrick 2012-03-17 19:37:23
DPatrick 2012-03-17 19:37:31
alcra 2012-03-17 19:37:51
Our final answer is 71.
lucylai 2012-03-17 19:37:52
71 is prime, so it never changes
aopsmomisawesome 2012-03-17 19:37:52
71 is prime
centrino 2012-03-17 19:37:52
71 is prime
scgorantla 2012-03-17 19:37:52
so n is 071 no matter what
mcdonalds106_7 2012-03-17 19:37:52
but 71 is prime and k<71 so the numerator and denominator are relatively prime, so the answer is 071
DPatrick 2012-03-17 19:38:11
Right.  It doesn't matter what k is: since 71 is prime, we cannot reduce the denominator.  (Except to 1, if k=71, but that's not allowed by the problem statement.)
DPatrick 2012-03-17 19:38:16
iwantcombo 2012-03-17 19:38:20
why was this your least favorite question?
Binomial-theorem 2012-03-17 19:38:25
This was one of those you know it or you don't questions.
DPatrick 2012-03-17 19:38:41
Exactly.  I found this problem unpleasing.  If you know roots of unity, it should have been very, very easy.  If you don't, it's pretty much impossible.  What I didn't like is that there's not much "problem solving" -- it's just an exercise with complex numbers and roots of unity.
FalconPower 2012-03-17 19:38:59
like homework almost
DPatrick 2012-03-17 19:39:17
Right, if you've had a trig/complex numbers class (or learned it on your own), this problem is just a homework exercise.
DPatrick 2012-03-17 19:39:25
Fortunately, the next problem is more interesting!
DPatrick 2012-03-17 19:39:31
DPatrick 2012-03-17 19:39:42
DPatrick 2012-03-17 19:39:53
If you stare at the picture a little while, what do you notice?
noedne 2012-03-17 19:40:13
there are three rings of five around the center
DPatrick 2012-03-17 19:40:29
The students appear to be in four "rings".  I've shaded the rings in the picture below:
DPatrick 2012-03-17 19:40:33
DPatrick 2012-03-17 19:41:21
Each student only gives and receive coins from its adjacent rings, and within its own ring (in the case of the outer green ring).
ABCDE 2012-03-17 19:42:32
We can assign variables for each color.
DPatrick 2012-03-17 19:43:12
Sorry, I need to take a short 2-3 minute break...it's pouring rain and our roof is leaking! :(
DPatrick 2012-03-17 19:43:16
Back in 2-3 minutes...
DPatrick 2012-03-17 19:44:12
Sorry about that.  I put a bucket under it.
DPatrick 2012-03-17 19:44:26
Back to our problem...
DPatrick 2012-03-17 19:44:30
DPatrick 2012-03-17 19:44:48
Let's start at the outside and work in.  How are g and b related?
mastermang02 2012-03-17 19:45:16
g=b
theone142857 2012-03-17 19:45:16
g=b
othuum0149 2012-03-17 19:45:16
they're equal
mastermang02 2012-03-17 19:45:16
g=b since they both give 1/4 of their coins to each other:rose:
DPatrick 2012-03-17 19:45:41
I can't really say if all the individual greens and blues are equal (it appears they might be, but we can't prove that).
DPatrick 2012-03-17 19:46:02
But as a group, the green students give half their coins to each other, and half their coins to the students in the blue ring.
DPatrick 2012-03-17 19:46:22
On the other hand, the students in the blue ring also give half their coins to the students in the green ring.
DPatrick 2012-03-17 19:46:31
And for the number of coins for each student to remain equal, we must have
(green -> blue coins) = (blue -> green coins).
DPatrick 2012-03-17 19:46:42
The total number of coins in the green ring equals the total number of coins in the blue ring.  Thus g=b.
DPatrick 2012-03-17 19:46:58
DPatrick 2012-03-17 19:47:14
How are b and r related?
mastermang02 2012-03-17 19:47:34
1/2b=2/3r
theone142857 2012-03-17 19:47:34
4r=3b
aopsmomisawesome 2012-03-17 19:47:34
r = 3b/4
googol.plex 2012-03-17 19:47:34
r=3/4b
Relativity1618 2012-03-17 19:47:34
4r=3b
DPatrick 2012-03-17 19:47:43
The blue ring gives half its coins to the red ring.  The red ring gives 2/3 of its coins to the blue ring.
DPatrick 2012-03-17 19:47:49
DPatrick 2012-03-17 19:48:20
Finally, how are r and w related?
fractals 2012-03-17 19:48:43
1/3r = w
proglote 2012-03-17 19:48:43
1/3 r = w
aussiedoodle 2012-03-17 19:48:43
r/3 = w
DPatrick 2012-03-17 19:49:01
The red ring gives 1/3 of its coins to the person in white in the middle.  This must equal what that person starts with.
DPatrick 2012-03-17 19:49:06
Relativity1618 2012-03-17 19:49:09
substitute and solve for w
DPatrick 2012-03-17 19:49:18
We have r = 3w, and b = (4/3)r = 4w, and g = b = 4w.
DPatrick 2012-03-17 19:49:31
aopsmomisawesome 2012-03-17 19:49:46
Thus, w=280, and the answer is 280
fractals 2012-03-17 19:49:46
12w = 3360, so w = 280
esiegel 2012-03-17 19:49:46
3360/12 good job!
DPatrick 2012-03-17 19:49:51
DPatrick 2012-03-17 19:50:16
Notice I solved the problem without assuming any symmetry (that is, without assuming that all the green circles were equal).
DPatrick 2012-03-17 19:50:42
You could assume symmetry and proceed from there.  It might be a little quicker but it doesn't necessarily cover all the cases.
DPatrick 2012-03-17 19:50:56
However, since the problem implies a unique answer, it's a legitimate strategy.
DPatrick 2012-03-17 19:51:25
DPatrick 2012-03-17 19:51:32
DPatrick 2012-03-17 19:51:48
We have three points where the plane hits edges the cube.  What else should we add to have a complete picture of how the plane intersects the cube?
centrino 2012-03-17 19:52:05
Find where plane intersects FB
Yongyi781 2012-03-17 19:52:06
The first thing you want to find is where the plane intersects FB
yankeefan6795 2012-03-17 19:52:06
point on fb
mcdonalds106_7 2012-03-17 19:52:06
where it intersects FB
NewAlbionAcademy 2012-03-17 19:52:06
Where it intersects with FB
ngahlot 2012-03-17 19:52:06
point on FB
DPatrick 2012-03-17 19:52:13
We should include the other intersection point of the cube's edges and the plane:
DPatrick 2012-03-17 19:52:17
DPatrick 2012-03-17 19:52:23
We know all about M and N.  How will we learn about Q?
Relativity1618 2012-03-17 19:52:57
extend the smaller solid
Relativity1618 2012-03-17 19:52:57
extend NQ
DPatrick 2012-03-17 19:53:11
Right.  It'd be nice to know where DM and NQ intersect.
DPatrick 2012-03-17 19:53:45
One thought is that NQ and BC must intersect somewhere, since they're both in plane BCGF and they're not parallel.  That relates Q to points we know about: N, B, C.
DPatrick 2012-03-17 19:53:55
Another thought is that DM and NQ intersect somewhere, since they're both in plane DMQN, and they're not parallel.  That relates Q to points we know about: D, M, and N.
accents 2012-03-17 19:54:14
Is the intersection on the line BC?
DPatrick 2012-03-17 19:54:34
How do we know this for sure?
fmasroor 2012-03-17 19:54:44
yes since dm is entirely on abcd
timelessmath 2012-03-17 19:54:49
yes because QN and BC are on the same plane
DPatrick 2012-03-17 19:54:57
Right.  Combine these last two comments and we have what we need.
DPatrick 2012-03-17 19:55:13
DM and BC intersect in the plane ABCD.
DPatrick 2012-03-17 19:55:25
NQ and BC intersect in the place BCGF.
DPatrick 2012-03-17 19:55:32
Those planes intersect in the line BC>
DPatrick 2012-03-17 19:56:02
So the plane (DMQN that we're chopping by) intersects the line BC at a point that DM, NQ, and BC all pass through.
DPatrick 2012-03-17 19:56:06
DPatrick 2012-03-17 19:56:29
So using this picture, how can we describe the area inside the cube underneath the plane?
fractals 2012-03-17 19:56:58
A frustum of a pyramid
lucylai 2012-03-17 19:56:58
it's a truncated pyramid
Relativity1618 2012-03-17 19:56:58
frustrum?
sigma96 2012-03-17 19:56:58
a frustrum
DPatrick 2012-03-17 19:57:16
Right!  The big shape PCND is a pyramid with base DCN and vertex P.
DPatrick 2012-03-17 19:57:41
We then chop off the smaller pyramid PMBQ to get the part of the cube under the cutting plane.
DPatrick 2012-03-17 19:57:50
What the size of the smaller pyramid relative to the larger pyramid?
hi how are you doing toda 2012-03-17 19:58:10
1/8
himym83 2012-03-17 19:58:10
1/8
Relativity1618 2012-03-17 19:58:10
1/8
mcdonalds106_7 2012-03-17 19:58:10
1/8
NewAlbionAcademy 2012-03-17 19:58:10
1/8
DPatrick 2012-03-17 19:58:32
Right.  (I was a little too vague when I used the word "size"...I should have said "volume".)
DPatrick 2012-03-17 19:58:52
Since B is the midpoint of PC, the smaller pyramid has half the edge length of the larger one, so has (1/2)^3=1/8 the volume.  Therefore the part of the pyramid that's inside the cube is 7/8 of the large pyramid.
DPatrick 2012-03-17 19:59:12
What is the volume of the large pyramid?
dooba 2012-03-17 19:59:22
Why is B the midpoint of PC?
Hydroxide 2012-03-17 19:59:22
how do you know that B is the midpoint of PC?
DPatrick 2012-03-17 19:59:41
We know that M is the midpoint of AB, so it's also the midpoint of DP.
DPatrick 2012-03-17 20:00:25
And from there we can use similar triangles.  (I'll omit the details for right now.)
ABCDE 2012-03-17 20:00:45
1/4*2*1/3=1/6
scgorantla 2012-03-17 20:00:45
volume of large pyramid is 1/6
Iggy Iguana 2012-03-17 20:00:45
volume of big pyramid = 1/4 * 2 / 3 = 1/6
DPatrick 2012-03-17 20:01:07
The large pyramid has a base with area of triangle DCN, which is 1/4, and its height is PC, which is 2.
DPatrick 2012-03-17 20:01:11
Relativity1618 2012-03-17 20:01:21
volume of frustrum=7/8*1/6=7/48
googol.plex 2012-03-17 20:01:21
the volume of the chunk inside the cube=7/48
DPatrick 2012-03-17 20:01:28
Iggy Iguana 2012-03-17 20:01:47
and thus the big piece is 41/48, giving 089 as answer
himym83 2012-03-17 20:01:47
thus answer is 41/48 or 89
Relativity1618 2012-03-17 20:01:47
subtract frustrum from cube to attain 1-7/48=41/48.41+48=89
theone142857 2012-03-17 20:01:47
volume of bigger portion=1-7/48=41/48
DPatrick 2012-03-17 20:01:52
DPatrick 2012-03-17 20:02:00
DPatrick 2012-03-17 20:02:30
DPatrick 2012-03-17 20:02:58
How can we approach this?
scgorantla 2012-03-17 20:03:35
make each equality equal to a variable, k.
himym83 2012-03-17 20:03:35
set the equalities equal to a variable
scgorantla 2012-03-17 20:03:35
make each equality equal to a variable, k.
vzl11014 2012-03-17 20:03:35
we can set everything as one variable
DPatrick 2012-03-17 20:03:46
eccfcco15 2012-03-17 20:03:59
log -> exponential
fmasroor 2012-03-17 20:03:59
get rid of logs
DPatrick 2012-03-17 20:04:13
DPatrick 2012-03-17 20:04:22
What can we do with these equations?
himym83 2012-03-17 20:04:54
2y*4z=8yz, thus we can use LHS
scgorantla 2012-03-17 20:04:54
multiply the first two equations
fireonice 2012-03-17 20:04:54
multiply the first two
turkeybob777 2012-03-17 20:04:54
multiply the first two together
DPatrick 2012-03-17 20:05:14
We certainly see that the product of the right sides of the first two gives us the right side of the third.
DPatrick 2012-03-17 20:05:24
DPatrick 2012-03-17 20:05:37
DPatrick 2012-03-17 20:05:47
Can we solve for x and/or r using this?
scgorantla 2012-03-17 20:06:12
take the rth root on both sides
aussiedoodle 2012-03-17 20:06:12
take the rth root of both sides
vzl11014 2012-03-17 20:06:12
yes, we can take the r root on both sides
DPatrick 2012-03-17 20:06:21
DPatrick 2012-03-17 20:06:32
Now we can solve for x!
lucylai 2012-03-17 20:06:43
square both sides
mathswimmer 2012-03-17 20:06:43
square both sides 2x^2=4x^8
DPatrick 2012-03-17 20:06:48
DPatrick 2012-03-17 20:06:57
(It's OK to do this since everything in sight is positive.)
himym83 2012-03-17 20:07:14
x = 2^-1/6
cstar124 2012-03-17 20:07:17
x^6=1/2
DPatrick 2012-03-17 20:07:21
DPatrick 2012-03-17 20:07:46
Great, we found x!  We're 1/3 of the way there (hopefully).  Now what?
DPatrick 2012-03-17 20:07:56
Recall our system:
DPatrick 2012-03-17 20:08:03
FalconPower 2012-03-17 20:08:15
plug back in?
scgorantla 2012-03-17 20:08:15
plug in x
lucylai 2012-03-17 20:08:15
plug x back in
DPatrick 2012-03-17 20:08:34
I could plug x back in, but let's leave it as "x" for a moment.  2^(-1/6) seems messy to use until we really need it.
DPatrick 2012-03-17 20:09:01
Also note that we've used the information in the 3rd equation already, so it's not going to help us further.
DPatrick 2012-03-17 20:09:14
fractals 2012-03-17 20:09:39
Multiply: (first equation)^5*(second equation)
fireonice 2012-03-17 20:09:39
we need y^5z so let's find that in terms of the equations
theone142857 2012-03-17 20:09:39
take the first equation to the 5th and multiply the second and x on both sides
DPatrick 2012-03-17 20:09:46
Aha!  Let's keep our eye on the ball!
DPatrick 2012-03-17 20:09:56
We want the quantity y^5z as part of our final answer, so we can use the first two equations to get it on the right side.
DPatrick 2012-03-17 20:10:03
Specifically, let's raise the first equation to the 5th power and multiply by the second.
DPatrick 2012-03-17 20:10:13
DPatrick 2012-03-17 20:10:27
What does the left side simplify to?
FalconPower 2012-03-17 20:10:57
2^(r/2)*x^3r
DPatrick 2012-03-17 20:11:05
calculus17 2012-03-17 20:11:19
plug in for x
DPatrick 2012-03-17 20:11:31
Now seems like a good time to plug in the x -- we've simplified as far as we can.
DPatrick 2012-03-17 20:11:40
lucylai 2012-03-17 20:11:56
it cancels it out
himym83 2012-03-17 20:11:56
r's cancel when x is plugged back in
fireonice 2012-03-17 20:11:56
the left side is 1!
calculus17 2012-03-17 20:11:56
1=2^7y^5z
DPatrick 2012-03-17 20:12:00
Something wonderful happened!
DPatrick 2012-03-17 20:12:07
fractals 2012-03-17 20:12:19
y^5z=2^-7
lucylai 2012-03-17 20:12:19
y^5*z=2^-7
DPatrick 2012-03-17 20:12:23
theone142857 2012-03-17 20:12:40
mutiply x on both sides
coldsummer 2012-03-17 20:12:40
multiply by x
accents 2012-03-17 20:12:44
But don't forget, we're looking for xy^5z, and we have both!@@
DPatrick 2012-03-17 20:12:53
DPatrick 2012-03-17 20:13:29
DPatrick 2012-03-17 20:13:54
Again, a lot of words and notation.
DPatrick 2012-03-17 20:14:03
How can we describe the numbers in S?
calculus17 2012-03-17 20:14:23
all squares ending in 256
vwu9 2012-03-17 20:14:23
squares that end with 256
AlcumusGuy 2012-03-17 20:14:23
perfect squares ending in 256
fireonice 2012-03-17 20:14:23
perfect squares that end with 256
DPatrick 2012-03-17 20:14:38
How can we algebraically describe a number ending in 256?
Relativity1618 2012-03-17 20:14:56
1000a+256
somanywindows 2012-03-17 20:14:56
1000x + 256
fractals 2012-03-17 20:14:56
1000n+256
kealand 2012-03-17 20:14:56
1000N + 256
DPatrick 2012-03-17 20:15:00
DPatrick 2012-03-17 20:15:10
DPatrick 2012-03-17 20:15:17
Relativity1618 2012-03-17 20:15:43
so we do difference of squares
CantonMathGuy 2012-03-17 20:15:43
(k + 16)(k - 16) = 1000n
Iggy Iguana 2012-03-17 20:15:43
subtract 256 and factor
professordad 2012-03-17 20:15:43
difference of squares?
fmasroor 2012-03-17 20:15:43
256=16^2, so we have 1000n=(k-16)(k+16)
DPatrick 2012-03-17 20:15:47
Good -- we can write as the difference of two perfect squares and factor.
DPatrick 2012-03-17 20:15:53
DPatrick 2012-03-17 20:16:08
How does this help?
professordad 2012-03-17 20:16:32
both k-16 and k+16 cannot be divisible by 125, so 125 divides either k-16 or k+16
fireonice 2012-03-17 20:16:32
only one of the terms can contribute 125
gomath888 2012-03-17 20:16:32
now just look where the 2s and 5s go
DPatrick 2012-03-17 20:16:45
Indeed, the right side must be a multiple of 1000.
DPatrick 2012-03-17 20:16:53
Only one of the factors can be a multiple of 5 (since the factors are 32 apart).  Therefore one of the factors is a multiple of 125.
GeorgiaTechMan 2012-03-17 20:17:04
also, both k+16 and k-16 must be divisible by 4
DPatrick 2012-03-17 20:17:33
Right.  They clearly must both be even, but if one is not a multiple of 4, neither is the other (since they're 32 apart), and we can't then have the right side be a multiple of 8.
somanywindows 2012-03-17 20:17:45
(k + 16) or (k - 16) has to be a multiple of 500
himym83 2012-03-17 20:17:45
thus must be divisible by 500
DPatrick 2012-03-17 20:18:03
Right.  One of the factors on the right side must be a multiple of 500.
DPatrick 2012-03-17 20:18:08
Relativity1618 2012-03-17 20:18:35
so now we list values for which either is true
Iggy Iguana 2012-03-17 20:18:35
16, 500-16, 500 + 16, ...2500-16 is 10th
lucylai 2012-03-17 20:18:35
form a list of values for k
DPatrick 2012-03-17 20:18:44
Right.  We can just make a list.  The first 10 k's are 16, 500-16, 500+16, 1000-16, ..., up to the tenth one being 2500-16.
DPatrick 2012-03-17 20:18:52
DPatrick 2012-03-17 20:18:59
Don't simplify 2500-16, because it's easier to square as it is!
DPatrick 2012-03-17 20:19:10
DPatrick 2012-03-17 20:19:12
Now what?
AlcumusGuy 2012-03-17 20:19:36
look back at T
himym83 2012-03-17 20:19:37
get rid of 256
CantonMathGuy 2012-03-17 20:19:37
make it mod 1000
fireonice 2012-03-17 20:19:37
drop the 256 and divide 1000
scgorantla 2012-03-17 20:19:37
subtract 256 and divide by 1000
DPatrick 2012-03-17 20:19:45
Right, we chop off the 256, and divide by 1000.
DPatrick 2012-03-17 20:19:52
GeorgiaTechMan 2012-03-17 20:20:06
so our answer is 170!
AkshajK 2012-03-17 20:20:06
Therefore, its 170
himym83 2012-03-17 20:20:06
remainder is 170
DPatrick 2012-03-17 20:20:10
DPatrick 2012-03-17 20:20:45
I'm going to take a short break to rest my typing fingers before we tackle the final five.  We'll resume at :25 past the hour (about 4 minutes from now).  Go stretch your legs!
DPatrick 2012-03-17 20:25:36
OK, we're back.  The ceiling is still leaking here but rrusczyk is here to put more buckets underneath! :)
DPatrick 2012-03-17 20:25:43
DPatrick 2012-03-17 20:26:09
(For what it's worth I thought this was the hardest problem.)
DPatrick 2012-03-17 20:26:40
DPatrick 2012-03-17 20:27:01
You can't make much headway unless you make a couple of key observations.
Iggy Iguana 2012-03-17 20:27:26
note that x+y is always divisible by 3 and x-y is always divisible by 5, so now all we have to do is prove all of these points can be hopped to
gomath888 2012-03-17 20:27:26
sum invariant mod 3, difference invariant mod 5
GeorgiaTechMan 2012-03-17 20:27:26
sum divisible by 3, difference divisible by 5
DPatrick 2012-03-17 20:27:41
Right.  These are the key observations.
DPatrick 2012-03-17 20:27:50
All of the coordinates add to a multiple of three.  Therefore no matter where we travel, we will always be on a point with coordinates that add to a multiple of 3.
DPatrick 2012-03-17 20:28:08
And, the differences in the coordinates are always a multiple of five.  Therefore no matter where we travel, we will always be on a point with coordinates that have difference equal to a multiple of 5.
DPatrick 2012-03-17 20:28:27
DPatrick 2012-03-17 20:28:39
DPatrick 2012-03-17 20:29:20
The question is, before we start counting: can we actually reach all such points?
scgorantla 2012-03-17 20:29:55
yes
somanywindows 2012-03-17 20:29:55
yes
scgorantla 2012-03-17 20:29:55
yes, we can
GeorgiaTechMan 2012-03-17 20:29:55
yes
DPatrick 2012-03-17 20:30:23
How do we know?
DPatrick 2012-03-17 20:31:09
Remember, we can only add these vectors above.  We can’t subtract them.  Therefore that might restrict the places we can go.  (For example, even if we could move left some amount that doesn’t show us that we can move right that same amount.)
Iggy Iguana 2012-03-17 20:32:05
you can make (0, 15)
CantonMathGuy 2012-03-17 20:32:06
5A + 5B + 9C + 9D = 0
DPatrick 2012-03-17 20:32:42
Indeed, we can track the points that we can move to horizontally and vertically, and we can also see that we can get back to 0.
DPatrick 2012-03-17 20:33:39
There are a number of ways to show that you can reach every possible point.  (It might also be reasonable, on the contest, to assume you can reach every possible point, and proceed with the counting.)
DPatrick 2012-03-17 20:34:42
What I did is to show that you can move from a point (x,y) on x-y = 5l to a point (x,y) on either x-y = 5(l-1) or 5(l+1) by using either A or B.  So we can hope between the lines of slope 1.
DPatrick 2012-03-17 20:35:46
We can then show that we can move along the lines of slope 1.  For example, A+B = (9,9) and C+D = (-15,-15).  So 2(A+B) + (C+D) = (3,3) and 3(A+B) + 2(C+D) = (-3,-3), and then we can hop up and down the line at will.
DPatrick 2012-03-17 20:36:07
(And there are many other ways to show this too.  It's rather tedious and boring.)
DPatrick 2012-03-17 20:36:35
So we can reach every lattice point (x,y) with x+y a multiple of 3 and x-y a multiple of 5.
DPatrick 2012-03-17 20:36:42
Now we are set to count.  We want all of these points:
DPatrick 2012-03-17 20:36:46
DPatrick 2012-03-17 20:36:49
Start counting.  I’ll wait. . . .
Relativity1618 2012-03-17 20:37:06
there must be a better way!
DPatrick 2012-03-17 20:37:14
This is a square of dots, but it’s troublesome because the boundaries are at strange slopes and it’ll be very easy get off-by-one errors when counting each of these rows.
DPatrick 2012-03-17 20:37:27
Any better ideas?
fmasroor 2012-03-17 20:37:36
rotate it
DPatrick 2012-03-17 20:37:40
:)
DPatrick 2012-03-17 20:37:55
Technically what "rotate it" means is to use new coordinates.
DPatrick 2012-03-17 20:38:02
DPatrick 2012-03-17 20:38:23
This also makes the lattice conditions nicer: we need u to be a multiple of 3 and v to be a multiple of 5.
DPatrick 2012-03-17 20:38:31
Relativity1618 2012-03-17 20:38:45
a square
AkshajK 2012-03-17 20:38:45
a square
lucylai 2012-03-17 20:38:45
squares!!!
RelaxationUtopia 2012-03-17 20:38:45
square
DPatrick 2012-03-17 20:38:53
DPatrick 2012-03-17 20:39:06
But there's one more condition in (u,v) land.
Iggy Iguana 2012-03-17 20:39:24
they must have same parity
baldcypress 2012-03-17 20:39:29
u and v have the same parity
DPatrick 2012-03-17 20:39:46
Right.  Since our original x,y points were integers, we must have u and v be the same parity.
DPatrick 2012-03-17 20:39:56
They're either both even or both odd.
DPatrick 2012-03-17 20:40:08
So we want to count solutions to:
DPatrick 2012-03-17 20:40:11
DPatrick 2012-03-17 20:40:34
Now for the counting.
hrithikguy 2012-03-17 20:40:43
clearly casework on whether they are both even or both odd
GeorgiaTechMan 2012-03-17 20:40:43
we can do two cases; both are even or both are d=odd.
DPatrick 2012-03-17 20:40:54
Sounds good.
DPatrick 2012-03-17 20:41:05
If u is an even multiple of 3, then u is a multiple of 6.  The multiples of 6 between -100 and 100 are -96,-90,...,90,96, and there are 33 of those.
DPatrick 2012-03-17 20:41:17
How many v's work?
mcdonalds106_7 2012-03-17 20:41:41
21 vs
aussiedoodle 2012-03-17 20:41:41
21
DPatrick 2012-03-17 20:41:45
If v is an even multiple of 5, then v is a multiple of 10.  There are 21 multiples of 10 between -100 and 100 (inclusive).
DPatrick 2012-03-17 20:42:00
So there are 33*21 (u,v)'s in the u,v even case.
DPatrick 2012-03-17 20:42:10
How many odd (u,v)'s?
mcdonalds106_7 2012-03-17 20:42:30
34*20
Bryan.C 2012-03-17 20:42:30
34*20
ProbaBillity 2012-03-17 20:42:30
34*20
DPatrick 2012-03-17 20:42:42
The odd multiples of 3 in the range are -99,-93,...,93,99.  There are 34 of those.
DPatrick 2012-03-17 20:42:54
The odd multiples of 5 in this range occur between the even values, so there is one fewer.  There are 20 odd multiples of 5 in this region.
DPatrick 2012-03-17 20:43:00
(-95, -85, ..., 85, 95)
DPatrick 2012-03-17 20:43:11
So there are 34*20 (u,v)'s in the u,v odd case.
professordad 2012-03-17 20:43:26
33*21 + 34*20 = 1373, so 373
Iggy Iguana 2012-03-17 20:43:26
so we have 680+693=1373, for an answer of 373
fractals 2012-03-17 20:43:26
So 1373 total, so the answer is 373.
DPatrick 2012-03-17 20:43:29
DPatrick 2012-03-17 20:43:35
DPatrick 2012-03-17 20:44:17
What's slightly bad about this problem from the point-of-view of a contest is that you don't have to prove that all the points are reachable -- you could assume it (and be right).  And proving it is tedious.
DPatrick 2012-03-17 20:44:35
DPatrick 2012-03-17 20:44:50
lucylai 2012-03-17 20:45:01
30 degree angles
RelaxationUtopia 2012-03-17 20:45:01
30 degrees
ABCDE 2012-03-17 20:45:01
30 degree angles
Iggy Iguana 2012-03-17 20:45:01
3 30 degree angles
DPatrick 2012-03-17 20:45:04
We have 30 degree angles.  We're also given DE/BE = 8/15, so we'll add that to the diagram, too:
DPatrick 2012-03-17 20:45:07
thecmd999 2012-03-17 20:45:21
angle bisector theorem!
RelaxationUtopia 2012-03-17 20:45:21
diagram ---> angle bisector
Typhoon131 2012-03-17 20:45:21
Angle Bisector Theorem
DPatrick 2012-03-17 20:45:27
We have a ratio of sides and we have angle bisectors; we break out the Angle Bisector Theorem, which tells us that CD/CB = DE/BE.  So, we have CD/CB = 8/15, and we add that to the diagram:
DPatrick 2012-03-17 20:45:34
DPatrick 2012-03-17 20:45:59
Now, there are lots of ways to proceed via trig bashing.  But there is a very quick, simple solution that just uses 30-60-90 triangles!
scgorantla 2012-03-17 20:46:12
draw the height from D to BC
Typhoon131 2012-03-17 20:46:12
Drop perpendicular from D to BC
ABCDE 2012-03-17 20:46:12
Drop altitude from D to BC.
DPatrick 2012-03-17 20:46:21
We build a 30-60-90 triangle involving a side we have information about by dropping an altitude from D:
DPatrick 2012-03-17 20:46:25
DPatrick 2012-03-17 20:46:37
Why did I bother doing that?
Iggy Iguana 2012-03-17 20:46:47
we have YC = 4t and DY = 4sqrt3 t
DPatrick 2012-03-17 20:46:54
Typhoon131 2012-03-17 20:47:12
CY = 4t, BY = 11t
Relativity1618 2012-03-17 20:47:12
BY=11T
Iggy Iguana 2012-03-17 20:47:21
so BY = 11t
scgorantla 2012-03-17 20:47:21
BY=11t
mathswimmer 2012-03-17 20:47:27
DY/YB
krmathcounts 2012-03-17 20:47:27
tan B = DY/YB
DPatrick 2012-03-17 20:47:30
And now we're done!
DPatrick 2012-03-17 20:47:41
BY = BC - YC = 15t - 4t = 11t.
DPatrick 2012-03-17 20:48:03
And because we have our eye on the ball, we can immediately finish by computing tan B.
DPatrick 2012-03-17 20:48:16
DPatrick 2012-03-17 20:48:25
mcdonalds106_7 2012-03-17 20:48:33
way easier than #11 wow
DPatrick 2012-03-17 20:48:40
I agree. Don't make problems harder than they are!
DPatrick 2012-03-17 20:48:58
ahaanomegas 2012-03-17 20:49:12
Diagram!
Relativity1618 2012-03-17 20:49:12
draw a diagram
DPatrick 2012-03-17 20:49:16
In a construction problem, it is often best to start with the final diagram, and look for properties that will help us construct the diagram.
DPatrick 2012-03-17 20:49:20
DPatrick 2012-03-17 20:49:56
What is a key fact that we need to determine?
mcdonalds106_7 2012-03-17 20:50:14
draw center to vertices
theone142857 2012-03-17 20:50:14
Draw the center  and connect it to P,Q,R
fractals 2012-03-17 20:50:14
Where the center is.
DPatrick 2012-03-17 20:50:41
Right.  It looks like the center is inside the triangle.  If you do an accurate enough sketch you'll convince yourself.
RelaxationUtopia 2012-03-17 20:50:48
Wait how do I know what triangle would be the biggest?
tc1729 2012-03-17 20:51:10
is there only one such equilateral triangle that can be constructed?
Iggy Iguana 2012-03-17 20:51:13
there's another really small triangle such that the center is outside triangle
DPatrick 2012-03-17 20:51:28
These are good questions -- how do we know that this is essentially the only triangle that we want?
DPatrick 2012-03-17 20:51:54
We can let R be any point on circle C_5.  We then want to find the appropriate points P and Q.
DPatrick 2012-03-17 20:52:08
What do we know about equilateral triangle PQR that may help us construct it?
Relativity1618 2012-03-17 20:52:22
60 degrees
mathswimmer 2012-03-17 20:52:22
60 degrees
Iggy Iguana 2012-03-17 20:52:22
equal sides
scgorantla 2012-03-17 20:52:22
all angles are 60
DPatrick 2012-03-17 20:52:42
In particular we know that <PRQ = 60.  How can we find points P on circle C_3 and Q on circle C_4 so that <PRQ = 60?
scgorantla 2012-03-17 20:52:57
rotation?
DPatrick 2012-03-17 20:53:03
We can rotate circle C_3 around point R by 60 degrees, to obtain circle C_3'.
DPatrick 2012-03-17 20:53:05
DPatrick 2012-03-17 20:53:32
If P is on C_3, then P rotates around to Q, so Q must be on C_3'.
ProbaBillity 2012-03-17 20:53:36
the intersection of C3' and C4 is Q
DPatrick 2012-03-17 20:53:54
But Q is also on C_4.  So there are only two possible locations for Q, and thus only 2 possible triangles to consider.
ProbaBillity 2012-03-17 20:53:58
the tiny equilateral triangle comes from the other intersection point
DPatrick 2012-03-17 20:54:04
DPatrick 2012-03-17 20:54:22
Right, there's a tiny triangle coming from the other intersection point, but it is clearly way way smaller.
DPatrick 2012-03-17 20:54:33
So the triangle we started with in our diagram is definitely the one we want.
DPatrick 2012-03-17 20:54:49
And if you brought a ruler and compass, you could actually try to draw a pretty accurate picture.
DPatrick 2012-03-17 20:55:03
And back to an earlier question: note that for this solution, the center of the circles O lies inside equilateral triangle PQR.
DPatrick 2012-03-17 20:55:08
DPatrick 2012-03-17 20:55:15
We know that OP = 3, OQ = 4, and OR = 5.  Do these lengths suggest anything to you?
scgorantla 2012-03-17 20:55:25
I see a right triangle!
Relativity1618 2012-03-17 20:55:25
pythag
himym83 2012-03-17 20:55:25
right triangles
Iggy Iguana 2012-03-17 20:55:25
right trianlges!
ProbaBillity 2012-03-17 20:55:25
look for right triangles
henrypickle 2012-03-17 20:55:25
3-4-5 right triangle
DPatrick 2012-03-17 20:55:36
The lengths 3, 4, and 5 form the sides of a right triangle.  So it would be ideal if we can construct a triangle with these lengths in the diagram.  Is there any way to do that?
dandan 2012-03-17 20:55:56
rotate it
himym83 2012-03-17 20:55:57
rotation
mathswimmer 2012-03-17 20:55:57
some transformation?
mcdonalds106_7 2012-03-17 20:55:57
rotate the triangle a bunch of times
prezcoin 2012-03-17 20:55:57
rotation by 60deg to make that triangle appear
DPatrick 2012-03-17 20:56:32
There are a lot of things you could do -- one good idea is to rotate the picture 60 degrees clockwise, centered at point P:
DPatrick 2012-03-17 20:56:36
DPatrick 2012-03-17 20:56:59
So R gets rotated over to Q, and O gets rotated to a new point O'.  (I've removed the circles for a little clarity)
Iggy Iguana 2012-03-17 20:57:12
OO'P is equalatera
DPatrick 2012-03-17 20:57:36
Right: OP = O'P because of the rotation, and also angle OPO' = 60 degrees because of the rotation.  So OPO' is equilateral.
mcdonalds106_7 2012-03-17 20:57:56
we see that OO'Q is 3-4-5 as well
letsruletheworldtogether 2012-03-17 20:57:56
QO' is 5
DPatrick 2012-03-17 20:58:11
Right!  RO rotates to QO', so the length RO is preserved, and QO' = 5.
DPatrick 2012-03-17 20:58:16
DPatrick 2012-03-17 20:58:26
This means that OO'Q is our 3-4-5 right triangle.  How does that help us?
baldcypress 2012-03-17 20:58:40
so < qop is 150
scgorantla 2012-03-17 20:58:41
Now we can just apply the law of cosines
DPatrick 2012-03-17 20:59:11
Right.  We know angle QOO' is 90 (it's the right angle in our 3-4-5 triangle), and O'OP is 60 degrees, so QOP is a 150-degree angle.
DPatrick 2012-03-17 20:59:37
So we can use the Law of Cosines on the triangle QOP to compute the length QP, which is the side of the triangle PQR that we care about in the problem!
FalconPower 2012-03-17 21:00:02
Qp^2=4^2+3^2-2*4*3*cos150
tc1729 2012-03-17 21:00:06
QP^2 = 3^2+4^2-2(12)cos 150
DPatrick 2012-03-17 21:00:10
DPatrick 2012-03-17 21:00:27
superpi83 2012-03-17 21:00:49
Then use area=s^2*sqrt(3)/4
Relativity1618 2012-03-17 21:00:49
now we can find the area
Iggy Iguana 2012-03-17 21:00:49
so now just multiply by sqrt3 / 4 to get area
fractals 2012-03-17 21:00:49
And the area is PQ^2sqrt3/4
letsruletheworldtogether 2012-03-17 21:00:49
times sqrt(3)/4
theone142857 2012-03-17 21:00:49
multiply by sqrt{3}/4
himym83 2012-03-17 21:00:49
multiply by sqrt3/4 to get ans
DPatrick 2012-03-17 21:00:57
theone142857 2012-03-17 21:01:13
So since 25+9+4+3=41. So 041 is our answer.
Relativity1618 2012-03-17 21:01:14
9+25+4+3=41
DPatrick 2012-03-17 21:01:15
fractals 2012-03-17 21:01:26
Reflecting O over PQ, QR, and RP and making a hexagon, and drawing O'O''O''' works as well.
DPatrick 2012-03-17 21:01:47
Certainly there are other transformations that work -- the key is doing something that produces a 3-4-5 triangle, and thus a right angle.
DPatrick 2012-03-17 21:01:59
Also, this problem can be solved very carefully by using coordinates -- the trick is to fix the coordinates of the the points (using the fact that the triangle is equilateral), and solve for the center.
DPatrick 2012-03-17 21:02:24
DPatrick 2012-03-17 21:02:39
What information do we get from the cubic?
thecmd999 2012-03-17 21:02:53
vieta! a+b+c=0
fireonice 2012-03-17 21:02:53
a+b+c=0 from Vieta
lucylai 2012-03-17 21:02:53
a+b+c=0
Yongyi781 2012-03-17 21:02:53
a+b+c = 0
scgorantla 2012-03-17 21:02:53
sum of roots is 0
professordad 2012-03-17 21:02:53
the sum of a,b,c is 0
DPatrick 2012-03-17 21:03:16
DPatrick 2012-03-17 21:03:23
We can throw the cubic away now -- it can't possibly tell us anything else.
DPatrick 2012-03-17 21:04:05
Now this problem had the unfortunate feature that you could make simplifying assumptions about the roots, and everything would work out nicely.  But I want to show how to solve the problem without making any such assumptions.
Relativity1618 2012-03-17 21:04:14
plot the roots?
DPatrick 2012-03-17 21:04:22
Let's suppose (without loss of generality) that c is the right angle, and that a rotates counterclockwise about c to get to b, like so:
DPatrick 2012-03-17 21:04:27
DPatrick 2012-03-17 21:04:54
What is rotation by 90 degrees in the complex plane equivalent to?
Relativity1618 2012-03-17 21:05:11
multiplying by i
osmosis92 2012-03-17 21:05:11
times i
gomath888 2012-03-17 21:05:11
multiplication by i
djmathman 2012-03-17 21:05:11
Multiplying by i
mathswimmer 2012-03-17 21:05:11
multiply by i
superpi83 2012-03-17 21:05:11
multiplication by i
DPatrick 2012-03-17 21:05:20
It's multiplication by i.  And possibly scaling by a positive real number.
DPatrick 2012-03-17 21:05:38
DPatrick 2012-03-17 21:06:01
Now what?
coldsummer 2012-03-17 21:06:21
simplifying and substitution?
DPatrick 2012-03-17 21:06:34
DPatrick 2012-03-17 21:06:50
DPatrick 2012-03-17 21:07:01
How does this help.  How do we "solve" this?
scgorantla 2012-03-17 21:07:20
ratio of a to b
calculus17 2012-03-17 21:07:24
A IN TERMS OF B
DPatrick 2012-03-17 21:07:31
Right, it fixes the ratio of a to b.
DPatrick 2012-03-17 21:07:40
haihongjin 2012-03-17 21:07:46
r was used in the proplem
DPatrick 2012-03-17 21:08:03
oops...good point.  The r I'm using here is not the same r that's in the cubic in the problem.  My bad.
fmasroor 2012-03-17 21:08:15
|a|^2+...=250
capu 2012-03-17 21:08:15
well remember that the squares add up to 250
DPatrick 2012-03-17 21:08:30
Now we use the remaining bit of data: the sum of the squares of the magnitudes is 250.
DPatrick 2012-03-17 21:08:48
DPatrick 2012-03-17 21:09:16
Well, that may look messy, but keep your eye on the ball!  What are we trying to find?
Iggy Iguana 2012-03-17 21:09:27
hypotenuse
Relativity1618 2012-03-17 21:09:27
h^2
proglote 2012-03-17 21:09:35
(a-b)^2
somanywindows 2012-03-17 21:09:39
distance from a to b
DPatrick 2012-03-17 21:09:46
djmathman 2012-03-17 21:10:03
Multiply by 3/2!
DPatrick 2012-03-17 21:10:06
This is exactly 3/2 times the quantity of 250 from our previous calculation!
CantonMathGuy 2012-03-17 21:10:21
375
ProbaBillity 2012-03-17 21:10:22
375
FalconPower 2012-03-17 21:10:22
375!!!
DPatrick 2012-03-17 21:10:24
DPatrick 2012-03-17 21:10:47
There are some shortcuts to this problem.  You could assume that the triangle is isosceles (that is, that r=1 in the above computation) and it'd still work.  But my computation actually proves that it works for all a,b,c satisfying the conditions of the problem.
DPatrick 2012-03-17 21:11:27
My colleague Jeremy Copeland pointed out that he assumed the triangle was degenerate, that is that b=c, and it still works with much simpler computation.
DPatrick 2012-03-17 21:11:39
And last but not least,
DPatrick 2012-03-17 21:11:43
DPatrick 2012-03-17 21:12:04
Yikes.  How can we begin to break this down?
Relativity1618 2012-03-17 21:12:16
mods?
fractals 2012-03-17 21:12:16
Modular Arithmetic!
DPatrick 2012-03-17 21:12:52
We're almost certainly going to want to work mod n, since we only care about how far about things are around the circle, which means looking at the differences mod n.
DPatrick 2012-03-17 21:13:01
What does condition (1) tell us?
scgorantla 2012-03-17 21:13:27
multiply the seat you were in by a
fractals 2012-03-17 21:13:27
a is relatively prime to n
DPatrick 2012-03-17 21:13:52
Right.  The reseating operation is taking everyone's seat and multiplying it by a, then taking mod n to figure out where they sit.
DPatrick 2012-03-17 21:14:07
But everybody has to have their own seat after this is all done!
DPatrick 2012-03-17 21:14:17
DPatrick 2012-03-17 21:14:36
(Otherwise two of them will end up in the same seat after multiplying by a.)
turak 2012-03-17 21:14:51
a is not a zero divisor mod n
ahaanomegas 2012-03-17 21:14:52
(a, n) = 1.
scgorantla 2012-03-17 21:14:52
so a is relatively prime to n!
DPatrick 2012-03-17 21:14:56
DPatrick 2012-03-17 21:15:12
DPatrick 2012-03-17 21:15:23
DPatrick 2012-03-17 21:15:35
(For example, if n = 14 and a = 10, then j-k = 7 fails, since (j-k)a = 10*7 = 70 is a multiple of 14.)
DPatrick 2012-03-17 21:15:43
Relativity1618 2012-03-17 21:15:51
introduce statement 2
DPatrick 2012-03-17 21:15:55
What about condition (2)?  What can we say about it?
karatemagic7 2012-03-17 21:16:38
gcd(a-1,n)=1 and gcd(a+1,n)=1
turak 2012-03-17 21:16:38
neither a-1 nor a+1 are zero divisors mod n
DPatrick 2012-03-17 21:16:56
That's true, but that skips a couple of steps we need to get there.  Let me fill in the missing steps.
DPatrick 2012-03-17 21:17:04
DPatrick 2012-03-17 21:17:27
(That is, the difference between seats ak and aj is not the same as the difference from k to j in one direction)
DPatrick 2012-03-17 21:17:33
DPatrick 2012-03-17 21:18:13
Iggy Iguana 2012-03-17 21:18:31
by same logic as before
DPatrick 2012-03-17 21:18:50
DPatrick 2012-03-17 21:19:06
DPatrick 2012-03-17 21:19:20
What does this imply about n?
Iggy Iguana 2012-03-17 21:19:36
so that means that 2 or 3 does not divide n because one of a-1, a, a+1 is divisible by 2, and one is divisible by 3
baldcypress 2012-03-17 21:19:36
well then a can't have a factor of 2 or 3
mcdonalds106_7 2012-03-17 21:19:36
so n cannot have a factor of 2 or 3
guilt 2012-03-17 21:19:36
not divisible by 2, 3
DPatrick 2012-03-17 21:19:56
Right.  We can't have n even, since one of a-1, a, and a+1 must be even, and thus would have the factor of 2 in common with n.
DPatrick 2012-03-17 21:20:30
In a similar way, one of a-1, a, and a+1 must be a multiple of 3, and thus n can't be a multiple of 3 (because it'd have a factor of 3 in common with one of a-1, a, or a+1).
DPatrick 2012-03-17 21:20:40
So n cannot be a multiple of 2 or 3.  Any other conditions?
scgorantla 2012-03-17 21:20:58
nope, thats it
ProbaBillity 2012-03-17 21:20:58
no
superpi83 2012-03-17 21:21:01
nope, no other conditions if a=2
DPatrick 2012-03-17 21:21:19
That's actually sufficient too.  If n>1 is not a multiple of 2 or 3, then we can pick a = 2 and everything works, since neither 1, 2, nor 3 would have a common factor with n.
DPatrick 2012-03-17 21:21:31
So to finish the problem, we need to count all the n with 1 < n < 1000 such that n is not a multiple of 2 or 3.
Iggy Iguana 2012-03-17 21:21:41
n has to be 1 or 5 mod 6
DPatrick 2012-03-17 21:21:49
Yes: an easy way is to recognize that this means that n must be 1 or 5 (mod 6).
DPatrick 2012-03-17 21:21:54
So for every 6k (that is, every multiple of 6) between 1 and 1000, the numbers 6k-1 and 6k+1 will both work.
ProbaBillity 2012-03-17 21:22:12
166*2 = 332
mcdonalds106_7 2012-03-17 21:22:14
so our answer is 166*2=332
DPatrick 2012-03-17 21:22:20
The smallest multiple is 6 and the largest is 996 = 6*166, so there are 166 valid multiples.
DPatrick 2012-03-17 21:22:25
DPatrick 2012-03-17 21:23:05
(What's potentially sad about #15, of course, is that you could do all the hard number theory work, and then mess up the counting at the end.  That's why it's important to be careful with the endgame to an AIME problem!)
DPatrick 2012-03-17 21:23:15
That's it!  That's for hanging out with me on a Saturday night.
DPatrick 2012-03-17 21:23:22
And please join us again for the AIME II Math Jam on Friday, March 30, when we'll do all this again for the AIME II.

Copyright © 2024 AoPS Incorporated. This page is copyrighted material. You can view and print this page for your own use, but you cannot share the contents of this file with others.