Difference between revisions of "2024 AMC 12B Problems/Problem 13"
(→Solution 2 (Coordinate Geometry and HM-GM)) |
(→Solution 3) |
||
| Line 46: | Line 46: | ||
~[https://artofproblemsolving.com/wiki/index.php/User:Cyantist luckuso] | ~[https://artofproblemsolving.com/wiki/index.php/User:Cyantist luckuso] | ||
| − | == | + | ==Solution 3== |
| − | + | [[Image: 2024_AMC_12B_P13.jpeg|thumb|center|600px|]] | |
| − | + | ~Kathan | |
Revision as of 13:45, 14 November 2024
Contents
[hide]Problem 13
There are real numbers ![]() and
and ![]() that satisfy the system of equations
that satisfy the system of equations![]()
![]() What is the minimum possible value of
What is the minimum possible value of ![]() ?
?
![]()
Solution 1 (Easy and Fast)
Adding up the first and second statement, we get h+k with:
= 2x^2 + 2y^2 - 16x - 4y
= 2(x^2 - 8x) + 2(y^2 - 2y)
= 2(x^2 - 8x + 16) - (2)(16) + 2(y^2 - 2y + 1) - (2)(1)
= 2(x - 4)^2 + 2(y - 1)^2 - 34
All squared values must be greater or equal to 0. As we are aiming for the minimum value, we let the 2 squared terms be 0.
This leads to (h+k)min = 0 + 0 - 34 = (C) -34
~mitsuihisashi14
Solution 2 (Coordinate Geometry and HM-GM)
![]()
![]() distance between 2 circle centers is
distance between 2 circle centers is ![]()
![]()
![\[h + k + 54 = (h + 25) + (k + 29) =\sqrt{(h + 25)}^2 + \sqrt{(k + 29)}^2 \geq \frac{\left(\sqrt{h + 25} + \sqrt{k + 29}\right)^2}{2} = \frac{\left(2\sqrt{10}\right)^2}{2} = 20.\]](http://latex.artofproblemsolving.com/4/d/d/4dd6945ff3be5001554b4c445692bbcccb29a178.png) min( h + k ) =
min( h + k ) = ![]() .
.
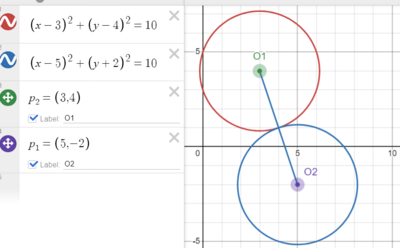
Solution 3
~Kathan