Difference between revisions of "2024 AMC 10A Problems/Problem 22"
(→Solution) |
(→Solution) |
||
| Line 14: | Line 14: | ||
Due to the many similarities present, we can find that AB is <math>4(MQ)</math>, and the height of <math>\Delta ABC</math> is <math>NQ+MN</math> | Due to the many similarities present, we can find that AB is <math>4(MQ)</math>, and the height of <math>\Delta ABC</math> is <math>NQ+MN</math> | ||
| − | AB is <math>4(3/2)=6</math> and the height of <math>\Delta ABC</math> is <math>sqrt3+sqrt3/2= | + | AB is <math>4(3/2)=6</math> and the height of <math>\Delta ABC</math> is <math>\sqrt3+\sqrt3/2=3\sqrt3/2</math>. |
| + | |||
| + | Solving for the area of <math>\Delta ABC</math> gives <math>6*3\sqrt3/2*1/2</math> which is <math>9\sqrt3/2</math> | ||
==See also== | ==See also== | ||
{{AMC10 box|year=2024|ab=A|num-b=21|num-a=23}} | {{AMC10 box|year=2024|ab=A|num-b=21|num-a=23}} | ||
{{MAA Notice}} | {{MAA Notice}} | ||
Revision as of 17:46, 8 November 2024
Problem
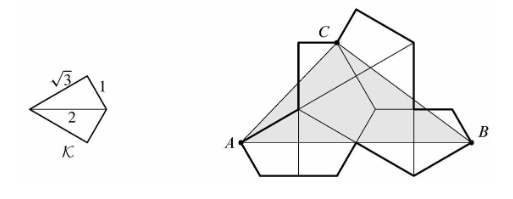
Let ![]() be the kite formed by joining two right triangles with legs
be the kite formed by joining two right triangles with legs ![]() and
and ![]() along a common hypotenuse. Eight copies of
along a common hypotenuse. Eight copies of ![]() are used to form the polygon shown below. What is the area of triangle
are used to form the polygon shown below. What is the area of triangle ![]() ?
?
![]()
Solution
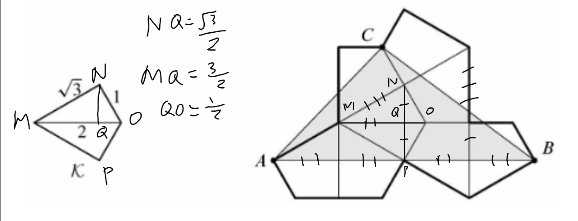
Let ![]() be quadrilateral MNOP. Drawing line MO splits the triangle into
be quadrilateral MNOP. Drawing line MO splits the triangle into ![]() .
Drawing the altitude from N to point Q on line MO, we know NQ is
.
Drawing the altitude from N to point Q on line MO, we know NQ is ![]() , MQ is
, MQ is ![]() , and QO is
, and QO is ![]() .
.
Due to the many similarities present, we can find that AB is ![]() , and the height of
, and the height of ![]() is
is ![]()
AB is ![]() and the height of
and the height of ![]() is
is ![]() .
.
Solving for the area of ![]() gives
gives ![]() which is
which is ![]()
See also
| 2024 AMC 10A (Problems • Answer Key • Resources) | ||
| Preceded by Problem 21 |
Followed by Problem 23 | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 | ||
| All AMC 10 Problems and Solutions | ||
The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions.