2006 AMC 12A Problems/Problem 13
Revision as of 18:53, 5 November 2006 by Xantos C. Guin (talk | contribs) (added link to previous and next problem)
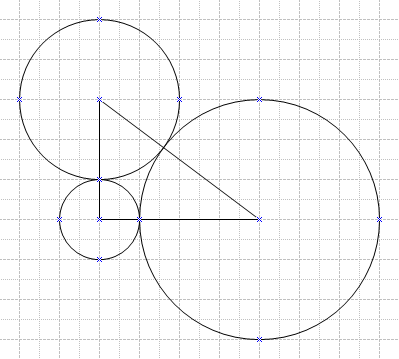
Problem
The vertices of a ![]() right triangle are the centers of three mutually externally tangent circles, as shown. What is the sum of the areas of the three circles?
right triangle are the centers of three mutually externally tangent circles, as shown. What is the sum of the areas of the three circles?
![]()
Solution
Let the radius of the smallest circle be ![]() . We find that the radius of the largest circle is
. We find that the radius of the largest circle is ![]() and the radius of the second largest circle is
and the radius of the second largest circle is ![]() . Thus,
. Thus, ![]() . The radii of the other circles are
. The radii of the other circles are ![]() and
and ![]() . The sum of their areas is
. The sum of their areas is ![]()