Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "2007 AIME II Problems/Problem 11"

m (→‎Solution: change of wording)
m (Box the solution)
 
(One intermediate revision by one other user not shown)
Line 5: Line 5:
 
[[Image:2007 AIME II-11.png]]
 
[[Image:2007 AIME II-11.png]]
  
−
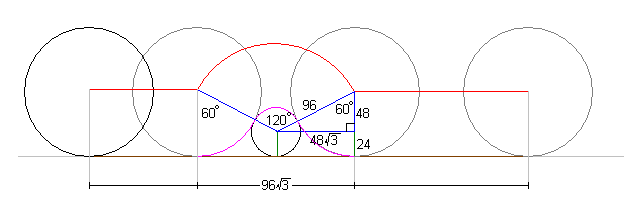
If it weren’t for the small tube, the larger tube would travel <math>\displaystyle 144\pi</math>. Consider the distance from which the larger tube first contacts the smaller tube, until when it completely loses contact with the smaller tube.
+
If it weren’t for the small tube, the larger tube would travel <math>144\pi</math>. Consider the distance from which the larger tube first contacts the smaller tube, until when it completely loses contact with the smaller tube.
  
 
Drawing the radii as shown in the diagram, notice that the [[hypotenuse]] of the [[right triangle]] in the diagram has a length of <math>72 + 24 = 96</math>. The horizontal line divides the radius of the larger circle into <math>72 - 24 = 48</math> on the top half, which indicates that the right triangle has leg of 48 and hypotenuse of 96, a <math>30-60-90 \triangle</math>.  
 
Drawing the radii as shown in the diagram, notice that the [[hypotenuse]] of the [[right triangle]] in the diagram has a length of <math>72 + 24 = 96</math>. The horizontal line divides the radius of the larger circle into <math>72 - 24 = 48</math> on the top half, which indicates that the right triangle has leg of 48 and hypotenuse of 96, a <math>30-60-90 \triangle</math>.  
Line 13: Line 13:
 
The actual horizontal distance it takes can be found by using the <math>30-60-90 \triangle</math>s. The missing leg is equal in length to <math>48\sqrt{3}</math>. Thus, the total horizontal distance covered is <math>96\sqrt{3}</math>.  
 
The actual horizontal distance it takes can be found by using the <math>30-60-90 \triangle</math>s. The missing leg is equal in length to <math>48\sqrt{3}</math>. Thus, the total horizontal distance covered is <math>96\sqrt{3}</math>.  
  
−
Thus, we get <math>144\pi - 64\pi + 96\sqrt{3} = 80\pi + 96\sqrt{3}</math>, and our answer is <math>179</math>.
+
Thus, we get <math>144\pi - 64\pi + 96\sqrt{3} = 80\pi + 96\sqrt{3}</math>, and our answer is <math>\boxed{179}</math>.
  
 
== See also ==
 
== See also ==
Line 19: Line 19:
  
 
[[Category:Intermediate Algebra Problems]]
 
[[Category:Intermediate Algebra Problems]]
 +
{{MAA Notice}}

Latest revision as of 16:31, 29 February 2020

Problem

Two long cylindrical tubes of the same length but different diameters lie parallel to each other on a flat surface. The larger tube has radius $72$ and rolls along the surface toward the smaller tube, which has radius $24$. It rolls over the smaller tube and continues rolling along the flat surface until it comes to rest on the same point of its circumference as it started, having made one complete revolution. If the smaller tube never moves, and the rolling occurs with no slipping, the larger tube ends up a distance $x$ from where it starts. The distance $x$ can be expressed in the form $a\pi+b\sqrt{c},$ where $a,$ $b,$ and $c$ are integers and $c$ is not divisible by the square of any prime. Find $a+b+c.$

Solution

2007 AIME II-11.png

If it weren’t for the small tube, the larger tube would travel $144\pi$. Consider the distance from which the larger tube first contacts the smaller tube, until when it completely loses contact with the smaller tube.

Drawing the radii as shown in the diagram, notice that the hypotenuse of the right triangle in the diagram has a length of $72 + 24 = 96$. The horizontal line divides the radius of the larger circle into $72 - 24 = 48$ on the top half, which indicates that the right triangle has leg of 48 and hypotenuse of 96, a $30-60-90 \triangle$.

Find the length of the purple arc in the diagram (the distance the tube rolled, but not the horizontal distance). The sixty degree central angle indicates to take $\frac{60}{360} = \frac 16$ of the circumference of the larger circle (twice), while the $180 - 2(30) = 120^{\circ}$ central angle in the smaller circle indicates to take $\frac{120}{360} = \frac 13$ of the circumference. This adds up to $2 \cdot \frac 16 144\pi + \frac 13 48\pi = 64\pi$.

The actual horizontal distance it takes can be found by using the $30-60-90 \triangle$s. The missing leg is equal in length to $48\sqrt{3}$. Thus, the total horizontal distance covered is $96\sqrt{3}$.

Thus, we get $144\pi - 64\pi + 96\sqrt{3} = 80\pi + 96\sqrt{3}$, and our answer is $\boxed{179}$.

See also

2007 AIME II (Problems • Answer Key • Resources)
Preceded by
Problem 10 		Followed by
Problem 12
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15
All AIME Problems and Solutions

The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. AMC logo.png

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=2007_AIME_II_Problems/Problem_11&oldid=118573"