Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "2019 IMO Problems/Problem 6"

m (→‎Problem)
(→‎Problem)
Line 2: Line 2:
 
Let <math>I</math> be the incenter of acute triangle <math>ABC</math> with <math>AB \neq AC</math>. The incircle <math>\omega</math> of <math>ABC</math> is tangent to sides <math>BC</math>, <math>CA</math>, and <math>AB</math> at <math>D</math>, <math>E</math>, and <math>F</math>, respectively. The line through <math>D</math> perpendicular to <math>EF</math> meets <math>\omega</math> again at <math>R</math>. Line <math>AR</math> meets ω again at <math>P</math>. The circumcircles of triangles <math>PCE</math> and <math>PBF</math> meet again at <math>Q</math>.
 
Let <math>I</math> be the incenter of acute triangle <math>ABC</math> with <math>AB \neq AC</math>. The incircle <math>\omega</math> of <math>ABC</math> is tangent to sides <math>BC</math>, <math>CA</math>, and <math>AB</math> at <math>D</math>, <math>E</math>, and <math>F</math>, respectively. The line through <math>D</math> perpendicular to <math>EF</math> meets <math>\omega</math> again at <math>R</math>. Line <math>AR</math> meets ω again at <math>P</math>. The circumcircles of triangles <math>PCE</math> and <math>PBF</math> meet again at <math>Q</math>.
 
Prove that lines <math>DI</math> and <math>PQ</math> meet on the line through <math>A</math> perpendicular to <math>AI</math>.
 
Prove that lines <math>DI</math> and <math>PQ</math> meet on the line through <math>A</math> perpendicular to <math>AI</math>.
 +
 +
==Solution==
 +
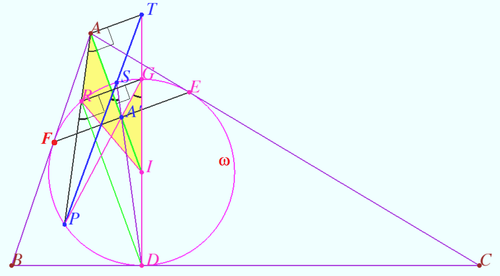
[[File:2019 6 s1.png|500px|right]]
 +
<i><b>Step 1</b></i>
 +
 +
We find an auxiliary point <math>S.</math>
 +
 +
Let <math>G</math> be the antipode of <math>D</math> on <math>\omega, GD = 2R,</math> where <math>R</math> is radius <math>\omega.</math>
 +
 +
We define <math>A' = PG \cap AI.</math>
 +
<math>RD||AI, PRGD</math> cyclic <math>\implies \angle IAP = \angle DRP = \angle DGP.</math>
 +
<math>RD||AI, RD \perp RG, RI=GI \implies \angle AIR = \angle AIG  \implies \triangle AIR \sim \triangle GIA' \implies  \frac {AI}{GI} = \frac {RI}{A'I}\implies A'I \cdot AI = R^2 \implies</math> an inversion with respect <math>omega</math> swap <math>A</math> and <math>A' \implies A'</math> is the midpoint <math>EF.</math>
 +
Let <math>DA'</math> meets <math>\omega</math> again at S (other than D). We define <math>T = PS \cap DI.</math>
 +
Opposite sides of any quadrilateral inscribed in the circle <math>omega</math> meet on the polar line of the intersection of the diagonals with respect to <math>\omega \implies DI</math> and <math>PS</math> meet on the line through <math>A</math> perpendicular to <math>AI.</math>
 +
The problem is reduced to proving that <math>Q \in PST.</math>

Revision as of 12:07, 29 August 2022

Problem

Let $I$ be the incenter of acute triangle $ABC$ with $AB \neq AC$. The incircle $\omega$ of $ABC$ is tangent to sides $BC$, $CA$, and $AB$ at $D$, $E$, and $F$, respectively. The line through $D$ perpendicular to $EF$ meets $\omega$ again at $R$. Line $AR$ meets ω again at $P$. The circumcircles of triangles $PCE$ and $PBF$ meet again at $Q$. Prove that lines $DI$ and $PQ$ meet on the line through $A$ perpendicular to $AI$.

Solution

2019 6 s1.png

Step 1

We find an auxiliary point $S.$

Let $G$ be the antipode of $D$ on $\omega, GD = 2R,$ where $R$ is radius $\omega.$

We define $A' = PG \cap AI.$ $RD||AI, PRGD$ cyclic $\implies \angle IAP = \angle DRP = \angle DGP.$ $RD||AI, RD \perp RG, RI=GI \implies \angle AIR = \angle AIG \implies \triangle AIR \sim \triangle GIA' \implies \frac {AI}{GI} = \frac {RI}{A'I}\implies A'I \cdot AI = R^2 \implies$ an inversion with respect $omega$ swap $A$ and $A' \implies A'$ is the midpoint $EF.$ Let $DA'$ meets $\omega$ again at S (other than D). We define $T = PS \cap DI.$ Opposite sides of any quadrilateral inscribed in the circle $omega$ meet on the polar line of the intersection of the diagonals with respect to $\omega \implies DI$ and $PS$ meet on the line through $A$ perpendicular to $AI.$ The problem is reduced to proving that $Q \in PST.$

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=2019_IMO_Problems/Problem_6&oldid=177655"