Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "2021 USAMO Problems/Problem 1"

(The 2021 USAMO has not been out yet. Please do not edit this page. If you are a site admin, please delete this page.)
(→‎Solution)
(3 intermediate revisions by 2 users not shown)
Line 1: Line 1:
−
The 2021 USAMO has not been out yet. Please do not edit this page. If you are a site admin, please delete this page.
+
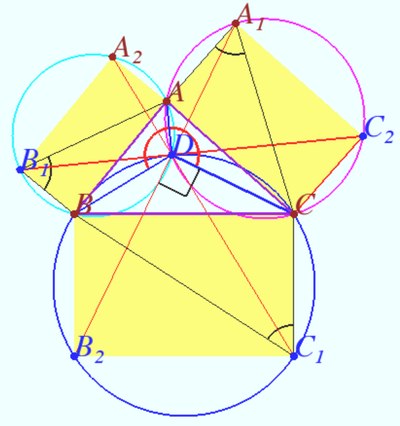
Rectangles <math>BCC_1B_2,</math> <math>CAA_1C_2,</math> and <math>ABB_1A_2</math> are erected outside an acute triangle <math>ABC.</math> Suppose that<cmath>\angle BC_1C+\angle CA_1A+\angle AB_1B=180^{\circ}.</cmath>Prove that lines <math>B_1C_2,</math> <math>C_1A_2,</math> and <math>A_1B_2</math> are concurrent.
 +
 
 +
==Solution==
 +
[[File:2021 USAMO 1.png|400px|right]]
 +
Let <math>D</math> be the second point of intersection of the circles <math>AB_1B</math> and <math>AA_1C.</math> Then
 +
<cmath>\angle ADB = 180^\circ – \angle AB_1B,\angle ADC = 180^\circ – \angle AA_1C \implies</cmath>
 +
<cmath>\angle BDC = 360^\circ – \angle ADB – \angle ADC =</cmath>
 +
<cmath>= 360^\circ – (180^\circ – \angle AB_1B) – (180^\circ – \angle AA_1C) =</cmath>
 +
<cmath>=\angle AB_1B + \angle AA_1C  \implies \angle BDC + \angle BC_1C = 180^\circ \implies</cmath>
 +
<math>BDCC_1B_2</math> is cyclic with diameters <math>BC_1</math> and <math>CB_2 \implies \angle CDB_2 = 90^\circ.</math>
 +
Similarly, <math>\angle CDA_1 = 90^\circ \implies</math> points <math>A_1, D,</math> and <math>B_2</math> are collinear.
 +
 
 +
Similarly, triples of points <math>A_2, D, C_1</math> and <math>C_2, D, B_1</math> are collinear.
 +
 +
(After USAMO 2021 Solution Notes – Evan Chen)
 +
 
 +
'''vladimir.shelomovskii@gmail.com, vvsss'''
 +
 
 +
{{MAA Notice}}

Revision as of 22:55, 18 October 2022

Rectangles $BCC_1B_2,$ $CAA_1C_2,$ and $ABB_1A_2$ are erected outside an acute triangle $ABC.$ Suppose that\[\angle BC_1C+\angle CA_1A+\angle AB_1B=180^{\circ}.\]Prove that lines $B_1C_2,$ $C_1A_2,$ and $A_1B_2$ are concurrent.

Solution

2021 USAMO 1.png

Let $D$ be the second point of intersection of the circles $AB_1B$ and $AA_1C.$ Then \[\angle ADB = 180^\circ – \angle AB_1B,\angle ADC = 180^\circ – \angle AA_1C \implies\] \[\angle BDC = 360^\circ – \angle ADB – \angle ADC =\] \[= 360^\circ – (180^\circ – \angle AB_1B) – (180^\circ – \angle AA_1C) =\] \[=\angle AB_1B + \angle AA_1C \implies \angle BDC + \angle BC_1C = 180^\circ \implies\] $BDCC_1B_2$ is cyclic with diameters $BC_1$ and $CB_2 \implies \angle CDB_2 = 90^\circ.$ Similarly, $\angle CDA_1 = 90^\circ \implies$ points $A_1, D,$ and $B_2$ are collinear.

Similarly, triples of points $A_2, D, C_1$ and $C_2, D, B_1$ are collinear.

(After USAMO 2021 Solution Notes – Evan Chen)

vladimir.shelomovskii@gmail.com, vvsss

The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. AMC logo.png

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=2021_USAMO_Problems/Problem_1&oldid=179231"