Simson line

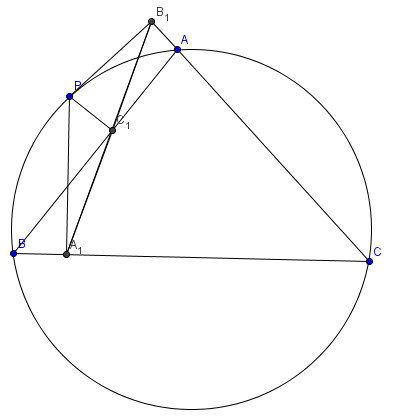
In geometry, given a triangle ABC and a point P on its circumcircle, the three closest points to P on lines AB, AC, and BC are collinear and form a Simson line.

Definition

Let a triangle $\triangle ABC$ and a point $P$ be given.

Let $D, E,$ and $F$ be the foots of the perpendiculars dropped from P to lines AB, AC, and BC, respectively.

Then points $D, E,$ and $F$ are collinear iff the point $P$ lies on circumcircle of $\triangle ABC.$

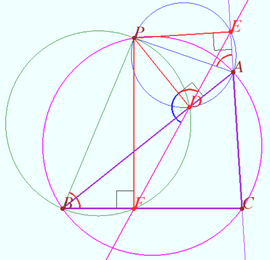
Proof of existence

Let the point $P$ be on the circumcircle of $\triangle ABC.$

$\angle BFP = \angle BDP = 90^\circ \implies$

$BPDF$ is cyclic $\implies \angle PDF = 180^\circ – \angle CBP.$

$\angle ADP = \angle AEP = 90^\circ \implies$

$AEPD$ is cyclic $\implies \angle PDE = \angle PAE.$

$ACBP$ is cyclic $\implies \angle PBC = \angle PAE \implies \angle PDF + \angle PDE = 180^\circ$

$\implies D, E,$ and $F$ are collinear as desired.

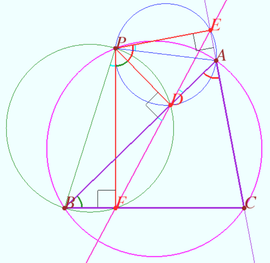
Proof

Let the points $D, E,$ and $F$ be collinear.

$AEPD$ is cyclic $\implies \angle APE = \angle ADE, \angle DPE = \angle BAC.$

$BFDP$ is cyclic $\implies \angle BPF = \angle BDF, \angle DPF = \angle ABC.$

$\angle ADE = \angle BDF \implies \angle BPA = \angle EPF$

$= \angle BAC + \angle ABC = 180^\circ – \angle ACB \implies$

$ACBP$ is cyclis as desired.

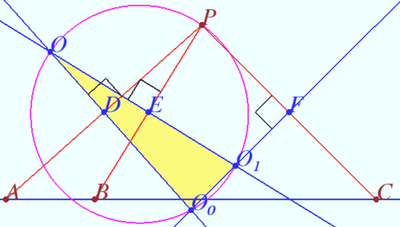
Simson line of a complete quadrilateral

Let four lines made four triangles of a complete quadrilateral. In the diagram these are $\triangle ABC, \triangle ADE, \triangle CEF, \triangle BDF.$

Let $M$ be the Miquel point of a complete quadrilateral.

Let $K, L, N,$ and $G$ be the foots of the perpendiculars dropped from $M$ to lines $AB, AC, EF,$ and $BC,$ respectively.

Prove that points $K,L, N,$ and $G$ are collinear.

Proof

Let $\Omega$ be the circumcircle of $\triangle ABC, \omega$ be the circumcircle of $\triangle CEF.$ Then $M = \Omega \cap \omega.$

Points $K, L,$ and $G$ are collinear as Simson line of $\triangle ABC.$

Points $L, N,$ and $G$ are collinear as Simson line of $\triangle CEF.$

Therefore points $K, L, N,$ and $G$ are collinear, as desired.

Problems

Let the points $A, B,$ and $C$ be collinear and the point $P \notin AB$ . Let $O,O_0,$ and $O_1$ be the circumcenters of triangles $\triangle ABP, \triangle ACP,$ and $\triangle BCP$ . Prove that $P$ lies on circumcircle of $\triangle OO_0O_1$ .