Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Japanese Theorem

Revision as of 13:42, 31 March 2025 by Shalomkeshet (talk | contribs) (Undo revision 230989 by Charking (talk))

The Japanese theorem exists for both cyclic quadrilaterals and cyclic polygons.

Japanese theorem for cyclic quadrilaterals

Definition

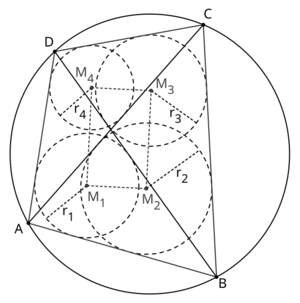
The Japanese theorem for cyclic quadrilaterals states that for a cyclic quadrilateral $ABCD$ and incenters $M_1$, $M_2$, $M_3$, $M_4$ of triangles $\triangle ABD$, $\triangle ABC$, $\triangle BCD$, $\triangle ACD$ the quadrilateral $M_1M_2M_3M_4$ is a rectangle.


Japanese theorem quadrilaterals.png

Proof

From $\triangle ABC$, we can see that

$\angle BM_2C = 90^{\circ} + \frac{1}{2} \angle CAB$

Similarly, from $\triangle BCD$ we have

$\angle BM_3C = 90^{\circ} + \frac{1}{2} \angle CDB$

Since $ABCD$ is cyclic, therefore $\angle CDB = \angle CAB$, which means that

$\angle BM_2C = \angle BM_3C$

From this, it follows that $BM_2M_3C$ is cyclic. This means that

$\angle BCM_3 + \angle BM_2M_3 = 180^{\circ}$

By symmetry, we can also derive

$\angle BAM_1 + \angle BM_2M_1 = 180^{\circ}$

Adding these equations up, we get

$\angle BAM_1 + \angle BCM_3 + \angle BM_2M_1 + \angle BM_2M_3 = 360^{\circ}$

$\Rightarrow \angle BM_2M_1 + \angle BM_2M_3 = 360^{\circ} - \angle BAM_1 - \angle BCM_3 = 360^{\circ} - \frac{1}{2} \left(\angle CAB + \angle CDB \right)$

Which implies

$M_1M_2M_3 = 90^{\circ}$

And other angles similarly.

$Q.E.D.$


Japanese theorem for cyclic polygons

Definition

The Japanese theorem for cyclic polygons states that for any triangulated cyclic polygon, the sum of the | inradii of the triangles is constant.

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=Japanese_Theorem&oldid=246294"