Difference between revisions of "2011 AIME II Problems/Problem 15"
m (→Solution 1) |
|||
| Line 69: | Line 69: | ||
Thus, the answer is <math>61 + 109 + 621 + 39 + 20 = \fbox{850}</math>. | Thus, the answer is <math>61 + 109 + 621 + 39 + 20 = \fbox{850}</math>. | ||
| − | |||
| − | |||
== Solution 2 == | == Solution 2 == | ||
Revision as of 08:32, 12 July 2021
Problem
Let ![]() . A real number
. A real number ![]() is chosen at random from the interval
is chosen at random from the interval ![]() . The probability that
. The probability that ![]() is equal to
is equal to ![]() , where
, where ![]() ,
, ![]() ,
, ![]() ,
, ![]() , and
, and ![]() are positive integers. Find
are positive integers. Find ![]() .
.
Solution 1
Table of values of ![]() :
:

In order for ![]() to hold,
to hold, ![]() must be an integer and hence
must be an integer and hence ![]() must be a perfect square. This limits
must be a perfect square. This limits ![]() to
to ![]() or
or ![]() or
or ![]() since, from the table above, those are the only values of
since, from the table above, those are the only values of ![]() for which
for which ![]() is an perfect square. However, in order for
is an perfect square. However, in order for ![]() to be rounded down to
to be rounded down to ![]() ,
, ![]() must be less than the next perfect square after
must be less than the next perfect square after ![]() (for the said intervals). Now, we consider the three cases:
(for the said intervals). Now, we consider the three cases:
Case ![]() :
:
![]() must be less than the first perfect square after
must be less than the first perfect square after ![]() , which is
, which is ![]() , i.e.:
, i.e.:
![]() (because
(because ![]() implies
implies ![]() )
)
Since ![]() is increasing for
is increasing for ![]() , we just need to find the value
, we just need to find the value ![]() where
where ![]() , which will give us the working range
, which will give us the working range ![]() .
.

So in this case, the only values that will work are ![]() .
.
Case ![]() :
:
![]() must be less than the first perfect square after
must be less than the first perfect square after ![]() , which is
, which is ![]() .
.

So in this case, the only values that will work are ![]() .
.
Case ![]() :
:
![]() must be less than the first perfect square after
must be less than the first perfect square after ![]() , which is
, which is ![]() .
.

So in this case, the only values that will work are ![]() .
.
Now, we find the length of the working intervals and divide it by the length of the total interval, ![]() :
:

Thus, the answer is ![]() .
.
Solution 2
Make the substitution ![]() , so
, so ![]() We're looking for solutions to
We're looking for solutions to ![\[\bigg\lfloor{\sqrt{\frac{y^2-45}{4}}\bigg\rfloor}=\sqrt{\frac{\lfloor{y\rfloor}^2-45}{4}}\]](http://latex.artofproblemsolving.com/7/8/7/787fed2bc80f1555ce140ff37036f1dbc7abd926.png) with the new bounds
with the new bounds ![]() . Since the right side is an integer, it must be that
. Since the right side is an integer, it must be that ![]() is a perfect square. For simplicity, write
is a perfect square. For simplicity, write ![]() and
and ![]() Since
Since ![]() , it must be that
, it must be that ![]() , which gives solutions
, which gives solutions ![]() , respectively. But this gives us three cases to check:
, respectively. But this gives us three cases to check:
Case 1: ![]() .
.
In this case, we have ![]() Case 2:
Case 2: ![]() .
.
In this case, we have ![]() Case 3:
Case 3: ![]()
In this case, we have ![]() To finish, the total length of the interval from which we choose
To finish, the total length of the interval from which we choose ![]() is
is ![]() . The total length of the success intervals is
. The total length of the success intervals is ![]() which means the probability is
which means the probability is ![]() AIMEifying the answer gives
AIMEifying the answer gives ![]() .
.
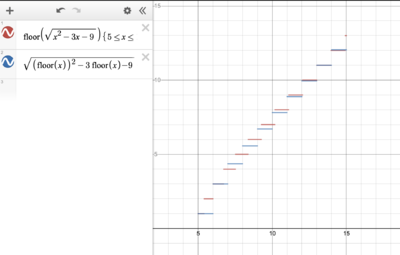
Solution 3 (Graphing)
It's definitely possible to conceptualize this problem visually, if that's your thing, since it is a geometric probability problem. Let ![]() and
and ![]() . The graph of
. The graph of ![]() and
and ![]() will look like this, with
will look like this, with ![]() having only integral y-values and
having only integral y-values and ![]() having only integral x-values:
having only integral x-values:
As both ![]() and
and ![]() consist of a bunch of line segments, the probability that
consist of a bunch of line segments, the probability that ![]() is the "length" of the overlap between the segments of
is the "length" of the overlap between the segments of ![]() and
and ![]() divided by the total length of the segments of
divided by the total length of the segments of ![]() .
.
Looking at the graph, we see that ![]() and
and ![]() will overlap only when
will overlap only when ![]() is an integer. Specifically, each region of overlap will begin when
is an integer. Specifically, each region of overlap will begin when ![]() has solutions for integral
has solutions for integral ![]() in the range of
in the range of ![]() , which consists of the integers
, which consists of the integers ![]() , and end when
, and end when ![]() jumps up to its next y-value.
jumps up to its next y-value.
Using the quadratic formula, we see that the start point of each of these overlapping segments will be the integral values of ![]() for
for ![]() in the specified range, meaning
in the specified range, meaning ![]() must be a perfect square. Plugging in all the possible values of
must be a perfect square. Plugging in all the possible values of ![]() , we get
, we get ![]() , corresponding to start points of
, corresponding to start points of ![]() . As already stated, the endpoints will occur when
. As already stated, the endpoints will occur when ![]() jumps up to the next integer
jumps up to the next integer ![]() at each of these segments, at which point the x-value will be
at each of these segments, at which point the x-value will be ![]() . On the graph, the overlapping segments of
. On the graph, the overlapping segments of ![]() and
and ![]() would be represented by the highlighted green segments below:
would be represented by the highlighted green segments below:
Taking the difference between this second x-value and the start point for each of our start points ![]() and summing them will give us the total length of these green segments. We can then divide this value by ten (the total length of the segments of
and summing them will give us the total length of these green segments. We can then divide this value by ten (the total length of the segments of ![]() ) to give us the probability of overlap between
) to give us the probability of overlap between ![]() and
and ![]() .
.
Doing so gives us:

![]() .
.
~ anellipticcurveoverq
Solution 4
Note that all the "bounds" have to be less than the number+1, otherwise it wouldn't fit the answer format. Therefore, the answer is ![]()
~Lcz
See also
| 2011 AIME II (Problems • Answer Key • Resources) | ||
| Preceded by Problem 14 |
Followed by Last Problem | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 | ||
| All AIME Problems and Solutions | ||
The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions.