Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "Steiner line"

(→‎Collings Clime)
(→‎Steiner line)
Line 1: Line 1:
 
==Steiner line==
 
==Steiner line==
−
Let <math>ABC</math> be a triangle with orthocenter <math>H. S</math> is a point on the circumcircle <math>\Omega</math> of <math>\triangle ABC.</math> Then, the reflections of <math>S</math> in three edges <math>BC, CA, AB</math> and point <math>H</math> lie on a line <math>s</math> which is known as the Steiner line of point <math>S</math> with respect to <math>\triangle ABC.</math>
+
[[File:Steiner and Simson lines.png|500px|right]]
 +
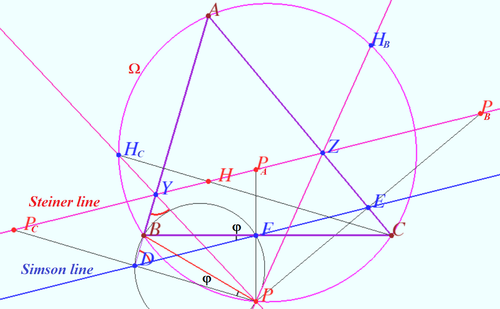
Let <math>ABC</math> be a triangle with orthocenter <math>H. P</math> is a point on the circumcircle <math>\Omega</math> of <math>\triangle ABC.</math>
 +
 +
Let <math>P_A, P_B, </math> and <math>P_C</math> be the reflections of <math>P</math> in three lines which contains edges <math>BC, AC,</math> and <math>AB,</math> respectively.
 +
 
 +
Prove that <math>P_A, P_B, P_C,</math> and <math>H</math> are collinear. Respective line is known as the Steiner line of point <math>P</math> with respect to <math>\triangle ABC.</math>
 +
 
 +
<i><b>Proof</b></i>
 +
 
 +
Let <math>D, E,</math> and <math>F</math> be the foots of the perpendiculars dropped from <math>P</math> to lines <math>AB, AC,</math> and <math>BC,</math> respectively.
 +
 
 +
WLOG, Steiner line cross <math>AB</math> at <math>Y</math> and <math>AC</math> at <math>Z.</math>
 +
 
 +
The line <math>DEF</math> is Simson line of point <math>P</math> with respect of <math>\triangle ABC.</math>
 +
 
 +
<math>D</math> is midpoint of segment <math>PP_C \implies</math> homothety centered at <math>P</math> with ratio <math>2</math> sends point <math>D</math> to a point <math>P_C.</math>
 +
 
 +
Similarly, this homothety sends point <math>E</math> to a point <math>P_B</math>, point <math>F</math> to a point <math>P_A,</math> therefore this homothety send Simson line to line <math>P_AP_BP_C.</math>
 +
 
 +
Let <math>\angle ABC = \beta, \angle BFD = \varphi \implies \angle BDF = \beta – \varphi.</math>
 +
<cmath>P_CP_A||DF \implies \angle P_CYB = \beta – \varphi.</cmath>
 +
<math>P</math> is simmetric to <math>P_C \implies \angle PYD = \beta – \varphi.</math>
 +
 
 +
Quadrungle <math>BDPF</math> is cyclic <math>\implies \angle BPD = \varphi \implies \angle BPY = 90^\circ – \angle BYP – \angle BPD = 90^\circ – \beta.</math>
 +
 
 +
<math>\angle BCH = \angle BPY \implies PY \cap CH</math> at point <math>H_C \in \Omega.</math>
 +
Similarly, line <math>BH \cap PZ</math> at <math>H_B \in \Omega.</math>
 +
 
 +
According the Collins Claim <math>YZ</math> is <math>H-line,</math> therefore <math>H \in P_AP_B.</math>
 +
 
 +
'''vladimir.shelomovskii@gmail.com, vvsss'''
 +
 
 
==Collings Clime==
 
==Collings Clime==
 
[[File:Steiner H line.png|500px|right]]
 
[[File:Steiner H line.png|500px|right]]

Revision as of 11:09, 7 December 2022

Steiner line

Steiner and Simson lines.png

Let $ABC$ be a triangle with orthocenter $H. P$ is a point on the circumcircle $\Omega$ of $\triangle ABC.$

Let $P_A, P_B,$ and $P_C$ be the reflections of $P$ in three lines which contains edges $BC, AC,$ and $AB,$ respectively.

Prove that $P_A, P_B, P_C,$ and $H$ are collinear. Respective line is known as the Steiner line of point $P$ with respect to $\triangle ABC.$

Proof

Let $D, E,$ and $F$ be the foots of the perpendiculars dropped from $P$ to lines $AB, AC,$ and $BC,$ respectively.

WLOG, Steiner line cross $AB$ at $Y$ and $AC$ at $Z.$

The line $DEF$ is Simson line of point $P$ with respect of $\triangle ABC.$

$D$ is midpoint of segment $PP_C \implies$ homothety centered at $P$ with ratio $2$ sends point $D$ to a point $P_C.$

Similarly, this homothety sends point $E$ to a point $P_B$, point $F$ to a point $P_A,$ therefore this homothety send Simson line to line $P_AP_BP_C.$

Let $\angle ABC = \beta, \angle BFD = \varphi \implies \angle BDF = \beta – \varphi.$ \[P_CP_A||DF \implies \angle P_CYB = \beta – \varphi.\] $P$ is simmetric to $P_C \implies \angle PYD = \beta – \varphi.$

Quadrungle $BDPF$ is cyclic $\implies \angle BPD = \varphi \implies \angle BPY = 90^\circ – \angle BYP – \angle BPD = 90^\circ – \beta.$

$\angle BCH = \angle BPY \implies PY \cap CH$ at point $H_C \in \Omega.$ Similarly, line $BH \cap PZ$ at $H_B \in \Omega.$

According the Collins Claim $YZ$ is $H-line,$ therefore $H \in P_AP_B.$

vladimir.shelomovskii@gmail.com, vvsss

Collings Clime

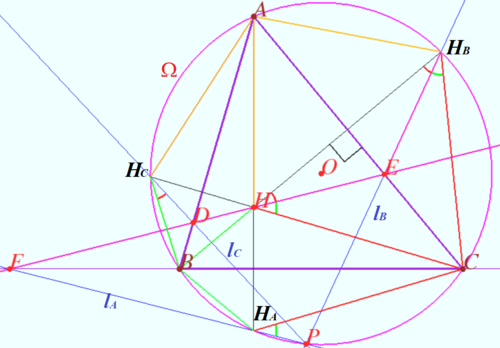
Steiner H line.png

Let triangle $ABC$ be the triangle with the orthocenter $H$ and circumcircle $\Omega.$ Denote $H–line$ any line containing point $H.$

Let $l_A, l_B,$ and $l_C$ be the reflections of $H-line$ in the edges $BC, AC,$ and $AB,$ respectively.

Prove that lines $l_A, l_B,$ and $l_C$ are concurrent and the point of concurrence lies on $\Omega.$

Proof

Let $D, E,$ and $F$ be the crosspoints of $H–line$ with $AB, AC,$ and $BC,$ respectively.

WLOG $D \in AB, E \in AC.$ Let $H_A, H_B,$ and $H_C$ be the points symmetric to $H$ with respect $BC, AC,$ and $AB,$ respectively.

Therefore $H_A \in l_A, H_B \in l_B, H_C \in l_C,$ \[AH = AH_B = AH_C, BH = BH_A = BH_C, CH = CH_A = CH_B \implies\] \[\angle HH_BE = \angle EHH_B = \angle BHD = \angle BH_CD.\]

Let $P$ be the crosspoint of $l_B$ and $l_C \implies BH_CH_BP$ is cyclic $\implies P \in \Omega.$

Similarly $\angle CH_BE = \angle CHE = \angle CH_A \implies CH_BH_AP$ is cyclic $\implies P \in \Omega \implies$ the crosspoint of $l_B$ and $l_A$ is point $P.$

Usually the point $P$ is called the anti-Steiner point of the $H-line$ with respect to $\triangle ABC.$

vladimir.shelomovskii@gmail.com, vvsss

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=Steiner_line&oldid=183703"