Difference between revisions of "Simson line"

Revision as of 11:26, 7 December 2022

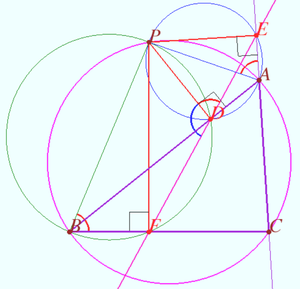
In geometry, given a triangle ABC and a point P on its circumcircle, the three closest points to P on lines AB, AC, and BC are collinear.

Simson line (main)

Let a triangle $\triangle ABC$ and a point $P$ be given.

Let $D, E,$ and $F$ be the foots of the perpendiculars dropped from P to lines AB, AC, and BC, respectively.

Then points $D, E,$ and $F$ are collinear iff the point $P$ lies on circumcircle of $\triangle ABC.$

Proof

Let the point $P$ be on the circumcircle of $\triangle ABC.$

$\angle BFP = \angle BDP = 90^\circ \implies$

$BPDF$ is cyclic $\implies \angle PDF = 180^\circ – \angle CBP.$

$\angle ADP = \angle AEP = 90^\circ \implies$

$AEPD$ is cyclic $\implies \angle PDE = \angle PAE.$

$ACBP$ is cyclic $\implies \angle PBC = \angle PAE \implies \angle PDF + \angle PDE = 180^\circ$

$\implies D, E,$ and $F$ are collinear as desired.

Proof

Let the points $D, E,$ and $F$ be collinear.

$AEPD$ is cyclic $\implies \angle APE = \angle ADE, \angle APE = \angle BAC.$

$BFDP$ is cyclic $\implies \angle BPF = \angle BDF, \angle DPF = \angle ABC.$

$\angle ADE = \angle BDF \implies \angle BPA = \angle EPF$

$= \angle BAC + \angle ABC = 180^\circ – \angle ACB \implies$

$ACBP$ is cyclis as desired.

vladimir.shelomovskii@gmail.com, vvsss

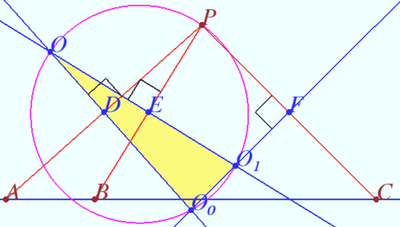
Simson line of a complete quadrilateral

Let four lines made four triangles of a complete quadrilateral. In the diagram these are $\triangle ABC, \triangle ADE, \triangle CEF, \triangle BDF.$ Let $M$ be the Miquel point of a complete quadrilateral. Let $D, E, F,$ and $G$ be the foots of the perpendiculars dropped from $M$ to lines $AB, AC, BC,$ and EF, respectively.

Prove that points $D, E, F,$ and $G$ are collinear.

Proof

Miquel point

vladimir.shelomovskii@gmail.com, vvsss

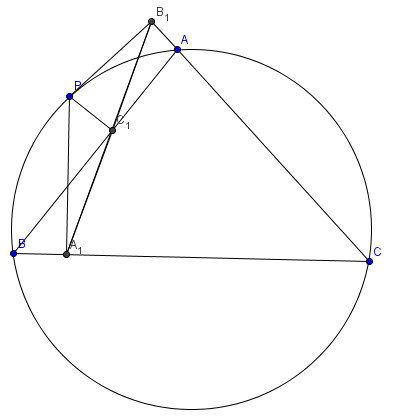
Problem

Let the points $A, B,$ and $C$ be collinear and the point $P \notin AB.$

Let $O,O_0,$ and $O_1$ be the circumcenters of triangles $\triangle ABP, \triangle ACP,$ and $\triangle BCP.$

Prove that $P$ lies on circumcircle of $\triangle OO_0O_1.$

Proof

Let $D, E,$ and $F$ be the midpoints of segments $AB, AC,$ and $BC,$ respectively.

Then points $D, E,$ and $F$ are collinear $(DE||AB, EF||DC).$

$PD \perp OO_0, PE \perp OO_1, PF \perp O_0O_1 \implies$ $DEF$ is Simson line of $\triangle OO_0O_1 \implies P$ lies on circumcircle of $\triangle OO_0O_1$ as desired.

vladimir.shelomovskii@gmail.com, vvsss

@@ Line 40: / Line 40: @@
 <math>ACBP</math> is cyclis as desired.
+'''vladimir.shelomovskii@gmail.com, vvsss'''
+==Simson line of a complete quadrilateral==
+Let four lines made four triangles of a complete quadrilateral. In the diagram these are <math>\triangle ABC, \triangle ADE, \triangle CEF, \triangle BDF.</math>
+Let <math>M</math> be the Miquel point of a complete quadrilateral. Let <math>D, E, F,</math> and <math>G</math> be the foots of the perpendiculars dropped from <math>M</math> to lines <math>AB, AC, BC,</math> and EF, respectively.
+Prove that points <math>D, E, F,</math> and <math>G</math> are collinear.
+<i><b>Proof</b></i>
+*[[Miquel point]]
 '''vladimir.shelomovskii@gmail.com, vvsss'''

Page

Toolbox

Search

Difference between revisions of "Simson line"

Revision as of 11:26, 7 December 2022

Simson line (main)

Simson line of a complete quadrilateral

Problem