Difference between revisions of "2016 IMO Problems/Problem 1"
(→Solution) |
(→Solution) |
||
| Line 9: | Line 9: | ||
[[File:2016IMOQ1Solution.jpg|600px]] | [[File:2016IMOQ1Solution.jpg|600px]] | ||
| − | + | The Problem shows that | |
| − | + | And | |
| − | + | Finally | |
~Athmyx | ~Athmyx | ||
Revision as of 12:32, 20 April 2024
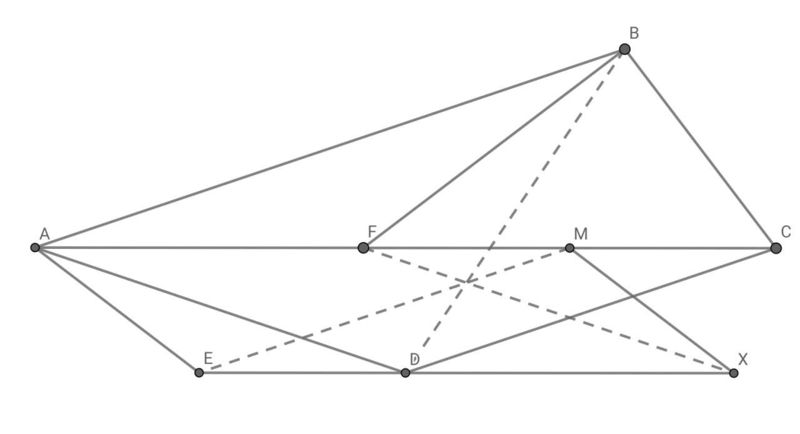
Problem
Triangle ![]() has a right angle at
has a right angle at ![]() . Let
. Let ![]() be the point on line
be the point on line ![]() such that
such that ![]() and
and ![]() lies between
lies between ![]() and
and ![]() . Point
. Point ![]() is chosen so that
is chosen so that ![]() and
and ![]() is the bisector of
is the bisector of ![]() . Point
. Point ![]() is chosen so that
is chosen so that ![]() and
and ![]() is the bisector of
is the bisector of ![]() . Let
. Let ![]() be the midpoint of
be the midpoint of ![]() . Let
. Let ![]() be the point such that
be the point such that ![]() is a parallelogram. Prove that
is a parallelogram. Prove that ![]() and
and ![]() are concurrent.
are concurrent.
Solution
The Problem shows that
And
Finally
~Athmyx
See Also
| 2016 IMO (Problems) • Resources | ||
| Preceded by First Problem |
1 • 2 • 3 • 4 • 5 • 6 | Followed by Problem 2 |
| All IMO Problems and Solutions | ||