Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "Surface of constant width"

(→‎Reuleaux triangle analogue)
(→‎Rotation of the Reuleaux triangle)
Line 32: Line 32:
  
 
==Rotation of the Reuleaux triangle==
 
==Rotation of the Reuleaux triangle==
−
[[File:Solid Reuleaux triangle rotation.png|390px|right]]
+
[[File:Solid Reuleaux triangle rotation.png|430px|right]]
 
The left part of diagram shows the curve <math>ABC</math> which is a Reuleaux triangle determined by the center <math>O</math> and the vertex <math>A.</math>
 
The left part of diagram shows the curve <math>ABC</math> which is a Reuleaux triangle determined by the center <math>O</math> and the vertex <math>A.</math>
  
Line 38: Line 38:
  
 
The right part of the diagram shows the surface which arose as the result of the rotation <math>ABC.</math>  
 
The right part of the diagram shows the surface which arose as the result of the rotation <math>ABC.</math>  
 +
 +
'''vladimir.shelomovskii@gmail.com, vvsss'''
 +
==Rotation of the Reuleaux triangle analogue==
 +
[[File:Surface Reuleaux triangle analogy construction.png|490px|right]]
 +
The left part of diagram shows the curve <math>G</math> which is a Reuleaux triangle analogy determined by the center <math>O,</math> the vertex <math>A</math> and point <math>G</math> on curve analogue.
 +
 +
Similarly, we use the Reuleaux triangle <math>AB'C'</math> is constructed by the rotation of the curve <math>G</math> around axis <math>AO</math> and get the second position of the  Reuleaux triangle analogue.
 +
 +
The middle part of the diagram shows part of the surface. We can see all four parts of this surface shown by different colors.
 +
 +
The right part of the diagram shows the surface which arose as the result of the rotation curve <math>G.</math> It is impossible see the down side of the surface (blue) in this view.
  
 
'''vladimir.shelomovskii@gmail.com, vvsss'''
 
'''vladimir.shelomovskii@gmail.com, vvsss'''

Revision as of 07:49, 10 August 2024

A curve of constant width is a simple closed curve in the plane whose width (the distance between parallel supporting lines) is the same in all directions. A surface of constant width (orbiform) is a convex form whose width, measured by the distance between two opposite parallel planes touching its boundary, is the same regardless of the direction of those two parallel planes.

Reuleaux triangle

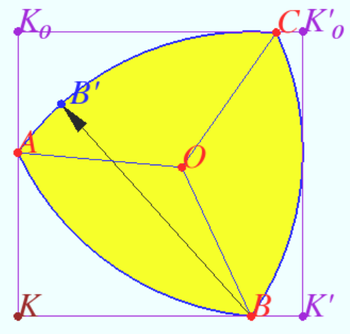
Reuleaux triangle.png

The boundary of a Reuleaux triangle is a constant width curve based on an equilateral triangle.

Let $\triangle ABC$ be equilateral triangle.

Let $\overset{\Large\frown} {AC}$ be the arc centered at $B$ with radius $BB' = BA, \angle ABC = 60^\circ.$

Arcs $\overset{\Large\frown} {AB}$ and $\overset{\Large\frown} {BC}$ define similarly.

All points on this arcs are equidistant from the opposite vertex.

Distance is $|AB|.$

vladimir.shelomovskii@gmail.com, vvsss

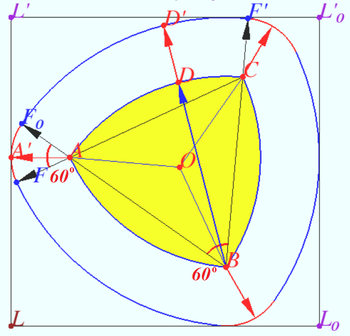
Reuleaux triangle analogue

Reuleaux triangle analogue.png

Let $ABCD$ be the boundary of a Reuleaux triangle, $O$ be the centroid of $\triangle ABC.$

Let $\overset{\Large\frown} {FF_0}$ be the arc centered at $A$ with radius $AA', \angle FAF_0 = 60^\circ,$ points $F, A, C$ and $F_0, A, B$ are collinear.

Let $\overset{\Large\frown} {F_0F'}$ be the arc centered at $B$ with radius \[|AA'| + |AB|,\] $\angle F'AF_0 = \angle ABC = 60^\circ,$ points $F', C, B$ are collinear.

Similarly define arcs from point $F'$ to $F.$

The width of this curve is \[|AB| + 2|AA'|.\]

vladimir.shelomovskii@gmail.com, vvsss

Rotation of the Reuleaux triangle

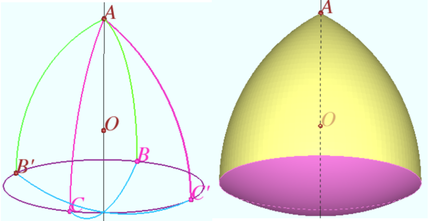
Solid Reuleaux triangle rotation.png

The left part of diagram shows the curve $ABC$ which is a Reuleaux triangle determined by the center $O$ and the vertex $A.$

The Reuleaux triangle $AB'C'$ is constructed by the rotation of the curve $ABC$ around axis $AO.$

The right part of the diagram shows the surface which arose as the result of the rotation $ABC.$

vladimir.shelomovskii@gmail.com, vvsss

Rotation of the Reuleaux triangle analogue

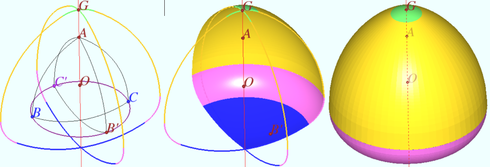
Surface Reuleaux triangle analogy construction.png

The left part of diagram shows the curve $G$ which is a Reuleaux triangle analogy determined by the center $O,$ the vertex $A$ and point $G$ on curve analogue.

Similarly, we use the Reuleaux triangle $AB'C'$ is constructed by the rotation of the curve $G$ around axis $AO$ and get the second position of the Reuleaux triangle analogue.

The middle part of the diagram shows part of the surface. We can see all four parts of this surface shown by different colors.

The right part of the diagram shows the surface which arose as the result of the rotation curve $G.$ It is impossible see the down side of the surface (blue) in this view.

vladimir.shelomovskii@gmail.com, vvsss

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=Surface_of_constant_width&oldid=224863"