Difference between revisions of "Squeeze Theorem"
(rmv) |
|||
| Line 1: | Line 1: | ||
| − | |||
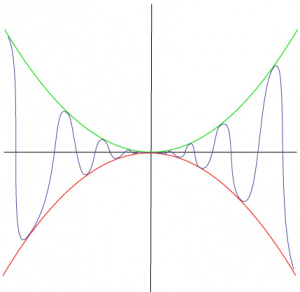
The '''Squeeze Theorem''' (also called the '''Sandwich Theorem''' or the '''Squeeze Play Theorem''') is a relatively simple [[theorem]] that deals with [[calculus]], specifically [[limit]]s. | The '''Squeeze Theorem''' (also called the '''Sandwich Theorem''' or the '''Squeeze Play Theorem''') is a relatively simple [[theorem]] that deals with [[calculus]], specifically [[limit]]s. | ||
Revision as of 20:50, 19 May 2008
The Squeeze Theorem (also called the Sandwich Theorem or the Squeeze Play Theorem) is a relatively simple theorem that deals with calculus, specifically limits.
Contents
[hide]Theorem
Suppose ![]() is between
is between ![]() and
and ![]() for all
for all ![]() in a neighborhood of the point
in a neighborhood of the point ![]() . If
. If ![]() and
and ![]() approach some common limit
approach some common limit ![]() as
as ![]() approaches
approaches ![]() , then
, then ![]() .
.
Proof
If ![]() is between
is between ![]() and
and ![]() for all
for all ![]() in the neighborhood of
in the neighborhood of ![]() , then either
, then either ![]() or
or ![]() for all
for all ![]() in this neighborhood. The two cases are the same up to renaming our functions, so assume without loss of generality that
in this neighborhood. The two cases are the same up to renaming our functions, so assume without loss of generality that ![]() .
.
We must show that for all ![]() there is some
there is some ![]() for which
for which ![]() implies
implies ![]() .
.
Now since ![]() , there must exist
, there must exist ![]() such that
such that
![]()
Now let ![]() . If
. If ![]() then
then
![]()
So ![]() . Now by the definition of a limit we get
. Now by the definition of a limit we get ![]() as desired.
as desired.
Applications and examples