Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "Mock AIME 2 2006-2007 Problems/Problem 7"

(→‎Solution)
 
(9 intermediate revisions by 3 users not shown)
Line 1: Line 1:
 
== Problem ==
 
== Problem ==
  
−
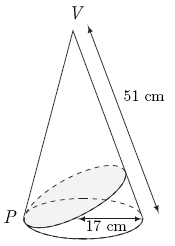
A right circular cone of base radius <math>17</math>cm and slant height <math>34</math>cm is given. <math>P</math> is a point on the circumference of the base and the shortest path from <math>P</math> around the cone and back is drawn (see diagram). If the length of this path is <math>m\sqrt{n},</math> where <math>m</math> and <math>n</math> are relatively prime positive integers, find <math>m+n.</math>
+
A right circular cone of base radius <math>17</math>cm and slant height <math>51</math>cm is given. <math>P</math> is a point on the circumference of the base and the shortest path from <math>P</math> around the cone and back is drawn (see diagram). If the length of this path is <math>m\sqrt{n},</math> where <math>n</math> is squarefree, find <math>m+n.</math>
  
 
[[Image:Mock_AIME_2_2007_Problem8.jpg]]
 
[[Image:Mock_AIME_2_2007_Problem8.jpg]]
  
 
==Solution==
 
==Solution==
−
{{solution}}
+
"Unfolding" this cone results in a circular sector with radius <math>51</math> and arc length <math>17\cdot 2\pi=34\pi</math>. Let the vertex of this sector be <math>O</math>. The problem is then reduced to finding the shortest distance between the two points <math>A</math> and <math>B</math> on the arc that are the farthest away from each other. Since <math>34\pi</math> is <math>1/3</math> of the circumference of a circle with radius <math>51</math>, we must have that <math>\angle AOB=\frac{360^{\circ}}{3}=120^{\circ}</math>. We know that <math>AO=OB=51</math>, so we can use the Law of Cosines to find the length of <math>AB</math>:
 +
<cmath>AB=\sqrt{AO^2+OB^2-2AO\cdot OB\cdot\cos{120^{\circ}}}=\sqrt{51^2+51^2+51^2}=51\sqrt{3}.</cmath>
 +
Hence <math>m=51</math>, <math>n=3</math>, <math>m+n=\boxed{054}</math>.
  
−
We begin by noticing that that the shortest path from a point on the base to and around the cone is the perpendicular from the aforementioned point to the slant line. We can now construct two equations based on this fact. Let x be the length from the point on the other end of the diameter from P to the point at which P is perpendicular to the slant. Let y now be the diameter of the shaded circle. We now have two equations : <math>x_{}^{2} + y_{}^{2} = 34_{}^{}</math> and <math>y_{}^{2} + (34 - x)_{}^{2} = 34_{}^{2}</math>. We can tell from this equation that <math>34 - x = x</math>, so <math>x = 17</math>. From this we can deduce that y = <math>17\sqrt(3)</math>.
+
==See Also==
−
 
+
{{Mock AIME box|year=2006-2007|n=2|num-b=6|num-a=8}}
−
 
 
−
 
 
−
 
 
−
 
 
−
----
 
−
 
 
−
*[[Mock AIME 2 2006-2007/Problem 6 | Previous Problem]]
 
−
 
 
−
*[[Mock AIME 2 2006-2007/Problem 8 | Next Problem]]
 
−
 
 
−
*[[Mock AIME 2 2006-2007]]
 
  
 
[[Category:Intermediate Geometry Problems]]
 
[[Category:Intermediate Geometry Problems]]

Latest revision as of 17:10, 10 July 2014

Problem

A right circular cone of base radius $17$cm and slant height $51$cm is given. $P$ is a point on the circumference of the base and the shortest path from $P$ around the cone and back is drawn (see diagram). If the length of this path is $m\sqrt{n},$ where $n$ is squarefree, find $m+n.$

Mock AIME 2 2007 Problem8.jpg

Solution

"Unfolding" this cone results in a circular sector with radius $51$ and arc length $17\cdot 2\pi=34\pi$. Let the vertex of this sector be $O$. The problem is then reduced to finding the shortest distance between the two points $A$ and $B$ on the arc that are the farthest away from each other. Since $34\pi$ is $1/3$ of the circumference of a circle with radius $51$, we must have that $\angle AOB=\frac{360^{\circ}}{3}=120^{\circ}$. We know that $AO=OB=51$, so we can use the Law of Cosines to find the length of $AB$: \[AB=\sqrt{AO^2+OB^2-2AO\cdot OB\cdot\cos{120^{\circ}}}=\sqrt{51^2+51^2+51^2}=51\sqrt{3}.\] Hence $m=51$, $n=3$, $m+n=\boxed{054}$.

See Also

Mock AIME 2 2006-2007 (Problems, Source)
Preceded by
Problem 6 		Followed by
Problem 8
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15
Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=Mock_AIME_2_2006-2007_Problems/Problem_7&oldid=62481"