Difference between revisions of "1977 Canadian MO Problems/Problem 2"
(box) |
(→Solution 2) |
||
| (2 intermediate revisions by 2 users not shown) | |||
| Line 4: | Line 4: | ||
== Solution == | == Solution == | ||
| − | {{ | + | If <math>AB</math> is the chord perpendicular to <math>OX</math> through point <math>P</math>, then extend <math>AO</math> to meet the circle at point <math>C</math>. It is now evident that <math>O</math> is the midpoint of <math>AC</math>, <math>X</math> is the midpoint of <math>AB</math>, and hence <math>OX=\dfrac{BC}{2}</math>. |
| + | |||
| + | Similarly, let <math>P</math> be a point on arc <math>AB</math>. Extend <math>PO</math> to meet the circle at point <math>R</math>. Extend <math>PX</math> to meet the circle a second time at <math>Q</math>. | ||
| + | |||
| + | We now plot <math>S</math> on <math>XQ</math> such that <math>XS=XP</math>. Then, <math>OX=\dfrac{RS}{2}</math>. Since <math>\angle RQS=90</math>, <math>RS>RQ</math>. Hence, <math>RQ<\dfrac{OX}{2}</math>, and therefore, <math>\angle OPX=\angle OAX=\angle RPQ</math>. | ||
| + | |||
| + | Ergo, the points <math>P</math> such that <math>\angle OPA</math> is maximized are none other than points <math>A</math> and <math>B</math>. <math>\Box</math> | ||
| + | |||
| + | ==Solution 2== | ||
| + | Let <math>XY</math> be the chord perpendicular to <math>OA</math> through <math>A</math>. We claim that the choices of <math>P</math> that maximize <math>\angle{OPA}</math> are <math>X</math> and <math>Y</math>. | ||
| + | |||
| + | Let <math>\omega</math> be the circumcircle of <math>OPA</math>. Then clearly <math>\angle{OPA}</math> is maximized if the radius of <math>\omega</math> is minimized, which occurs if <math>\omega</math> is internally tangent to circle <math>O</math>. Hence <math>OP</math> is a diameter of <math>\omega</math> (whose center <math>OP</math> is collinear with), and so <math>\angle{OAP} = 90^\circ</math>. It follows that <math>P = X</math> or <math>Y</math>, as desired. | ||
{{Old CanadaMO box|num-b=1|num-a=3|year=1977}} | {{Old CanadaMO box|num-b=1|num-a=3|year=1977}} | ||
Latest revision as of 22:28, 21 September 2014
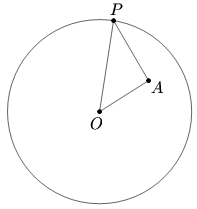
Let ![]() be the center of a circle and
be the center of a circle and ![]() be a fixed interior point of the circle different from
be a fixed interior point of the circle different from ![]() Determine all points
Determine all points ![]() on the circumference of the circle such that the angle
on the circumference of the circle such that the angle ![]() is a maximum.
is a maximum.
Solution
If ![]() is the chord perpendicular to
is the chord perpendicular to ![]() through point
through point ![]() , then extend
, then extend ![]() to meet the circle at point
to meet the circle at point ![]() . It is now evident that
. It is now evident that ![]() is the midpoint of
is the midpoint of ![]() ,
, ![]() is the midpoint of
is the midpoint of ![]() , and hence
, and hence ![]() .
.
Similarly, let ![]() be a point on arc
be a point on arc ![]() . Extend
. Extend ![]() to meet the circle at point
to meet the circle at point ![]() . Extend
. Extend ![]() to meet the circle a second time at
to meet the circle a second time at ![]() .
.
We now plot ![]() on
on ![]() such that
such that ![]() . Then,
. Then, ![]() . Since
. Since ![]() ,
, ![]() . Hence,
. Hence, ![]() , and therefore,
, and therefore, ![]() .
.
Ergo, the points ![]() such that
such that ![]() is maximized are none other than points
is maximized are none other than points ![]() and
and ![]() .
. ![]()
Solution 2
Let ![]() be the chord perpendicular to
be the chord perpendicular to ![]() through
through ![]() . We claim that the choices of
. We claim that the choices of ![]() that maximize
that maximize ![]() are
are ![]() and
and ![]() .
.
Let ![]() be the circumcircle of
be the circumcircle of ![]() . Then clearly
. Then clearly ![]() is maximized if the radius of
is maximized if the radius of ![]() is minimized, which occurs if
is minimized, which occurs if ![]() is internally tangent to circle
is internally tangent to circle ![]() . Hence
. Hence ![]() is a diameter of
is a diameter of ![]() (whose center
(whose center ![]() is collinear with), and so
is collinear with), and so ![]() . It follows that
. It follows that ![]() or
or ![]() , as desired.
, as desired.
| 1977 Canadian MO (Problems) | ||
| Preceded by Problem 1 |
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • | Followed by Problem 3 |