Difference between revisions of "DVI exam"
(→2024 Problem 18 (EGE)) |
(→2024 var 246 Problem 5) |
||
| (32 intermediate revisions by the same user not shown) | |||
| Line 162: | Line 162: | ||
<i><b>Answer:<math> min(f) = 8 \sqrt{5}, a = 2, x = \frac {\pi}{2} + 4k \pi.</math></b></i> | <i><b>Answer:<math> min(f) = 8 \sqrt{5}, a = 2, x = \frac {\pi}{2} + 4k \pi.</math></b></i> | ||
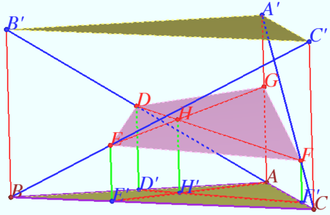
| − | ==2020 201 problem 6== | + | ==2020 var 201 problem 6== |
[[File:2020 201 6.png|330px|right]] | [[File:2020 201 6.png|330px|right]] | ||
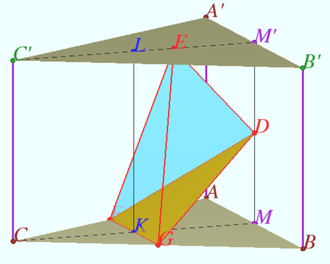
Let a triangular prism <math>ABCA'B'C'</math> with a base <math>ABC</math> be given, <math>D \in AB', E \in BC', F \in CA'.</math> Find the ratio in which the plane <math>DEF</math> divides the segment <math>AA',</math> if <math>AD : DB' = 1 : 1,</math> <cmath>BE : EC' = 1 : 2, CF : FA' = 1 : 3.</cmath> | Let a triangular prism <math>ABCA'B'C'</math> with a base <math>ABC</math> be given, <math>D \in AB', E \in BC', F \in CA'.</math> Find the ratio in which the plane <math>DEF</math> divides the segment <math>AA',</math> if <math>AD : DB' = 1 : 1,</math> <cmath>BE : EC' = 1 : 2, CF : FA' = 1 : 3.</cmath> | ||
| Line 180: | Line 180: | ||
Answer: <math>AG : GA' = 4 : 3.</math> | Answer: <math>AG : GA' = 4 : 3.</math> | ||
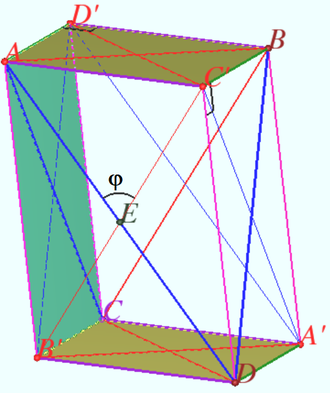
| − | ==2020 202 problem 6== | + | ==2020 var 202 problem 6== |
[[File:2020 202 6.png|330px|right]] | [[File:2020 202 6.png|330px|right]] | ||
Let a tetrahedron <math>ABCD</math> be given, <math>AB = BC = CD = 5, CA = AD = DB = 6.</math> Find the cosine of the angle <math>\varphi</math> between the edges <math>BC</math> and <math>AD.</math> | Let a tetrahedron <math>ABCD</math> be given, <math>AB = BC = CD = 5, CA = AD = DB = 6.</math> Find the cosine of the angle <math>\varphi</math> between the edges <math>BC</math> and <math>AD.</math> | ||
| Line 199: | Line 199: | ||
Answer: <math>\frac {11}{30}. </math> | Answer: <math>\frac {11}{30}. </math> | ||
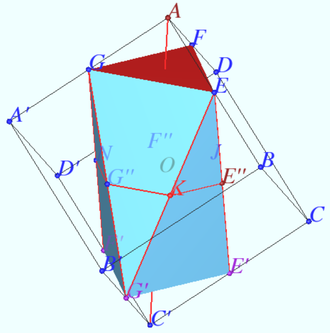
| − | + | ==2020 var 203 problem 6== | |
| − | |||
| − | ==2020 203 problem 6== | ||
[[File:2020 203 6 3.png|330px|right]] | [[File:2020 203 6 3.png|330px|right]] | ||
[[File:2020 203 6 2.png|330px|right]] | [[File:2020 203 6 2.png|330px|right]] | ||
| Line 225: | Line 223: | ||
Answer: <math>\frac {1}{3}.</math> | Answer: <math>\frac {1}{3}.</math> | ||
| − | ==2020 204 problem 6== | + | ==2020 var 204 problem 6== |
[[File:2020 204 6.png|300px|right]] | [[File:2020 204 6.png|300px|right]] | ||
| Line 240: | Line 238: | ||
Answer: <math>\frac {12}{1 + \sqrt{13}}.</math> | Answer: <math>\frac {12}{1 + \sqrt{13}}.</math> | ||
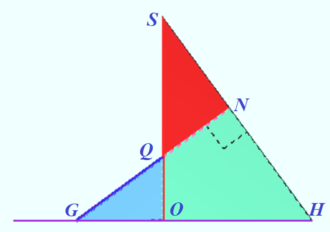
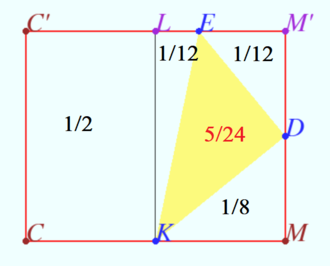
| − | ==2020 205 problem 6== | + | ==2020 var 205 problem 6== |
[[File:2020 205 6.png|330px|right]] | [[File:2020 205 6.png|330px|right]] | ||
Let the quadrangular pyramid <math>ABCDS</math> with the base parallelogram <math>ABCD</math> be given. | Let the quadrangular pyramid <math>ABCDS</math> with the base parallelogram <math>ABCD</math> be given. | ||
| Line 263: | Line 261: | ||
<cmath>\frac {[AEGFS]}{[ABDS]} = \frac {[AEFS]+[GEFS]}{[ABDS]} = \frac{2}{9} + \frac{4}{63} = \frac{2}{7} \implies \frac {[AEGFS]}{[ABCDS]} = \frac{1}{7}.</cmath> | <cmath>\frac {[AEGFS]}{[ABDS]} = \frac {[AEFS]+[GEFS]}{[ABDS]} = \frac{2}{9} + \frac{4}{63} = \frac{2}{7} \implies \frac {[AEGFS]}{[ABCDS]} = \frac{1}{7}.</cmath> | ||
Answer: 1 : 6. | Answer: 1 : 6. | ||
| − | ==2020 206 problem 6== | + | |
| + | ==2020 var 206 problem 6== | ||
[[File:2020 206 6.png|330px|right]] | [[File:2020 206 6.png|330px|right]] | ||
Given a cube <math>ABCDA'B'C'D'</math> with the base <math>ABCD</math> and side edges <math>AA', BB', CC', DD' =1.</math> Find the distance between the line passing through the midpoints of the edges <math>AB</math> and <math>AA'</math> and the line passing through the midpoints of the edges <math>BB'</math> and <math>B'C'.</math> | Given a cube <math>ABCDA'B'C'D'</math> with the base <math>ABCD</math> and side edges <math>AA', BB', CC', DD' =1.</math> Find the distance between the line passing through the midpoints of the edges <math>AB</math> and <math>AA'</math> and the line passing through the midpoints of the edges <math>BB'</math> and <math>B'C'.</math> | ||
| Line 288: | Line 287: | ||
Answer: <math>\frac {1}{\sqrt{3}}</math> | Answer: <math>\frac {1}{\sqrt{3}}</math> | ||
| − | ==2021 215 problem 7== | + | ==2021 var 215 problem 7== |
The sphere touches all edges of the tetrahedron <math>ABCD.</math> It is known that the products of the lengths of crossing edges are equal. It is also known that <math>AB = 3, BC = 1.</math> Find <math>AC.</math> | The sphere touches all edges of the tetrahedron <math>ABCD.</math> It is known that the products of the lengths of crossing edges are equal. It is also known that <math>AB = 3, BC = 1.</math> Find <math>AC.</math> | ||
| Line 310: | Line 309: | ||
Answer: 3. | Answer: 3. | ||
| − | ==2022 221 problem 7== | + | ==2022 var 221 problem 7== |
[[File:MSU 2022 7.png|330px|right]] | [[File:MSU 2022 7.png|330px|right]] | ||
[[File:MSU 2022 7a.png|330px|right]] | [[File:MSU 2022 7a.png|330px|right]] | ||
| Line 332: | Line 331: | ||
<i><b>Answer: 5.</b></i> | <i><b>Answer: 5.</b></i> | ||
| − | ==2022 222 problem 7== | + | ==2022 var 222 problem 7== |
[[File:MSU 2022 2 7.png|400px|right]] | [[File:MSU 2022 2 7.png|400px|right]] | ||
A sphere of diameter <math>1</math> is inscribed in a pyramid at the base of which lies a rhombus with an acute angle <math>2\alpha</math> and side <math>\sqrt{6}.</math> Find the angle <math>2\alpha</math> if it is known that all lateral faces of the pyramid are inclined to plane of its base at an angle of <math>60^\circ.</math> | A sphere of diameter <math>1</math> is inscribed in a pyramid at the base of which lies a rhombus with an acute angle <math>2\alpha</math> and side <math>\sqrt{6}.</math> Find the angle <math>2\alpha</math> if it is known that all lateral faces of the pyramid are inclined to plane of its base at an angle of <math>60^\circ.</math> | ||
| Line 351: | Line 350: | ||
<cmath>\sin {2 \alpha} = \frac {SM}{AB} = \frac {\sqrt{3}}{\sqrt{6}} = \frac {1}{\sqrt{2}}.</cmath> | <cmath>\sin {2 \alpha} = \frac {SM}{AB} = \frac {\sqrt{3}}{\sqrt{6}} = \frac {1}{\sqrt{2}}.</cmath> | ||
<i><b>Answer:<math> \frac {\pi}{4}.</math></b></i> | <i><b>Answer:<math> \frac {\pi}{4}.</math></b></i> | ||
| − | ==2022 | + | |
| + | ==2022 var 222 problem 6== | ||
Find all possible values of the product <math>xy</math> if it is known that <math>x,y \in \left [ 0, \frac{\pi}{2} \right)</math> and it is true | Find all possible values of the product <math>xy</math> if it is known that <math>x,y \in \left [ 0, \frac{\pi}{2} \right)</math> and it is true | ||
<cmath>\frac{1 - \sin(x - y)}{1 - \cos(x - y)}= \frac{1 - \sin(x + y)}{1 - \cos(x + y)}.</cmath> | <cmath>\frac{1 - \sin(x - y)}{1 - \cos(x - y)}= \frac{1 - \sin(x + y)}{1 - \cos(x + y)}.</cmath> | ||
| Line 366: | Line 366: | ||
<i><b>Answer:<math>0.</math></b></i> | <i><b>Answer:<math>0.</math></b></i> | ||
| − | + | ==2022 var 224 problem 6== | |
| − | ==2022 | ||
Find all triples of real numbers <math>(x,y,z)</math> in the interval <math>\left ( 0; \frac {\pi}{2} \right)</math> satisfying the system of equations | Find all triples of real numbers <math>(x,y,z)</math> in the interval <math>\left ( 0; \frac {\pi}{2} \right)</math> satisfying the system of equations | ||
<cmath>\begin{equation} \left\{ \begin{aligned} | <cmath>\begin{equation} \left\{ \begin{aligned} | ||
| Line 390: | Line 389: | ||
<i><b>Answer:<math>\left (\frac {\pi}{4},\frac {\pi}{4},\frac {\pi}{4} \right ).</math></b></i> | <i><b>Answer:<math>\left (\frac {\pi}{4},\frac {\pi}{4},\frac {\pi}{4} \right ).</math></b></i> | ||
| − | ==2023 | + | ==2023 var 231 problem 6== |
Let positive numbers <math>a,b,c</math> be such that <math>\frac {1}{a+1} + \frac {1}{b+1} +\frac {1}{c+1} = 1. </math> | Let positive numbers <math>a,b,c</math> be such that <math>\frac {1}{a+1} + \frac {1}{b+1} +\frac {1}{c+1} = 1. </math> | ||
| Line 414: | Line 413: | ||
<cmath>F'(x_0) = \frac {2 - x_0^2}{(x_0^2 + 2)^2} = -\frac{1}{18} = G'(x_0) = m \cdot g'(x_0) = - \frac {m}{(1+ x_0)^2} = -\frac{m}{9} \implies m = \frac{1}{2}, k = \frac {1}{6}.</cmath> | <cmath>F'(x_0) = \frac {2 - x_0^2}{(x_0^2 + 2)^2} = -\frac{1}{18} = G'(x_0) = m \cdot g'(x_0) = - \frac {m}{(1+ x_0)^2} = -\frac{m}{9} \implies m = \frac{1}{2}, k = \frac {1}{6}.</cmath> | ||
| − | ==2023 | + | ==2023 var 231 EM problem 6== |
<cmath>F(x) = log_{\frac{5}{2}} (2 + \cos x) \cdot log_{\frac{5}{2}} (3 - \cos x).</cmath> | <cmath>F(x) = log_{\frac{5}{2}} (2 + \cos x) \cdot log_{\frac{5}{2}} (3 - \cos x).</cmath> | ||
Find the maximum value <math>F_m = max (F(x))</math> and all argument values <math>x_0</math> such that <math>F_m = F(x_0)</math>. | Find the maximum value <math>F_m = max (F(x))</math> and all argument values <math>x_0</math> such that <math>F_m = F(x_0)</math>. | ||
| Line 426: | Line 425: | ||
Therefore <cmath>F_m = 1, \cos x_0 = \frac {1}{2}, x_0 = \pm \frac{\pi}{3} + 2 k \pi.</cmath> | Therefore <cmath>F_m = 1, \cos x_0 = \frac {1}{2}, x_0 = \pm \frac{\pi}{3} + 2 k \pi.</cmath> | ||
| − | + | ==2023 var 232 problem 6== | |
| − | ==2023 | ||
Let positive numbers <math>a,b,c</math> be such that <cmath>\left ( a+b+c \right) \cdot \left ( \frac {1}{a} + \frac {1}{b} +\frac {1}{c} \right ) = 10.</cmath> | Let positive numbers <math>a,b,c</math> be such that <cmath>\left ( a+b+c \right) \cdot \left ( \frac {1}{a} + \frac {1}{b} +\frac {1}{c} \right ) = 10.</cmath> | ||
Find the maximum value of <math>\frac {a+ b}{c}.</math> | Find the maximum value of <math>\frac {a+ b}{c}.</math> | ||
| Line 445: | Line 443: | ||
<i><b>Answer:<math>4.</math></b></i> | <i><b>Answer:<math>4.</math></b></i> | ||
| − | ==2023 | + | ==2023 var 233 problem 6== |
Let positive numbers <math>a,b,c</math> be such that <math>a^2 + b^2 + c^2 = 1.</math> | Let positive numbers <math>a,b,c</math> be such that <math>a^2 + b^2 + c^2 = 1.</math> | ||
| Line 489: | Line 487: | ||
<i><b>Answer: </b></i> <math>(- \infty,0 ]\cup (\frac{1}{3}, \infty ).</math> | <i><b>Answer: </b></i> <math>(- \infty,0 ]\cup (\frac{1}{3}, \infty ).</math> | ||
| + | ==2024 Test problem 7== | ||
| + | Find all values of the parameter a for which there is at least one solution to the inequality <cmath>\frac {1}{x} + \frac{2}{a} \le \frac {3}{a - x} - \frac{1}{x+a}</cmath> on the interval <math>x \in [2,3]</math> | ||
| + | |||
| + | <i><b>Solution</b></i> | ||
| + | |||
| + | <math>\frac {1}{x} + \frac{2}{a} \le \frac {3}{a - x} - \frac{1}{x+a} \leftrightarrow F(x,a) \ge 0,</math> where <math>F(x,a) = (a + 2x) \cdot\left( \frac{2}{a^2-x^2}-\frac{1}{ax} \right) = \frac {2(x+a/2)(x+a_1) (x-a_2)}{ax(x+a)(a - x)},</math> where <math>a_1 = a(\sqrt{2}+1), a_2= a(\sqrt{2}-1).</math> | ||
| + | |||
| + | The equation <math>F(x,a) = 0</math> has solutions <math>x= -\frac{a}{2}, x = -a_1,</math> and <math>x = a_2.</math> | ||
| + | <cmath>F(2,a) = \frac {(4+a)(2+a_1) (2-a_2)}{2a(2+a)(a -2)}.</cmath> | ||
| + | <math>F(2,a) \ge 0</math> if <math>a \in [-4, -2) \cup [2-2\sqrt{2},0) \cup (2, 2 \sqrt{2}+2],</math> so given inequality has the solution <math>x=2</math> for these <math>a.</math> | ||
| + | <cmath>F(3,a) = \frac {(6+a)(3+a_1) (3-a_2)}{3a(3+a)(a -3)}.</cmath> | ||
| + | <math>F(3,a) \ge 0</math> if <math>a \in [-6, -3) \cup [3-3\sqrt{2},0) \cup (3, 3 \sqrt{2}+3],</math> so given inequality has the solution <math>x=3</math> for these <math>a.</math> | ||
| + | |||
| + | <math>a \in (-\infty, -6), x \in [2,3] \implies F(x,a) < 0,</math> no solution of the given inequality. | ||
| + | |||
| + | <math>F(x,-2) < 0</math> no solution of the inequality if <math>a = -2.</math> | ||
| + | |||
| + | <math>a \in (-2, 3(1 - \sqrt{2})).</math> If <math>x \in [2,3] F(x,a) < 0 \implies</math> no solution of the inequality. | ||
| + | |||
| + | <math>a \in (0, 2). </math> If <math>x \in [2,3] F(x,a) < 0 \implies</math> no solution of the given inequality. | ||
| + | |||
| + | <math>a \in (3 + 3\sqrt{2},\infty).</math> If <math>x \in [2,3] F(a,x) < 0 \implies</math> no solution of the given inequality. | ||
| + | |||
| + | ==2024 var 241 Problem 2== | ||
| + | The natural numbers <math>a_1,...a_n</math> form a strictly increasing arithmetic progression. Find all possible values of <math>n</math> if it is known that <math>n</math> is odd, <math>n > 1</math> and <math>a_1 + a_2+...+a_n = 2024.</math> | ||
| + | |||
| + | <i><b>Solution</b></i> | ||
| + | |||
| + | <math>n</math> is odd, so <math>a_1 + a_2+...+a_n = n a_\frac{n+1}{2} = 2024 = 8 \cdot 11 \cdot 23.</math> | ||
| + | |||
| + | Let <math>n = 11 \implies a_6 = 8 \cdot 23 = 184,</math> the common difference may be <math>1,</math> increasing arithmetic progression exist. | ||
| + | |||
| + | Let <math>n = 23 \implies a_{12} = 8 \cdot 11 = 88,</math> the common difference may be <math>1,</math> increasing arithmetic progression exist. | ||
| + | |||
| + | Let <math>n = 11 \cdot 23 = 253 \implies a_{127} = 8 \implies a_{100} < 0</math> can not be the natural number. | ||
| + | |||
| + | Answer: <math>11,23.</math> | ||
| + | |||
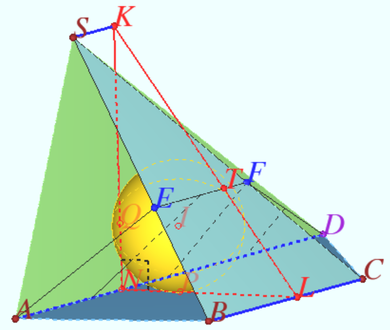
| + | ==2024 var 242 Problem 7== | ||
| + | [[File:2024 1 problem 7.png|390px|right]] | ||
| + | The base of the pyramid is the trapezoid <math>ABCD, AD||BC, AD = 2BC.</math> | ||
| + | |||
| + | A sphere of radius <math>1</math> touches the plane of the base of the pyramid and the planes of its lateral faces <math>ADS</math> and <math>BCS</math> at points <math>P,Q,</math> and <math>T,</math> respectively. | ||
| + | |||
| + | Find the ratio in which the volume of the pyramid is divided by the plane <math>ADT,</math> if the face <math>ADS</math> is perpendicular to the plane <math>ABD</math> and the height of the pyramid is <math>4.</math> | ||
| + | |||
| + | <i><b>Solution</b></i> | ||
| + | |||
| + | A sketch of the given pyramid is shown in the diagram. The planes <math>SAD</math> and <math>SBC</math> intersect along the straight line <math>SK||AD||BC,</math> that is, the planes <math>ABCD, SBC, SAD</math> form the lateral surface of a prism into which a sphere with center at point <math>I</math> is inscribed. | ||
| + | |||
| + | The plane <math>\pi</math> containing the point <math>I</math> and perpendicular to <math>AD</math> contains points <math>P, Q,</math> and <math>T.</math> Plane <math>\pi</math> intersects parallel lines <math>AD, BC,</math> and <math>SK</math> at points <math>N,L,</math> and <math>K,</math> respectively. | ||
| + | |||
| + | Let <math>EF</math> be the line parallel to <math>AD, E \in BS, F \in CS.</math> The plane <math>AETFD</math> cuts off the pyramid <math>SAEFD</math> with volume <math>v</math> from the pyramid <math>SABCD</math> with volume <math>V.</math> | ||
| + | |||
| + | <math>KN \perp AD</math> and equal to the distance from <math>S</math> to <math>AD, KN = 4, LN \perp AD, LN \perp KN</math> and equal to the distance between <math>BC</math> and <math>AD.</math> | ||
| + | <cmath>V = \frac {1}{3} KN \cdot KL \cdot \frac { AD + BC}{2} = BC \cdot \frac {KN \cdot KL}{2} = BC \cdot [KNL].</cmath> | ||
| + | Consider a right triangle <math>KLN ([KLN]</math> is the area of <math>\triangle KLN)</math> into which a circle <math>PQT</math> with radius <math>r = 1</math> is inscribed. | ||
| + | <cmath>QN = PN = r, KQ = KN - NQ = 3 = KT, x = TL = PL \implies</cmath> | ||
| + | <cmath>(x+1)^2 + 4^2 = (x+3)^2 \implies x = 2 \implies \frac {EF}{BC} = \frac {KT}{KL} = \frac {3}{5}.</cmath> | ||
| + | We are looking for <math>v.</math> Let <math>h</math> be the distance from <math>S</math> to the plane <math>AETFD.</math> | ||
| + | <cmath>v = \frac {1}{3} h \cdot NT \cdot \frac {AD + EF}{2} = BC \cdot (2 + \frac{3}{5}) \cdot \frac {h \cdot NT}{2} = BC \cdot \frac{13}{15} \cdot [KNT].</cmath> | ||
| + | <cmath>\frac {[KNT]} {[KNL]} = \frac {KT}{KL} = \frac {3}{5} \implies \frac {v}{V} = \frac{13}{15} \cdot \frac{3}{5} = \frac{13}{25} \implies \frac {v}{V-v} = \frac{13}{12}.</cmath> | ||
| + | Answer: <math>13 : 12.</math> | ||
| + | |||
| + | ==2024 var 243 Problem 6== | ||
| + | Solve the system of equations in the positive <math>x, y, z:</math> | ||
| + | <cmath>\left\{ | ||
| + | |||
| + | <i><b>Solution (after Natalia Zakharova)</b></i> | ||
| + | <cmath>x^4 + x^2\cdot y^2 + y^4 = (x^2 + xy + y^2) \cdot (x^2 - xy + y^2),</cmath> | ||
| + | <cmath>y^4 + y^2 \cdot z^2 + z^4 = (y^2 + yz + z^2) \cdot (y^2 - yz + z^2),</cmath> | ||
| + | <cmath>z^4 + z^2\cdot x^2 + x^4 = (x^2 + xz + z^2) \cdot (x^2 - xz + z^2) \implies</cmath> | ||
| + | <cmath>\frac {(x^4 + x^2\cdot y^2 + y^4) (y^4 + y^2 \cdot z^2 + z^4)(z^4 + z^2\cdot x^2 + x^4)}{(x^2 + xy + y^2) (y^2 + yz + z^2)(z^2 + zx + x^2)} = (x^2 - xy + y^2) (y^2 - yz + z^2)(z^2 - zx + x^2) = (xyz)^2.</cmath> | ||
| + | <cmath>x^2 - xy + y^2 \ge 2xy - xy = xy, y^2 - yz + z^2 \ge yz, z^2 - zx + x^2 \ge zx \implies</cmath> | ||
| + | <cmath>(xyz)^2 = (x^2 - xy + y^2) (y^2 - yz + z^2)(z^2 - zx + x^2) \ge xy \cdot yz \cdot zx = (xyz)^2 \implies x = y = z \implies x = \frac{1}{3}.</cmath> | ||
| + | Answer: <math>x = y = z = \frac{1}{3}.</math> | ||
| + | |||
| + | ==2024 var 244 Problem 7== | ||
| + | [[File:2024 244 problem 7.png|320px|right]] | ||
| + | [[File:2024 244 problem 7a.png|320px|right]] | ||
| + | Let <math>ABCDA'B'C'D'</math> be the cube, <math>AB = 1</math>. Let <math>K \in A'B', L \in B'B,</math> | ||
| + | <math>M \in BC, N \in CD, Q \in DD', P \in A'D', \angle A'AK = \angle LAK,</math> | ||
| + | <cmath>\angle BAM = \angle MAN, \angle DAQ = \angle PAQ,</cmath> | ||
| + | <cmath>A'K + LB = BM + ND = DQ + PA' = \frac {5}{4}.</cmath> | ||
| + | |||
| + | Find the ratio in which the plane <math>KMQ</math> divides the volume of the cube. | ||
| + | |||
| + | <i><b>Solution</b></i> | ||
| + | |||
| + | 1. Let <math>F</math> lie on the ray <math>B'A', A'F = BL.</math> | ||
| + | <cmath>AB = AA', \angle ABL = \angle AA'F \implies \triangle AA'F = \triangle ABL \implies AL = AF.</cmath> | ||
| + | <cmath>\angle KAF = \angle A'AF + \angle KAA' = \angle BAL + \angle KAA' =</cmath> | ||
| + | <cmath>= 90^\circ - \angle KAL = 90^\circ - \angle KAA' = \angle AKF \implies</cmath> | ||
| + | <cmath>AF = KF = KA' + BL = \frac {5}{4} = AL.</cmath> | ||
| + | So <math>BL = \sqrt{AL^2 – AB^2} = \frac {3}{4} \implies KA' = KB' = \frac {1}{2}.</math> | ||
| + | |||
| + | Similarly, <math>M</math> is the midpoint <math>BC, Q</math> is the midpoint <math>DD'.</math> | ||
| + | |||
| + | 2. <math>KQ = KM = QM, AQ = AK = AM = C'M = C'K = C'Q =\frac {\sqrt{5}}{2} \implies </math> | ||
| + | |||
| + | regular pyramids are equal <math>AKMQ = C'KMQ.</math> So <math>O</math> (midpoint <math>AC'</math>) lies in plane <math>KMQ.</math> | ||
| + | |||
| + | Let <math>M'</math> be the midpoint <math>A'D' \implies M'</math> symmetric to <math>M</math> with respect <math>O,</math> so <math>M' \in KMQ.</math> | ||
| + | |||
| + | Similarly <math>K' \in KMQ, Q' \in KMQ,</math> where <math>K'</math> midpoint <math>CD, Q'</math> the midpoint <math>BB'.</math> | ||
| + | |||
| + | For each point on the edges of the solid forming a part of the cube cut off by a plane <math>KMQ</math> from the side of vertex <math>A,</math> one can find a point symmetrical relative to the center of the cube <math>O</math> on the edges of the solid forming another part of the cube. | ||
| + | |||
| + | It means that these parts are congruent and the plane <math>KMQ</math> divides the cube in half. | ||
| + | |||
| + | Answer: <math>1:1.</math> | ||
| + | ==2024 var 245 Problem 6== | ||
| + | Let <math>a,b,c,</math> and <math>d</math> be the positive real numbers such that <math>a+b+c+d = 1.</math> Find the minimal value of <math> \frac {a^2}{1-a} + \frac {b^2}{1-b}+ \frac {c^2}{1-c} + \frac {d^2}{1-d}.</math> | ||
| + | |||
| + | <i><b>Solution</b></i> | ||
| + | |||
| + | <cmath> \frac {a^2}{1-a} + a = \frac {a}{1-a} = \frac{a}{b+c+d} = \frac{1}{\frac{b}{a}+\frac {c}{a}+\frac{d}{a}} \le \frac{1}{9} \left( \frac{a}{b}+\frac {a}{c}+\frac{a}{d}\right).</cmath> | ||
| + | For the last transform we use unequality between the harmonic mean and the arithmetic mean for three numbers. | ||
| + | Therefore | ||
| + | <cmath> \frac {a^2}{1-a} + \frac {b^2}{1-b}+ \frac {c^2}{1-c} + \frac {d^2}{1-d} \le \frac{1}{9} \left( \frac{a}{b}+\frac{b}{a}+\frac {a}{c}+\frac{c}{a}+\frac{a}{d}+\frac{d}{a}+\frac{b}{c}+\frac{c}{b}+\frac{b}{d}+\frac{d}{b}+\frac{c}{d}+\frac{d}{c}\right) - a - b - c - d \le \frac{12}{9} - 1 = \frac{1}{3}.</cmath> | ||
| + | Equality we get if <math>a = b = c = d = \frac {1}{4}.</math> | ||
| + | |||
| + | Answer: <math>\frac{1}{3}.</math> | ||
| + | ==2024 var 246 Problem 5== | ||
| + | [[File:2024 var 246 5.png|390px|right]] | ||
| + | Let <math>\triangle ABC</math> be given, <math>\angle BAC > 90^\circ.</math> Point <math>D</math> is located on side <math>BC</math> so that <math>AC = CD,</math> the circle <math>\odot ACD</math> touches <math>AB</math> at point <math>A.</math> | ||
| + | |||
| + | Point <math>E</math> is located on ray <math>AD</math> so that <math>CE = EA = AB.</math> | ||
| + | |||
| + | Find the ratio of <math>BC : AB.</math> | ||
| + | |||
| + | <i><b>Solution</b></i> | ||
| + | |||
| + | <cmath>\frac {\overset{\Large\frown} {AD}}{2} = \angle BAD = \angle ACB.</cmath> | ||
| + | Triangles <math>\triangle CAD</math> and <math>\triangle EAC</math> are isosceles with a common angle <math>\angle DAC \implies</math> | ||
| + | <cmath>\angle AEC = \angle ACD = \angle BAD \implies AB||CE.</cmath> | ||
| + | <math>AB = CE, AB||CE \implies ABEC</math> is the parallelogram <math>\implies BC = 2 BD.</math> | ||
| + | <cmath>\triangle ABD \sim \triangle CBA \implies \frac {AB}{BC} = \frac {BD}{AB} \implies 2 AB^2 = BC^2 \implies \frac {BC}{AB} = \sqrt{2}.</cmath> | ||
| + | Answer: <math>\sqrt{2}.</math> | ||
| + | ==2024 var 246 Problem 6== | ||
| + | |||
| + | <math>f(x) = x^4 – 12x^3 +ax^2+bx +81,</math> where <math>a</math> and <math>b</math> are real numbers. | ||
| + | |||
| + | Find <math>f(5)</math> if <math>f(x) = (x - c_1)(x - c_2)(x - c_3)(x – c_4)</math> where | ||
| + | |||
| + | 1) <math>c_i, i = 1..4</math> are real numbers, | ||
| + | |||
| + | 2) <math>c_i, i = 1..4</math> are positive numbers. | ||
| + | |||
| + | <i><b>Solution (after Natalia Zakharova)</b></i> | ||
| + | |||
| + | 1) <math>f(x) = (x^2 - t^2)(x^2 - 12x - \frac{9^2}{t^2})</math> has four real roots | ||
| + | <cmath>c_1 = t, c_2 = -t, c_3 = 6 + \sqrt{36 + 81/t^2}, c_4 = 6 - \sqrt{36 + 81/t^2}</cmath> | ||
| + | if <math>t \ne 0.</math> | ||
| + | <cmath>f(5) = (t^2 - 25)(35 + \frac{9^2}{t^2}) = 35t^2 - \frac{2025}{t^2} - 794.</cmath> | ||
| + | <math>F(5)</math> can take any real values. There are positive and negative roots <math>c_1 \cdot c_2 < 0.</math> | ||
| + | |||
| + | 2) <math>c_1 + c_2 + c_3 + c_4 = 12, c_1 \cdot c_2 \cdot c_3 \cdot c_4 = 81,</math> | ||
| + | <cmath>\frac {c_1 + c_2 + c_3 + c_4}{4} = 3 = \sqrt[4]{c_1 \cdot c_2 \cdot c_3 \cdot c_4} \implies c_1 = c_2 = c_3 = c_4 = 3 \implies f(x) = (x-3)^4, f(5) = 2^4 = 16.</cmath> | ||
| + | Answer: <math>1)</math> any real number, <math>2) 16.</math> | ||
| + | |||
| + | ==2024 var 246 Problem 7== | ||
| + | [[File:2024 var 246 7.png|300px|right]] | ||
| + | The distance from the midpoint <math>M</math> of the height <math>SO</math> of a regular quadrangular pyramid <math>SABCD</math> to the lateral face is <math>MF' = \sqrt{2}</math> and to the lateral edge is <math>ME' = \sqrt{3}.</math> | ||
| + | |||
| + | Find the volume of the pyramid. | ||
| + | |||
| + | <i><b>Solution</b></i> | ||
| + | |||
| + | Let <math>K</math> be the midpoint <math>AB, OF \perp SK, OE \perp AS \implies</math> | ||
| + | <cmath>EO = 2 E'M = 2 \sqrt{3}, FO = 2 F'M = 2 \sqrt{3}.</cmath> | ||
| + | Denote <math>AB = 2a, SO = h \implies KO = a, AO = a \sqrt{2}.</math> | ||
| + | |||
| + | Let us express the heights of right triangles through their legs: | ||
| + | <cmath>\frac {1}{EO^2} = \frac {1}{AO^2} + \frac {1}{SO^2} \implies \frac {1}{12} = \frac {1}{2a^2} + \frac {1}{h^2}.</cmath> | ||
| + | <cmath>\frac {1}{FO^2} = \frac {1}{KO^2} + \frac {1}{SO^2} \implies \frac {1}{8} = \frac {1}{a^2} + \frac {1}{h^2}.</cmath> | ||
| + | <cmath>\frac {1}{2a^2} = \frac {1}{8} - \frac {1}{12} = \frac {1}{24} \implies a^2 = 12.</cmath> | ||
| + | <cmath>\frac {1}{h^2} = \frac {1}{8} - \frac {1}{12} = \frac {1}{24} \implies h = 2 \sqrt{6} \implies V = \frac {h \cdot 4a^2}{3} = 32 \sqrt{6}.</cmath> | ||
| + | Answer: <math>32 \sqrt{6}.</math> | ||
| + | |||
| + | ==2024 var 247 Problem 6== | ||
| + | [[File:2024 7 problem 6.png|300px|right]] | ||
| + | Real numbers <math>a, b,</math> and <math>c</math> satisfy the system of equations | ||
| + | <cmath>\left\{ | ||
| + | Find the largest possible value of <math>c.</math> | ||
| + | |||
| + | <i><b>Solution</b></i> | ||
| + | |||
| + | In coordinates <math>a, b,</math> and <math>c</math> the first equation defines the plane <math>ABC,</math> the second - a sphere with the center at the origin. They are shown in the diagram. | ||
| + | |||
| + | The solution of the given system (if it exists) is a circle symmetrical with respect to the plane <math>a = b.</math> This plane intersects the plane of the first equation along the line <math>CED</math> on which the points of maximum (E) and minimum (D) of the values of <math>c</math> are located. | ||
| + | |||
| + | At these points the system takes the form | ||
| + | <cmath>\left\{ | ||
| + | <cmath>(4 - c)^2 = 4a^2 = 2(8 - c^2) \implies c^2 - 8c = - 2 c^2.</cmath> | ||
| + | These system has two solutions <math>c_1 = 0, c_2 = \frac {8}{3},</math> so solution of the given system exist. | ||
| + | |||
| + | Answer: <math>\frac {8}{3}.</math> | ||
| + | |||
| + | ==2024 var 247 Problem 7== | ||
| + | [[File:2024 247 problem 7.png|300px|right]] | ||
| + | Let the cube <math>ABCDA'B'C'D'</math> be given. Let points <math>K \in A'B', L \in BC, M \in CD,</math> and <math>N \in A'D'</math> be given, <math>A'K = BL, A'N = DM.</math> | ||
| + | |||
| + | Let the plane <math>A'BD</math> cross the plane <math>ALN</math> by the line <math>\ell,</math> and cross the plane <math>AKM</math> by line <math>\ell'.</math> | ||
| + | |||
| + | Find the angle between <math>\ell</math> and <math>\ell'.</math> | ||
| + | |||
| + | <i><b>Solution</b></i> | ||
| + | |||
| + | Denote <math>F = AL \cap BD, E = AN \cap A'D \implies \ell = EF,</math> | ||
| + | |||
| + | <math>E' = AM \cap BD, F' = A'B \cap AK \implies \ell' = E'F'.</math> | ||
| + | <cmath>A'K = BL, \angle BA'B' = \angle KA'F' = \angle CBD = \angle LBF, \angle AKA' = \angle F'KA' =</cmath> | ||
| + | <cmath>= \angle ALB = \angle FLB \implies \triangle A'KF' = \triangle BLF \implies A'F' = BF.</cmath> | ||
| + | Similarly, <math>A'E = DE'.</math> | ||
| + | <math>A'B = BD = DA' \implies A'BD</math> is the regular triangle. | ||
| + | |||
| + | Denote <math>r</math> the rotation of the plane <math>A'BD</math> around the center of <math>\triangle A'BD</math> by an angle of <math>60^\circ</math> which maps point <math>A'</math> into point <math>B.</math> | ||
| + | |||
| + | The transforming <math>r</math> maps point <math>F'</math> into point <math>F,</math> point <math>E'</math> into point <math>E,</math> that is, line <math>\ell' = E'F'</math> maps into line <math>\ell.</math> | ||
| + | |||
| + | The angle between these lines is <math>60^\circ.</math> | ||
| + | |||
| + | Answer: <math>60^\circ.</math> | ||
| + | |||
| + | '''vladimir.shelomovskii@gmail.com, vvsss''' | ||
Latest revision as of 07:59, 30 July 2024
DVI is an exam in mathematics at the Moscow State University named after M.V. Lomonosov. The first four problems have a standard level. Problem 5 is advanced level of geometry. Problem 6 is an advanced level equation or inequality. Problem 7 is advanced level of stereometry.
Below are the most difficult problems of this exam in recent years. The headings indicate the year when the problem was used, the variant option of the exam, and the number of the problem.
Contents
[hide]- 1 2011 Problem 8
- 2 2012 Problem 8
- 3 2014 1 Problem 6
- 4 2014 1 Problem 8
- 5 2015 1 Problem 7
- 6 2016 2 Problem 7
- 7 2016 2 Problem 8
- 8 2020 var 201 problem 6
- 9 2020 var 202 problem 6
- 10 2020 var 203 problem 6
- 11 2020 var 204 problem 6
- 12 2020 var 205 problem 6
- 13 2020 var 206 problem 6
- 14 2021 var 215 problem 7
- 15 2022 var 221 problem 7
- 16 2022 var 222 problem 7
- 17 2022 var 222 problem 6
- 18 2022 var 224 problem 6
- 19 2023 var 231 problem 6
- 20 2023 var 231 EM problem 6
- 21 2023 var 232 problem 6
- 22 2023 var 233 problem 6
- 23 2024 Problem 18 (EGE)
- 24 2024 Test problem 7
- 25 2024 var 241 Problem 2
- 26 2024 var 242 Problem 7
- 27 2024 var 243 Problem 6
- 28 2024 var 244 Problem 7
- 29 2024 var 245 Problem 6
- 30 2024 var 246 Problem 5
- 31 2024 var 246 Problem 6
- 32 2024 var 246 Problem 7
- 33 2024 var 247 Problem 6
- 34 2024 var 247 Problem 7
2011 Problem 8
Solve the system of equations
![]() Standard Solution
Standard Solution
![]() Denote
Denote
![]() We get
We get ![]() First equation define inner points of the circle with radius
First equation define inner points of the circle with radius ![]() and the circle.
The distance from the straight line to the origin of the coordinate system
and the circle.
The distance from the straight line to the origin of the coordinate system ![]() is
is
![]() so the system of the equations define the only tangent point of the circle and the line.
so the system of the equations define the only tangent point of the circle and the line.
![]() Short Solution
Short Solution
![]()
![]()
2012 Problem 8
Let the tetrahedron ![]() be given.
be given.
A right circular cylinder is located so that the circle of its upper base touches each of the faces which contains vertex ![]()
The circle of the lower base lies in the ![]() plane and touches straight lines
plane and touches straight lines ![]() and
and ![]()
Find the height ![]() of the cylinder.
of the cylinder.
Solution
Denote ![]() the midpoint
the midpoint ![]() Plane
Plane ![]() is the bisector plane of segment
is the bisector plane of segment ![]()
![]()
The inradius of ![]() equal to
equal to ![]() distance from incenter
distance from incenter ![]() to vertex
to vertex ![]() is
is ![]()
Denote ![]() the foot from
the foot from ![]() to
to ![]()
Denote ![]() the crosssection of
the crosssection of ![]() by plane of the upper base of cylinder,
by plane of the upper base of cylinder, ![]() is the incenter
is the incenter ![]() is the point of tangency incircle of
is the point of tangency incircle of ![]() and
and ![]()
Denote ![]() and
and ![]() the foots from
the foots from ![]() and
and ![]() to
to ![]() Denote the radius
Denote the radius ![]()
The circle of the lower base inscribed in angle equal to ![]() so
so
![]()
![]() Projection from the point
Projection from the point ![]() maps
maps ![]() onto
onto ![]()
![]()
![]()
![]()
Answer: ![]()
2014 1 Problem 6
Find all pares of real numbers ![]() satisfying the system of equations
satisfying the system of equations
![]() Solution
Solution
Denote ![]()
![]() Denote
Denote ![]()
![]()
![]() is the solution. Let
is the solution. Let
![]() If
If ![]() then
then ![]() if
if ![]() then
then ![]() therefore
therefore ![]() is the single root.
is the single root.
2014 1 Problem 8
Let ![]()
Find ![]() and
and ![]()
Solution
![]()
![]()
![]() where
where ![]()
![]()
![]()
![]()
Answer:![]()
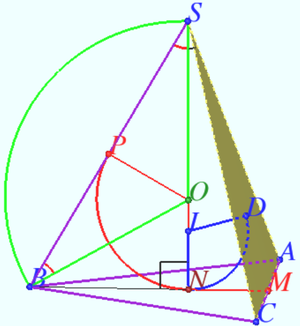
2015 1 Problem 7
A sphere is inscribed in a regular triangular prism with bases ![]() Find its radius if the distance between straight lines
Find its radius if the distance between straight lines ![]() and
and ![]() is equal to
is equal to ![]() where
where ![]() and
and ![]() are points lying on
are points lying on ![]() and
and ![]() , respectively, and
, respectively, and ![]()
Solution
The distance from the center of the sphere to the centers of the prism faces is equal to ![]() so
so ![]()
In order to find the distance ![]() between the lines
between the lines ![]() and
and ![]() , one can find the length of two perpendiculars
, one can find the length of two perpendiculars ![]() and
and ![]() to the line
to the line ![]() that are perpendicular to each other. Then
that are perpendicular to each other. Then
![]() since, when viewed along a straight line
since, when viewed along a straight line ![]() , the segment
, the segment ![]() is the altitude of a right triangle with legs
is the altitude of a right triangle with legs ![]() and
and ![]()
The plane ![]() containe the straight line
containe the straight line ![]() The straight line
The straight line ![]() crossed
crossed ![]() at the point
at the point ![]()
![]() In a right triangle
In a right triangle ![]()
![]()
![]() is the height falling on the hypotenuse,
is the height falling on the hypotenuse, ![]()
Let ![]() be the projection of
be the projection of ![]() onto plane
onto plane ![]()
Therefore ![]() is the projection of
is the projection of ![]() onto plane
onto plane ![]() at the point
at the point ![]()
![]()
![]()
![]()
![]() Answer:
Answer:![]()
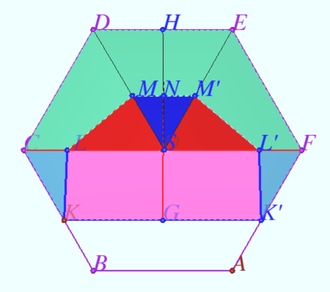
2016 2 Problem 7
Let the base of the regular pyramid with vertex ![]() be the hexagon
be the hexagon ![]() with side
with side ![]() The plane
The plane ![]() is parallel to the edge
is parallel to the edge ![]() , perpendicular to the plane
, perpendicular to the plane ![]() and intersects the edge
and intersects the edge ![]() at point
at point ![]() so that
so that ![]() The lines along which
The lines along which ![]() intersects the
intersects the ![]() plane and the base plane are perpendicular.
plane and the base plane are perpendicular.
Find the area of the triangle cut off by the plane ![]() from the face
from the face ![]()
Solution
Denote ![]()
![]() are the midpoints of
are the midpoints of ![]() respectively.
respectively.
Plane ![]() is the plane symmetry of pyramid,
is the plane symmetry of pyramid, ![]()
By condition ![]() so exist point
so exist point ![]()
![]() is the line along which
is the line along which ![]() intersects the
intersects the ![]() plane,
plane, ![]() is the line along which
is the line along which ![]() intersects the base plane, so
intersects the base plane, so ![]()
We use the top wiew and get
![]()
![]()
![]() Denote
Denote ![]() and use the side wiew.
and use the side wiew.
![]()
![]() Triangle
Triangle ![]() is the regular triangle with side
is the regular triangle with side ![]() , so
, so
![]()
![]()
![]() Answer: 8.
Answer: 8.
2016 2 Problem 8
Find the smallest value of the expression
![]() Solution
Solution
![]()
![]()
![]() Denote
Denote ![]()
![]()
![]() The shortest length of a broken line
The shortest length of a broken line ![]() with fixed ends is equal to the distance between points
with fixed ends is equal to the distance between points ![]() and
and ![]() which is
which is ![]() and is achieved if points
and is achieved if points ![]() and
and ![]() are collinear.
are collinear.
![]()
![]()
![]()
![]()
![]() Answer:
Answer:![]()
2020 var 201 problem 6
Let a triangular prism ![]() with a base
with a base ![]() be given,
be given, ![]() Find the ratio in which the plane
Find the ratio in which the plane ![]() divides the segment
divides the segment ![]() if
if ![]()
![]()
Solution
Let ![]() be the parallel projections of
be the parallel projections of ![]() on the plane
on the plane ![]()
![]()
We use and get
![]()
![]() Let
Let ![]()
Similarly ![]()
Answer: ![]()
2020 var 202 problem 6
Let a tetrahedron ![]() be given,
be given, ![]() Find the cosine of the angle
Find the cosine of the angle ![]() between the edges
between the edges ![]() and
and ![]()
Solution
Let us describe a parallelepiped ![]() around a given tetrahedron
around a given tetrahedron ![]()
![]() and
and ![]() are equal rectangles.
are equal rectangles.
![]() and
and ![]() are equal rectangles.
are equal rectangles.
Denote ![]()
![]()
![]()
![]()
![]() Answer:
Answer: ![]()
2020 var 203 problem 6
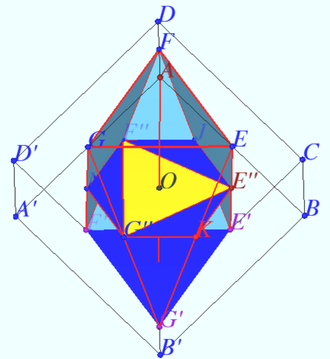
Let a cube ![]() with the base
with the base ![]() and side edges
and side edges ![]() be given. Find the volume of a polyhedron whose vertices are the midpoints of the edges
be given. Find the volume of a polyhedron whose vertices are the midpoints of the edges ![]()
Solution
Denote the vertices of polyhedron ![]() Triangles
Triangles ![]() and
and ![]() are equilateral triangles with sides
are equilateral triangles with sides ![]() and areas
and areas ![]()
This triangles lies in parallel planes, which are normal to cube diagonal ![]() The distance
The distance ![]() between this planes is
between this planes is ![]() So the volume of the regular prism with base
So the volume of the regular prism with base ![]() and height
and height ![]() is
is
![]()
Let the area ![]() be the quadratic function of
be the quadratic function of ![]() Let
Let
![]()
![]()
![]() Suppose, we move point
Suppose, we move point ![]() along axis
along axis ![]() and cross the solid by plane contains
and cross the solid by plane contains ![]() and normal to axis. Distance from
and normal to axis. Distance from ![]() to each crosspoint this plane with the edge change proportionally position
to each crosspoint this plane with the edge change proportionally position ![]() along axes, so the area is quadratic function from
along axes, so the area is quadratic function from ![]() position.
position.
![]()
![]()
Answer: ![]()
2020 var 204 problem 6
Let a regular triangular pyramid be given. The circumcenter of the sphere ![]() is equidistant from the edge and from the plane of the base of the pyramid. Find the radius of the sphere inscribed in this pyramid if the length of the edge of its base is
is equidistant from the edge and from the plane of the base of the pyramid. Find the radius of the sphere inscribed in this pyramid if the length of the edge of its base is ![]()
Solution
![]()
![]()
![]()
![]()
![]()
![]() Answer:
Answer: ![]()
2020 var 205 problem 6
Let the quadrangular pyramid ![]() with the base parallelogram
with the base parallelogram ![]() be given.
be given.
Point ![]() Point
Point ![]()
Find the ratio in which the plane ![]() divides the volume of the pyramid.
divides the volume of the pyramid.
Solution
Let plane ![]() cross edge
cross edge ![]() at point
at point ![]() We make the central projection from point
We make the central projection from point ![]() The images of points
The images of points ![]() are
are ![]() respectively.
The image of
respectively.
The image of ![]() is the crosspoint of
is the crosspoint of ![]() and
and ![]() So lines
So lines ![]() and
and ![]() are crossed at point
are crossed at point ![]()
![]()
![]() Let’s compare volumes of some tetrachedrons, denote the volume of
Let’s compare volumes of some tetrachedrons, denote the volume of ![]() as
as ![]()
![]()
![]()
![]()
![]() Answer: 1 : 6.
Answer: 1 : 6.
2020 var 206 problem 6
Given a cube ![]() with the base
with the base ![]() and side edges
and side edges ![]() Find the distance between the line passing through the midpoints of the edges
Find the distance between the line passing through the midpoints of the edges ![]() and
and ![]() and the line passing through the midpoints of the edges
and the line passing through the midpoints of the edges ![]() and
and ![]()
Solution
Let points ![]() be the midpoints of
be the midpoints of ![]() respectively. We need to prove that planes
respectively. We need to prove that planes ![]() and
and ![]() are parallel, perpendicular to
are parallel, perpendicular to ![]() Therefore,
Therefore, ![]()
Point ![]() is the midpoint
is the midpoint ![]()
![]()
![]() For proof we can use one of the following methods:
For proof we can use one of the following methods:
1. Vectors: ![]()
![]() Scalar product
Scalar product ![]() Similarly,
Similarly, ![]()
2. ![]()
3. Rotating the cube around its axis ![]() we find that the point
we find that the point ![]() move to
move to ![]() , then to
, then to ![]() then to
then to ![]()
Answer: ![]()
2021 var 215 problem 7
The sphere touches all edges of the tetrahedron ![]() It is known that the products of the lengths of crossing edges are equal. It is also known that
It is known that the products of the lengths of crossing edges are equal. It is also known that ![]() Find
Find ![]()
Solution
The tangent segments from the common point to the sphere are equal.
Let us denote the segments from the vertex ![]() to the sphere by
to the sphere by ![]()
Similarly, we define ![]()
![]()
![]()
![]()
![]() If
If ![]() then
then ![]()
If ![]()
The tetrahedron ![]() is a regular pyramid with a regular triangle with side
is a regular pyramid with a regular triangle with side ![]() at the base and side edges equal to
at the base and side edges equal to ![]()
Answer: 3.
2022 var 221 problem 7
The volume of a triangular prism ![]() with base
with base ![]() and side edges
and side edges ![]() is equal to
is equal to ![]() Find the volume of the tetrahedron
Find the volume of the tetrahedron ![]() where
where ![]() is the centroid of the face
is the centroid of the face ![]() is the point of intersection of the medians of
is the point of intersection of the medians of ![]() is the midpoint of the edge
is the midpoint of the edge ![]() and
and ![]() is the midpoint of the edge
is the midpoint of the edge ![]()
Solution
Let us consider the uniform triangular prism ![]() Let
Let ![]() be the midpoint of
be the midpoint of ![]() be the midpoint of
be the midpoint of ![]() be the midpoint of
be the midpoint of ![]() be the midpoint of
be the midpoint of ![]()
The area ![]() of
of ![]() in the sum with the areas of triangles
in the sum with the areas of triangles ![]() is half the area of rectangle
is half the area of rectangle ![]() so
so
![]()
![]() Denote the distance between these lines
Denote the distance between these lines ![]() The volume of the tetrahedron is
The volume of the tetrahedron is ![]()
![]() The volume of the prism is
The volume of the prism is ![]()
![]()
An arbitrary prism is obtained from a regular one as a result of an affine transformation.
All points on the tetrahedron are defined affinely, which means that the volume ratio will be preserved.
Answer: 5.
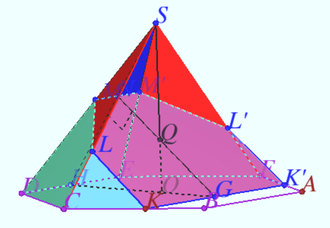
2022 var 222 problem 7
A sphere of diameter ![]() is inscribed in a pyramid at the base of which lies a rhombus with an acute angle
is inscribed in a pyramid at the base of which lies a rhombus with an acute angle ![]() and side
and side ![]() Find the angle
Find the angle ![]() if it is known that all lateral faces of the pyramid are inclined to plane of its base at an angle of
if it is known that all lateral faces of the pyramid are inclined to plane of its base at an angle of ![]()
Solution 1
Denote rhombus ![]() is the vertex of a pyramid
is the vertex of a pyramid ![]() is the center of the sphere,
is the center of the sphere, ![]() is the tangent point of
is the tangent point of ![]() and sphere,
and sphere, ![]()
![]()
![]()
![]()
![]() Solution 2
Solution 2
The area of the rhombus ![]()
The area of the lateral surface is ![]()
![]()
![]() Answer:
Answer:![]()
2022 var 222 problem 6
Find all possible values of the product ![]() if it is known that
if it is known that ![]() and it is true
and it is true
![]()
Solution
Let ![]() then for each
then for each ![]() equation is true,
equation is true, ![]() Let
Let ![]()
![]()
![]()
![]()
![]()
![]() no solution.
no solution.
Answer:![]()
2022 var 224 problem 6
Find all triples of real numbers ![]() in the interval
in the interval ![]() satisfying the system of equations
satisfying the system of equations
![]()
Solution
Denote ![]()
![]()
![]() Similarly,
Similarly, ![]()
 Therefore
Therefore
![]()
![]() Answer:
Answer:![]()
2023 var 231 problem 6
Let positive numbers ![]() be such that
be such that ![]()
Find the maximum value of ![]()
Solution
![]()
![]() Similarly
Similarly
![]() Adding this equations, we get:
Adding this equations, we get:
![]() If
If ![]() then
then ![]()
Answer:![]()
Explanation for students
For the function under study ![]() it is required to find the majorizing function
it is required to find the majorizing function ![]() This function must be a linear combination of the given function
This function must be a linear combination of the given function ![]() and a constant,
and a constant, ![]()
At the supposed extremum point ![]() the functions and their derivatives must coincide
the functions and their derivatives must coincide ![]()
![]()
![]()
2023 var 231 EM problem 6
![]() Find the maximum value
Find the maximum value ![]() and all argument values
and all argument values ![]() such that
such that ![]() .
.
Solution
![]()
![]()
![]() because
because ![]() and signs of
and signs of ![]() and
and ![]() are different, so
are different, so ![]() Therefore
Therefore ![]()
2023 var 232 problem 6
Let positive numbers ![]() be such that
be such that ![]() Find the maximum value of
Find the maximum value of ![]()
Solution
![]()
![]()
![]()
![]() It is clear that
It is clear that ![]() and
and ![]() Denote
Denote ![]() So
So ![]()
![]() If
If ![]() then
then ![]()
Answer:![]()
2023 var 233 problem 6
Let positive numbers ![]() be such that
be such that ![]()
Find the maximum value of ![]()
Solution
Let ![]() Then
Then
![]()
![]()
Equality is achieved if ![]()
Answer: ![]()
2024 Problem 18 (EGE)
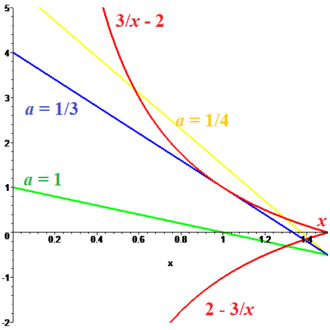
Find those values of the parameter a for which the system of equations has exactly one solution:
![]() Solution
Solution
1. Special case ![]() exactly one solution.
exactly one solution.
2. ![]()
3. We solve the first equation with respect ![]() and get
and get ![]()
This solution is shown in the diagram by red curve.
We solve the second equation with respect ![]() and get
and get
![]() This solution is shown in the diagram by segments which connect point
This solution is shown in the diagram by segments which connect point ![]() with axis
with axis ![]()
Each solution of the system is shown by the point of crosspoint red curve with segment.
If ![]() then segment (colored by blue) is tangent to red curve (discriminant is zero), so we have two solutions (1,1) and
then segment (colored by blue) is tangent to red curve (discriminant is zero), so we have two solutions (1,1) and ![]()
If ![]() we get three solutions (colored by yellow).
we get three solutions (colored by yellow).
In other cases the system has exactly one solution.
Answer: ![]()
2024 Test problem 7
Find all values of the parameter a for which there is at least one solution to the inequality ![]() on the interval
on the interval ![]()
Solution
![]() where
where ![]() where
where ![]()
The equation ![]() has solutions
has solutions ![]() and
and ![]()
![]()
![]() if
if ![]() so given inequality has the solution
so given inequality has the solution ![]() for these
for these ![]()
![]()
![]() if
if ![]() so given inequality has the solution
so given inequality has the solution ![]() for these
for these ![]()
![]() no solution of the given inequality.
no solution of the given inequality.
![]() no solution of the inequality if
no solution of the inequality if ![]()
![]() If
If ![]() no solution of the inequality.
no solution of the inequality.
![]() If
If ![]() no solution of the given inequality.
no solution of the given inequality.
![]() If
If ![]() no solution of the given inequality.
no solution of the given inequality.
2024 var 241 Problem 2
The natural numbers ![]() form a strictly increasing arithmetic progression. Find all possible values of
form a strictly increasing arithmetic progression. Find all possible values of ![]() if it is known that
if it is known that ![]() is odd,
is odd, ![]() and
and ![]()
Solution
![]() is odd, so
is odd, so ![]()
Let ![]() the common difference may be
the common difference may be ![]() increasing arithmetic progression exist.
increasing arithmetic progression exist.
Let ![]() the common difference may be
the common difference may be ![]() increasing arithmetic progression exist.
increasing arithmetic progression exist.
Let ![]() can not be the natural number.
can not be the natural number.
Answer: ![]()
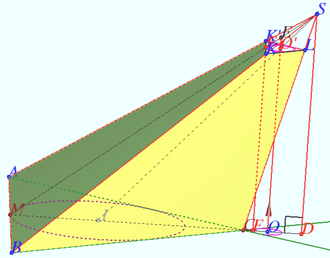
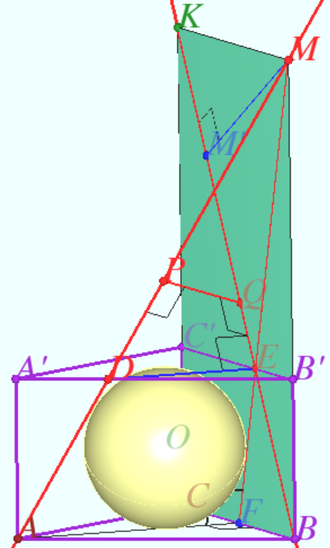
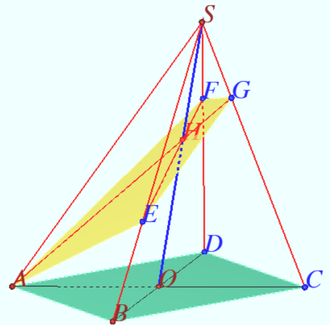
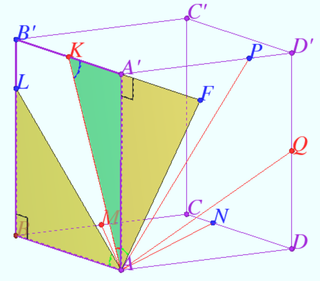
2024 var 242 Problem 7
The base of the pyramid is the trapezoid ![]()
A sphere of radius ![]() touches the plane of the base of the pyramid and the planes of its lateral faces
touches the plane of the base of the pyramid and the planes of its lateral faces ![]() and
and ![]() at points
at points ![]() and
and ![]() respectively.
respectively.
Find the ratio in which the volume of the pyramid is divided by the plane ![]() if the face
if the face ![]() is perpendicular to the plane
is perpendicular to the plane ![]() and the height of the pyramid is
and the height of the pyramid is ![]()
Solution
A sketch of the given pyramid is shown in the diagram. The planes ![]() and
and ![]() intersect along the straight line
intersect along the straight line ![]() that is, the planes
that is, the planes ![]() form the lateral surface of a prism into which a sphere with center at point
form the lateral surface of a prism into which a sphere with center at point ![]() is inscribed.
is inscribed.
The plane ![]() containing the point
containing the point ![]() and perpendicular to
and perpendicular to ![]() contains points
contains points ![]() and
and ![]() Plane
Plane ![]() intersects parallel lines
intersects parallel lines ![]() and
and ![]() at points
at points ![]() and
and ![]() respectively.
respectively.
Let ![]() be the line parallel to
be the line parallel to ![]() The plane
The plane ![]() cuts off the pyramid
cuts off the pyramid ![]() with volume
with volume ![]() from the pyramid
from the pyramid ![]() with volume
with volume ![]()
![]() and equal to the distance from
and equal to the distance from ![]() to
to ![]() and equal to the distance between
and equal to the distance between ![]() and
and ![]()
![]() Consider a right triangle
Consider a right triangle ![]() is the area of
is the area of ![]() into which a circle
into which a circle ![]() with radius
with radius ![]() is inscribed.
is inscribed.
![]()
![]() We are looking for
We are looking for ![]() Let
Let ![]() be the distance from
be the distance from ![]() to the plane
to the plane ![]()
![]()
![]() Answer:
Answer: ![]()
2024 var 243 Problem 6
Solve the system of equations in the positive ![]()
![]()
Solution (after Natalia Zakharova)
![]()
![]()
![]()
![]()
![]()
![]() Answer:
Answer: ![]()
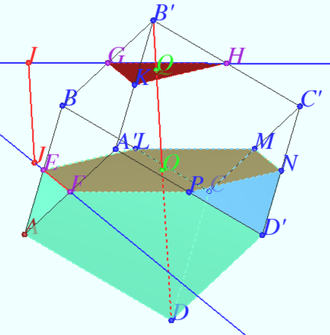
2024 var 244 Problem 7
Let ![]() be the cube,
be the cube, ![]() . Let
. Let ![]()
![]()
![]()
![]()
Find the ratio in which the plane ![]() divides the volume of the cube.
divides the volume of the cube.
Solution
1. Let ![]() lie on the ray
lie on the ray ![]()
![]()
![]()
![]()
![]() So
So ![]()
Similarly, ![]() is the midpoint
is the midpoint ![]() is the midpoint
is the midpoint ![]()
2. ![]()
regular pyramids are equal ![]() So
So ![]() (midpoint
(midpoint ![]() ) lies in plane
) lies in plane ![]()
Let ![]() be the midpoint
be the midpoint ![]() symmetric to
symmetric to ![]() with respect
with respect ![]() so
so ![]()
Similarly ![]() where
where ![]() midpoint
midpoint ![]() the midpoint
the midpoint ![]()
For each point on the edges of the solid forming a part of the cube cut off by a plane ![]() from the side of vertex
from the side of vertex ![]() one can find a point symmetrical relative to the center of the cube
one can find a point symmetrical relative to the center of the cube ![]() on the edges of the solid forming another part of the cube.
on the edges of the solid forming another part of the cube.
It means that these parts are congruent and the plane ![]() divides the cube in half.
divides the cube in half.
Answer: ![]()
2024 var 245 Problem 6
Let ![]() and
and ![]() be the positive real numbers such that
be the positive real numbers such that ![]() Find the minimal value of
Find the minimal value of ![]()
Solution
![]() For the last transform we use unequality between the harmonic mean and the arithmetic mean for three numbers.
Therefore
For the last transform we use unequality between the harmonic mean and the arithmetic mean for three numbers.
Therefore
![]() Equality we get if
Equality we get if ![]()
Answer: ![]()
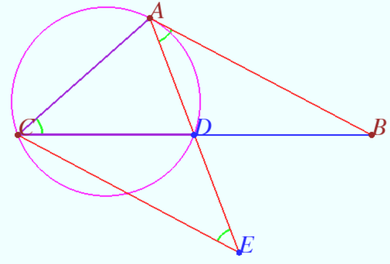
2024 var 246 Problem 5
Let ![]() be given,
be given, ![]() Point
Point ![]() is located on side
is located on side ![]() so that
so that ![]() the circle
the circle ![]() touches
touches ![]() at point
at point ![]()
Point ![]() is located on ray
is located on ray ![]() so that
so that ![]()
Find the ratio of ![]()
Solution
![]() Triangles
Triangles ![]() and
and ![]() are isosceles with a common angle
are isosceles with a common angle ![]()
![]()
![]() is the parallelogram
is the parallelogram ![]()
![]() Answer:
Answer: ![]()
2024 var 246 Problem 6
![]() where
where ![]() and
and ![]() are real numbers.
are real numbers.
Find ![]() if
if ![]() where
where
1) ![]() are real numbers,
are real numbers,
2) ![]() are positive numbers.
are positive numbers.
Solution (after Natalia Zakharova)
1) ![]() has four real roots
has four real roots
![]() if
if ![]()
![]()
![]() can take any real values. There are positive and negative roots
can take any real values. There are positive and negative roots ![]()
2) ![]()
![]() Answer:
Answer: ![]() any real number,
any real number, ![]()
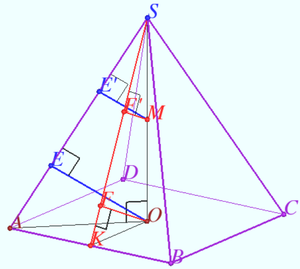
2024 var 246 Problem 7
The distance from the midpoint ![]() of the height
of the height ![]() of a regular quadrangular pyramid
of a regular quadrangular pyramid ![]() to the lateral face is
to the lateral face is ![]() and to the lateral edge is
and to the lateral edge is ![]()
Find the volume of the pyramid.
Solution
Let ![]() be the midpoint
be the midpoint ![]()
![]() Denote
Denote ![]()
Let us express the heights of right triangles through their legs:
![]()
![]()
![]()
![]() Answer:
Answer: ![]()
2024 var 247 Problem 6
Real numbers ![]() and
and ![]() satisfy the system of equations
satisfy the system of equations
![]() Find the largest possible value of
Find the largest possible value of ![]()
Solution
In coordinates ![]() and
and ![]() the first equation defines the plane
the first equation defines the plane ![]() the second - a sphere with the center at the origin. They are shown in the diagram.
the second - a sphere with the center at the origin. They are shown in the diagram.
The solution of the given system (if it exists) is a circle symmetrical with respect to the plane ![]() This plane intersects the plane of the first equation along the line
This plane intersects the plane of the first equation along the line ![]() on which the points of maximum (E) and minimum (D) of the values of
on which the points of maximum (E) and minimum (D) of the values of ![]() are located.
are located.
At these points the system takes the form
![]()
![]() These system has two solutions
These system has two solutions ![]() so solution of the given system exist.
so solution of the given system exist.
Answer: ![]()
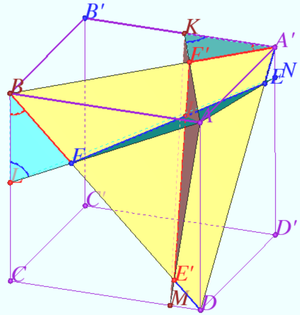
2024 var 247 Problem 7
Let the cube ![]() be given. Let points
be given. Let points ![]() and
and ![]() be given,
be given, ![]()
Let the plane ![]() cross the plane
cross the plane ![]() by the line
by the line ![]() and cross the plane
and cross the plane ![]() by line
by line ![]()
Find the angle between ![]() and
and ![]()
Solution
Denote ![]()
![]()
![]()
![]() Similarly,
Similarly, ![]()
![]() is the regular triangle.
is the regular triangle.
Denote ![]() the rotation of the plane
the rotation of the plane ![]() around the center of
around the center of ![]() by an angle of
by an angle of ![]() which maps point
which maps point ![]() into point
into point ![]()
The transforming ![]() maps point
maps point ![]() into point
into point ![]() point
point ![]() into point
into point ![]() that is, line
that is, line ![]() maps into line
maps into line ![]()
The angle between these lines is ![]()
Answer: ![]()
vladimir.shelomovskii@gmail.com, vvsss