Difference between revisions of "2006 AMC 12A Problems/Problem 13"
I_like_pie (talk | contribs) |
m (→Problem) |
||
| Line 5: | Line 5: | ||
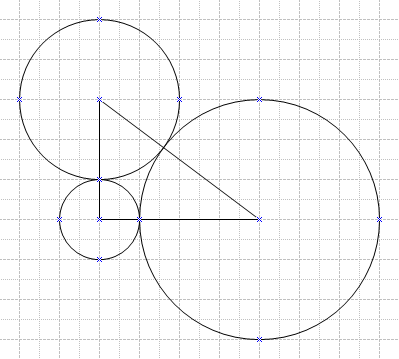
The vertices of a <math>3-4-5</math> right triangle are the centers of three mutually externally tangent circles, as shown. What is the sum of the areas of the three circles? | The vertices of a <math>3-4-5</math> right triangle are the centers of three mutually externally tangent circles, as shown. What is the sum of the areas of the three circles? | ||
| − | <math> \mathrm{(A) \ } 12\pi\qquad \mathrm{(B) \ } \frac{25\pi}{2}\qquad \mathrm{(C) \ } 13\pi\qquad \mathrm{(D) \ } \frac{27\pi}{2} | + | <math> \mathrm{(A) \ } 12\pi\qquad \mathrm{(B) \ } \frac{25\pi}{2}\qquad \mathrm{(C) \ } 13\pi\qquad \mathrm{(D) \ } \frac{27\pi}{2}\qquad\mathrm{(E) \ } 14\pi</math> |
== Solution == | == Solution == | ||
Revision as of 18:06, 4 November 2006
Problem
The vertices of a 
right triangle are the centers of three mutually externally tangent circles, as shown. What is the sum of the areas of the three circles?
Solution
This problem needs a solution. If you have a solution for it, please help us out by adding it.
