Difference between revisions of "Symmedians, Lemoine point"
(→Symmedian and tangents) |
(→Symmedian and tangents) |
||
| Line 38: | Line 38: | ||
'''vladimir.shelomovskii@gmail.com, vvsss''' | '''vladimir.shelomovskii@gmail.com, vvsss''' | ||
==Symmedian and tangents== | ==Symmedian and tangents== | ||
| − | [[File:Tangents and symmedian.png| | + | [[File:Tangents and symmedian.png|220px|right]] |
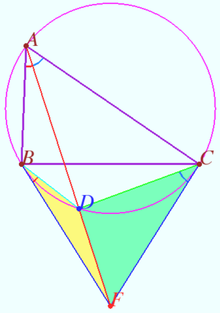
| − | Let <math>\triangle ABC</math> and it’s circumcircle <math>\Omega</math> be given. Tangents to <math>\Omega</math> at points <math>B</math> and <math>C</math> intersect at point <math>F.</math> | + | Let <math>\triangle ABC</math> and it’s circumcircle <math>\Omega</math> be given. |
| + | |||
| + | Tangents to <math>\Omega</math> at points <math>B</math> and <math>C</math> intersect at point <math>F.</math> | ||
| + | |||
Prove that <math>AF</math> is <math>A-</math> symmedian of <math>\triangle ABC.</math> | Prove that <math>AF</math> is <math>A-</math> symmedian of <math>\triangle ABC.</math> | ||
| Line 48: | Line 51: | ||
<cmath>\triangle FDC \sim \triangle FCA \implies \frac {CD}{AC} = \frac{DF}{CF}.</cmath> | <cmath>\triangle FDC \sim \triangle FCA \implies \frac {CD}{AC} = \frac{DF}{CF}.</cmath> | ||
<math>BF = CF \implies \frac {BD}{CD} = \frac{AB}{AC} \implies AD</math> is <math>A-</math> symmedian of <math>\triangle ABC.</math> | <math>BF = CF \implies \frac {BD}{CD} = \frac{AB}{AC} \implies AD</math> is <math>A-</math> symmedian of <math>\triangle ABC.</math> | ||
| + | |||
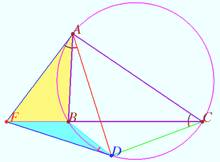
| + | <i><b>Corollary</b></i> | ||
| + | |||
| + | [[File:Tangents to symmedian.png|220px|right]] | ||
| + | Let <math>\triangle ABC</math> and it’s circumcircle <math>\Omega</math> be given. | ||
| + | |||
| + | Let tangent to <math>\Omega</math> at points <math>A</math> intersect line <math>BC</math> at point <math>F.</math> | ||
| + | |||
| + | Let <math>FD</math> be the tangent to <math>\Omega</math> different from <math>FA.</math> | ||
| + | |||
| + | Then <math>AD</math> is <math>A-</math> symmedian of <math>\triangle ABC.</math> | ||
'''vladimir.shelomovskii@gmail.com, vvsss''' | '''vladimir.shelomovskii@gmail.com, vvsss''' | ||
Revision as of 13:55, 14 July 2024
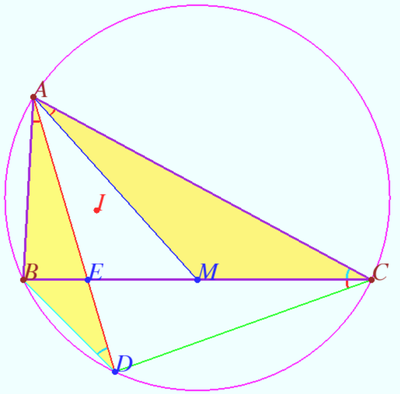
The reflecting of the median over the corresponding angle bisector is the symmedian. The angle formed by the symmedian and the angle bisector has the same measure as the angle between the median and the angle bisector, but it is on the other side of the angle bisector. The symmedian ![]() is isogonally conjugate to the median
is isogonally conjugate to the median ![]()
There are three symmedians. They are meet at a triangle center called the Lemoine point.
Proportions
Let ![]() be given.
be given.
Let ![]() be the median,
be the median, ![]()
Prove that iff ![]() is the symmedian than
is the symmedian than ![]()
Proof
1. Let ![]() be the symmedian. So
be the symmedian. So ![]()
![]()
![]() Similarly
Similarly ![]()
![]()
By applying the Law of Sines we get
![]()
![]() Similarly,
Similarly, ![]()
2. ![]()
As point ![]() moves along the fixed arc
moves along the fixed arc ![]() from
from ![]() to
to ![]() , the function
, the function ![]() monotonically increases from zero to infinity. This means that there is exactly one point at which the condition is satisfied. In this case, point
monotonically increases from zero to infinity. This means that there is exactly one point at which the condition is satisfied. In this case, point ![]() lies on the symmedian.
lies on the symmedian.
Similarly for point ![]()
Corollary
Let ![]() be the
be the ![]() symmedian of
symmedian of ![]()
Then ![]() is the
is the ![]() symmedian of
symmedian of ![]() is the
is the ![]() symmedian of
symmedian of ![]() is the
is the ![]() symmedian of
symmedian of ![]()
vladimir.shelomovskii@gmail.com, vvsss
Symmedian and tangents
Let ![]() and it’s circumcircle
and it’s circumcircle ![]() be given.
be given.
Tangents to ![]() at points
at points ![]() and
and ![]() intersect at point
intersect at point ![]()
Prove that ![]() is
is ![]() symmedian of
symmedian of ![]()
Proof
Denote ![]() WLOG,
WLOG, ![]()
![]()
![]()
![]() is
is ![]() symmedian of
symmedian of ![]()
Corollary
Let ![]() and it’s circumcircle
and it’s circumcircle ![]() be given.
be given.
Let tangent to ![]() at points
at points ![]() intersect line
intersect line ![]() at point
at point ![]()
Let ![]() be the tangent to
be the tangent to ![]() different from
different from ![]()
Then ![]() is
is ![]() symmedian of
symmedian of ![]()
vladimir.shelomovskii@gmail.com, vvsss