Difference between revisions of "2006 AMC 10B Problems/Problem 6"
Ragnarok23 (talk | contribs) |
(Added problem and solution) |
||
| Line 1: | Line 1: | ||
== Problem == | == Problem == | ||
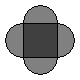
| + | A region is bounded by semicircular arcs constructed on the side of a square whose sides measure <math> \frac{2}{\pi} </math>, as shown. What is the perimeter of this region? | ||
| + | |||
| + | [[Image:2006amc10b06.gif]] | ||
| + | |||
| + | <math> \mathrm{(A) \ } \frac{4}{\pi}\qquad \mathrm{(B) \ } 2\qquad \mathrm{(C) \ } \frac{8}{\pi}\qquad \mathrm{(D) \ } 4\qquad \mathrm{(E) \ } \frac{16}{\pi} </math> | ||
| + | |||
== Solution == | == Solution == | ||
| + | Since the side of the square is the diameter of the semicircle, the radius of the semicircle is <math> \frac{1}{2}\cdot\frac{2}{\pi}=\frac{1}{\pi} </math> | ||
| + | |||
| + | Since the length of one of the semicircular arcs is half the circumference of the corresponding circle, the length of one arc is <math> \frac{1}{2}\cdot2\cdot\pi\cdot\frac{1}{\pi}=1</math> | ||
| + | |||
| + | Since the desired perimeter is made up of four of these arcs, the perimeter is <math>4\cdot1=4\Rightarrow D</math> | ||
| + | |||
== See Also == | == See Also == | ||
*[[2006 AMC 10B Problems]] | *[[2006 AMC 10B Problems]] | ||
Revision as of 19:37, 13 July 2006
Problem
A region is bounded by semicircular arcs constructed on the side of a square whose sides measure ![]() , as shown. What is the perimeter of this region?
, as shown. What is the perimeter of this region?
![]()
Solution
Since the side of the square is the diameter of the semicircle, the radius of the semicircle is ![]()
Since the length of one of the semicircular arcs is half the circumference of the corresponding circle, the length of one arc is ![]()
Since the desired perimeter is made up of four of these arcs, the perimeter is ![]()