Difference between revisions of "Mock AIME 2 2006-2007 Problems/Problem 15"
m |
m |
||
| Line 1: | Line 1: | ||
== Problem == | == Problem == | ||
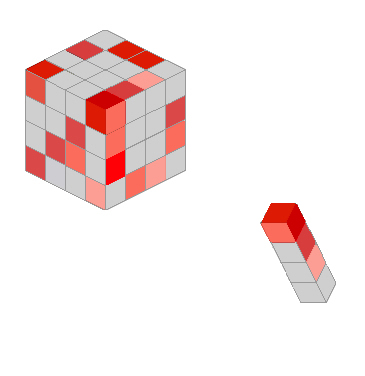
| − | A <math>\displaystyle 4\times4\times4</math> cube is composed of <math>\displaystyle 64</math> unit cubes. The faces of <math>\displaystyle 16</math> unit cubes are colored red. An arrangement of the cubes is <math>\mathfrak{Intriguing}</math> if there is exactly <math>\displaystyle 1</math> red unit cube in every <math>\displaystyle 1\times1\times4</math> rectangular box composed of <math>\displaystyle 4</math> unit cubes. Determine the number of <math>\mathfrak{Intriguing}</math> colorings. | + | A <math>\displaystyle 4\times4\times4</math> [[cube (geometry) | cube]] is composed of <math>\displaystyle 64</math> unit cubes. The faces of <math>\displaystyle 16</math> unit cubes are colored red. An arrangement of the cubes is <math>\mathfrak{Intriguing}</math> if there is exactly <math>\displaystyle 1</math> red unit cube in every <math>\displaystyle 1\times1\times4</math> rectangular box composed of <math>\displaystyle 4</math> unit cubes. Determine the number of <math>\mathfrak{Intriguing}</math> colorings. |
[[Image:CubeArt.jpg]] | [[Image:CubeArt.jpg]] | ||
Revision as of 11:29, 30 October 2006
Problem
A ![]() cube is composed of
cube is composed of ![]() unit cubes. The faces of
unit cubes. The faces of ![]() unit cubes are colored red. An arrangement of the cubes is
unit cubes are colored red. An arrangement of the cubes is ![]() if there is exactly
if there is exactly ![]() red unit cube in every
red unit cube in every ![]() rectangular box composed of
rectangular box composed of ![]() unit cubes. Determine the number of
unit cubes. Determine the number of ![]() colorings.
colorings.
Solution
This problem needs a solution. If you have a solution for it, please help us out by adding it.