Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "2011 AIME II Problems/Problem 8"

m (→‎Solution)
(→‎Solution)
Line 7: Line 7:
  
 
The twelve dots above represent the <math>12</math> roots of the equation <math>z^{12}-2^{36}=0</math>. If we write <math>z=a+bi</math>, then the real part of <math>z</math> is <math>a</math> and the real part of <math>iz</math> is <math>-b</math>. The blue dots represent those roots <math>z</math> for which the real part of <math>z</math> is greater than the real part of <math>iz</math>, and the red dots represent those roots <math>z</math> for which the real part of <math>iz</math> is greater than the real part of <math>z</math>. Now, the sum of the real parts of the blue dots is easily seen to be <math>8+16\cos\frac{\pi}{6}=8+8\sqrt{3}</math> and the negative of the sum of the imaginary parts of the red dots is easily seen to also be <math>8+8\sqrt{3}</math>. Hence our desired sum is <math>16+16\sqrt{3}=16+\sqrt{768}</math>, giving the answer <math>\boxed{784}</math>.
 
The twelve dots above represent the <math>12</math> roots of the equation <math>z^{12}-2^{36}=0</math>. If we write <math>z=a+bi</math>, then the real part of <math>z</math> is <math>a</math> and the real part of <math>iz</math> is <math>-b</math>. The blue dots represent those roots <math>z</math> for which the real part of <math>z</math> is greater than the real part of <math>iz</math>, and the red dots represent those roots <math>z</math> for which the real part of <math>iz</math> is greater than the real part of <math>z</math>. Now, the sum of the real parts of the blue dots is easily seen to be <math>8+16\cos\frac{\pi}{6}=8+8\sqrt{3}</math> and the negative of the sum of the imaginary parts of the red dots is easily seen to also be <math>8+8\sqrt{3}</math>. Hence our desired sum is <math>16+16\sqrt{3}=16+\sqrt{768}</math>, giving the answer <math>\boxed{784}</math>.
 +
 +
== Solution 2 ==
  
 
==See also==
 
==See also==

Revision as of 22:07, 11 December 2019

Problem

Let $z_1,z_2,z_3,\dots,z_{12}$ be the 12 zeroes of the polynomial $z^{12}-2^{36}$. For each $j$, let $w_j$ be one of $z_j$ or $i z_j$. Then the maximum possible value of the real part of $\displaystyle\sum_{j=1}^{12} w_j$ can be written as $m+\sqrt{n}$ where $m$ and $n$ are positive integers. Find $m+n$.

Solution

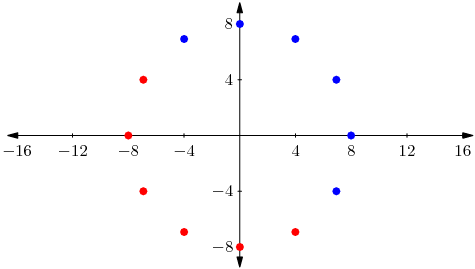
2011 AIME II -8.png

The twelve dots above represent the $12$ roots of the equation $z^{12}-2^{36}=0$. If we write $z=a+bi$, then the real part of $z$ is $a$ and the real part of $iz$ is $-b$. The blue dots represent those roots $z$ for which the real part of $z$ is greater than the real part of $iz$, and the red dots represent those roots $z$ for which the real part of $iz$ is greater than the real part of $z$. Now, the sum of the real parts of the blue dots is easily seen to be $8+16\cos\frac{\pi}{6}=8+8\sqrt{3}$ and the negative of the sum of the imaginary parts of the red dots is easily seen to also be $8+8\sqrt{3}$. Hence our desired sum is $16+16\sqrt{3}=16+\sqrt{768}$, giving the answer $\boxed{784}$.

Solution 2

See also

2011 AIME II (Problems • Answer Key • Resources)
Preceded by
Problem 7 		Followed by
Problem 9
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15
All AIME Problems and Solutions

The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. AMC logo.png

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=2011_AIME_II_Problems/Problem_8&oldid=112757"