Difference between revisions of "Spieker center"
(Created page with "The Spieker center is defined as the center of mass of the perimeter of the triangle. The Spieker center of a <math>\triangle ABC</math> is the center of gravity of a homogene...") |
|||
| Line 1: | Line 1: | ||
The Spieker center is defined as the center of mass of the perimeter of the triangle. The Spieker center of a <math>\triangle ABC</math> is the center of gravity of a homogeneous wire frame in the shape of <math>\triangle ABC.</math> The Spieker center is a triangle center and it is listed as the point <math>X_{10}.</math> | The Spieker center is defined as the center of mass of the perimeter of the triangle. The Spieker center of a <math>\triangle ABC</math> is the center of gravity of a homogeneous wire frame in the shape of <math>\triangle ABC.</math> The Spieker center is a triangle center and it is listed as the point <math>X_{10}.</math> | ||
| + | |||
| + | == Incenter of medial triangle== | ||
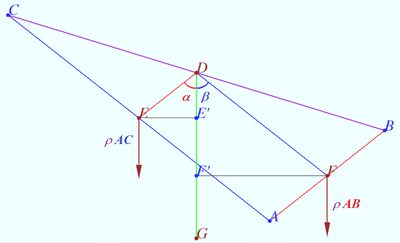
| + | [[File:Physical proof.png|400px|right]] | ||
| + | Prove that <math>X_{10}</math> is the incenter of the medial triangle <math>\triangle DEF</math> of a <math>\triangle ABC.</math> | ||
| + | |||
| + | <i><b>Proof</b></i> | ||
| + | |||
| + | Let's hang up the <math>\triangle ABC</math> in the middle of side <math>BC.</math> Side <math>BC</math> is balanced. | ||
| + | |||
| + | Let's replace side <math>AC</math> with point <math>E</math> (the center of mass of <math>AC,</math> the midpoint <math>AC).</math> Denote <math>\rho</math> the linear density of a homogeneous wire frame. | ||
| + | |||
| + | The mass of point <math>E</math> is equal to <math>\rho \cdot AC,</math> the shoulder of the gravity force is <math>EE' = ED \sin \alpha = \frac {AB \sin \alpha }{2}.</math> | ||
| + | |||
| + | The moment of this force is <math>g EE' \rho \cdot AC = \frac {g \rho\cdot AC \cdot AB}{2} \sin \alpha.</math> | ||
| + | |||
| + | Similarly the moment gravity force acting on AB is <math> \frac {g \rho\cdot AC \cdot AB}{2} \sin \beta.</math> | ||
| + | |||
| + | Therefore, equilibrium condition is <math>\alpha = \beta</math> and the center of gravity of a homogeneous wire frame <math>ABC</math> lies on each bisector of <math>\triangle DEF.</math> | ||
| + | |||
| + | This point is the incenter of the medial triangle <math>\triangle DEF.</math> | ||
| + | |||
| + | '''vladimir.shelomovskii@gmail.com, vvsss''' | ||
Revision as of 10:30, 7 August 2023
The Spieker center is defined as the center of mass of the perimeter of the triangle. The Spieker center of a ![]() is the center of gravity of a homogeneous wire frame in the shape of
is the center of gravity of a homogeneous wire frame in the shape of ![]() The Spieker center is a triangle center and it is listed as the point
The Spieker center is a triangle center and it is listed as the point ![]()
Incenter of medial triangle
Prove that ![]() is the incenter of the medial triangle
is the incenter of the medial triangle ![]() of a
of a ![]()
Proof
Let's hang up the ![]() in the middle of side
in the middle of side ![]() Side
Side ![]() is balanced.
is balanced.
Let's replace side ![]() with point
with point ![]() (the center of mass of
(the center of mass of ![]() the midpoint
the midpoint ![]() Denote
Denote ![]() the linear density of a homogeneous wire frame.
the linear density of a homogeneous wire frame.
The mass of point ![]() is equal to
is equal to ![]() the shoulder of the gravity force is
the shoulder of the gravity force is ![]()
The moment of this force is ![]()
Similarly the moment gravity force acting on AB is ![]()
Therefore, equilibrium condition is ![]() and the center of gravity of a homogeneous wire frame
and the center of gravity of a homogeneous wire frame ![]() lies on each bisector of
lies on each bisector of ![]()
This point is the incenter of the medial triangle ![]()
vladimir.shelomovskii@gmail.com, vvsss