Difference between revisions of "Bisector"

Revision as of 15:55, 10 December 2023

Division of bisector

Let a triangle $\triangle ABC, BC = a, AC = b, AB = c$ be given.

Let $AA', BB',$ and $CC'$ be the bisectors of $\triangle ABC.$

he segments $BB'$ and $A'C'$ meet at point $D.$ Find $\frac {BI}{BB'}, \frac {DA'}{DC'}, \frac {BD}{BB'}.$

Solution

$\frac {BA'}{CA'} = \frac {BA}{CA} = \frac {c}{b}, BA' + CA' = BC = a \implies BA' = \frac {a \cdot c}{b+c}.$

Similarly $BC' = \frac {a \cdot c}{a+b}, B'C = \frac {a \cdot b}{a+b}.$ $\frac {BI}{IB'} = \frac {a}{B'C} = \frac{a+c}{b} \implies \frac {BI}{BB'} = \frac {a+c}{a + b +c}.$

$\frac {DA'}{DC'} = \frac {BA'}{BC'} = \frac {a+ b}{b +c}.$

Denote $\angle ABC = 2 \beta.$ Bisector $BB' = 2 \frac {a \cdot c}{a + c} \cos \beta.$

Bisector $BD = 2 \frac {BC' \cdot BA'}{BC' + BA'} \cos \beta \implies$ $\frac {BD}{BB'} = \frac{a+c}{a+2b+c}.$ vladimir.shelomovskii@gmail.com, vvsss

Bisectors and tangent

Let a triangle $\triangle ABC (\angle BAC > \angle BCA)$ and it’s circumcircle $\Omega$ be given.

Let segments $BD, D \in AC$ and $BE, E \in AC,$ be the internal and external bisectors of $\triangle ABC.$ The tangent to $\Omega$ at $B$ meet $AC$ at point $M.$ Prove that

a) $EM = DM = BM,$

b) $\frac {1}{BM} = \frac {1}{AD} - \frac {1}{CD},$

c) $\frac {AD^2}{CD^2}=\frac {AM}{CM}.$

Proof

a) $\angle ABM = \angle ACB = \frac {\overset{\Large\frown} {AB}}{2} \implies \angle ADB = \angle CBD + \angle BCD = \angle ABD + \angle ABM = \angle MBD \implies BM = DM.$ $\angle DBE = 90^\circ \implies M$ is circumcenter $\triangle BDE \implies EM = MD.$

b) $\frac {AD}{DC} = \frac {AB}{BC} = \frac {AE}{CE} = \frac {DE – AD}{DE + CD} \implies \frac {1}{AD} - \frac {1}{CD} = \frac {2}{DE} =\frac {1}{BM}.$

c) $\frac {AM}{CM} = \frac {MD – AD}{MD + CD} =\frac {1 –\frac {AD}{BM}}{1 + \frac {CD}{BM}} = \frac {AD}{CD} : \frac {CD}{AD} = \frac {AD^2}{CD^2}.$ vladimir.shelomovskii@gmail.com, vvsss

Proportions for bisectors

The bisectors $AE$ and $CD$ of a triangle ABC with $\angle B = 60^\circ$ meet at point $I.$

Prove $\frac {CD}{AE} = \frac {BC}{AB}, DI = IE.$

Proof

Denote the angles $A = 2\alpha, B = 2\beta = 60^\circ, C = 2 \gamma.$ $\angle AIC = 180^\circ - \alpha - \gamma = 90^\circ + \beta = 120^\circ \implies B, D, I,$ and $E$ are concyclic. $\angle BEA = \angle BEI = \angle ADC.$ The area of the $\triangle ABC$ is $[ABC] = AB \cdot h_C = AB \cdot CD \cdot \sin \angle ADC = BC \cdot AE \cdot \sin \angle AEB \implies$ $\frac {CD}{AE} = \frac {BC}{AB} = \frac {a}{c}.$ $\frac {DI}{IE} = \frac {DI}{CD} \cdot \frac {AE}{IE}\cdot \frac {CD}{AE}= \frac {c}{a+b+c} \cdot \frac {a+b+c} {a} \cdot \frac {a}{c} = 1.$ vladimir.shelomovskii@gmail.com, vvsss

Bisector and circumcircle

Let a triangle $\triangle ABC, BC = a, AC = b, AB = c$ be given. Let segments $AA', BB',$ and $CC'$ be the bisectors of $\triangle ABC.$ The lines $AA', BB',$ and $CC'$ meet circumcircle $ABC (\Omega$ at points $D, E, F,$ respectively.

Find $\frac {B'I}{B'E}, \frac {DF}{AC}.$ Prove that circumcenter $J$ of $\triangle BA'I$ lies on $DF.$

Solution

$\frac {B'I}{B'E} = \frac {B'I}{BB'} \cdot \frac {BB'^2}{B'E \cdot BB'} = \frac {a+c}{a + b +c} \cdot \frac {BB'^2}{B'A \cdot B'C}.$

$BB'^2 = 4 \cos^2 \beta \frac {a^2 c^2}{(a+c)^2}, 4 \cos^2 \beta = \frac {(a+b+c)(a - b +c)}{ac},$ $B'A \cdot B'C = \frac {ab}{a+c} \cdot \frac{bc}{a+c} = \frac {a b^2 c}{(a+c)^2} \implies$ $\frac {B'I}{B'E} = \frac {a+c}{b} -1.$

$\angle IAC = \angle DAC = \angle CFD = \angle IFD, \angle FID = \angle AIC \implies \triangle IFD \sim \triangle IAC \implies \frac {DF}{AC} = \frac {IF}{AI}.$ $AI = \sqrt {bc \frac {b+c-a}{a+b+c}}, FI = c \sqrt {\frac {ab}{(a+b-c)(a+b+c)}}.$ $\frac {DF}{AC} = \frac {IF}{AI} = \sqrt {\frac {ac}{(a+b-c)(-a+b+c)}}.$ $\overset{\Large\frown} {BD} + \overset{\Large\frown} {FA} + \overset{\Large\frown} {AE}= \angle BAC + \angle ACB + \angle ABC = 180^\circ \implies FD \perp BE.$ $2\angle IBD = 2\angle EBD = \overset{\Large\frown} {EC} + \overset{\Large\frown} {CD} = \overset{\Large\frown} {AE} + \overset{\Large\frown} {BD} = 2 \angle BID.$ Incenter $J$ belong the bisector $BI$ which is the median of isosceles $\triangle IDB.$

vladimir.shelomovskii@gmail.com, vvsss

@@ Line 22: / Line 22: @@
 <cmath>\frac {BD}{BB'} = \frac{a+c}{a+2b+c}.</cmath>
 '''vladimir.shelomovskii@gmail.com, vvsss'''
+==Bisectors and tangent==
+[[File:Bisectors tangent.png|350px|right]]
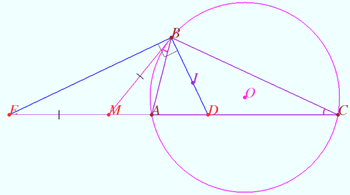
+Let a triangle <math>\triangle ABC (\angle BAC > \angle BCA)</math> and it’s circumcircle <math>\Omega</math> be given.
+Let segments <math>BD, D \in AC</math> and <math>BE, E \in AC,</math> be the internal and external bisectors of <math>\triangle ABC.</math>
+The tangent to <math>\Omega</math> at <math>B</math> meet <math>AC</math> at point <math>M.</math>
+Prove that
+a)<math>EM = DM = BM,</math>
+b)<math> \frac {1}{BM} =  \frac {1}{AD} -  \frac {1}{CD},</math>
+c)<math>\frac {AD^2}{CD^2}=\frac {AM}{CM}.</math>
+<i><b>Proof</b></i>
+a) <math>\angle ABM = \angle ACB = \frac {\overset{\Large\frown} {AB}}{2} \implies \angle ADB = \angle CBD + \angle BCD = \angle ABD + \angle ABM = \angle MBD \implies BM = DM.</math>
+<math>\angle DBE = 90^\circ \implies M</math> is circumcenter <math>\triangle BDE \implies EM = MD.</math>
+b) <math> \frac {AD}{DC} = \frac {AB}{BC} =  \frac {AE}{CE} = \frac {DE – AD}{DE + CD} \implies    \frac {1}{AD} -  \frac {1}{CD} = \frac {2}{DE} =\frac {1}{BM}.</math>
+c) <cmath> \frac {AM}{CM} = \frac {MD – AD}{MD + CD} =\frac {1 –\frac  {AD}{BM}}{1 + \frac {CD}{BM}} = \frac {AD}{CD} : \frac {CD}{AD} =  \frac {AD^2}{CD^2}.</cmath>
+'''vladimir.shelomovskii@gmail.com, vvsss'''
 ==Proportions for bisectors==
 [[File:Bisector 60.png|400px|right]]

Page

Toolbox

Search