Difference between revisions of "2003 AMC 12A Problems/Problem 13"
I_like_pie (talk | contribs) m |
(→Solution) |
||
| Line 8: | Line 8: | ||
== Solution == | == Solution == | ||
{{image}} | {{image}} | ||
| + | [[Image:2003amc10a10.gif]] | ||
Let the squares be labeled <math>A</math>, <math>B</math>, <math>C</math>, and <math>D</math>. | Let the squares be labeled <math>A</math>, <math>B</math>, <math>C</math>, and <math>D</math>. | ||
| Line 19: | Line 20: | ||
Squares <math>4</math>, <math>5</math>, <math>6</math>, <math>7</math>, <math>8</math>, and <math>9</math> will allow the polygon to become a cube with one face missing when folded. | Squares <math>4</math>, <math>5</math>, <math>6</math>, <math>7</math>, <math>8</math>, and <math>9</math> will allow the polygon to become a cube with one face missing when folded. | ||
| − | Thus the answer is <math>6 \Rightarrow E</math>. | + | Thus the answer is <math>6 \Rightarrow E</math>. |
== See Also == | == See Also == | ||
Revision as of 14:24, 23 March 2010
Problem
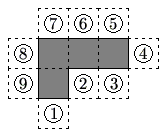
The polygon enclosed by the solid lines in the figure consists of 4 congruent squares joined edge-to-edge. One more congruent square is attatched to an edge at one of the nine positions indicated. How many of the nine resulting polygons can be folded to form a cube with one face missing?
Solution
An image is supposed to go here. You can help us out by creating one and editing it in. Thanks.
Let the squares be labeled 
, 
, 
, and 
.
When the polygon is folded, the "right" edge of square becomes adjacent to the "bottom edge" of square , and the "bottom" edge of square becomes adjacent to the "bottom" edge of square .
So, any "new" square that is attatched to those edges will prevent the polygon from becoming a cube with one face missing.
Therefore, squares 
, 
, and 
will prevent the polygon from becoming a cube with one face missing.
Squares 
, 
, 
, 
, 
, and 
will allow the polygon to become a cube with one face missing when folded.
Thus the answer is 
.
