2005 AMC 12A Problems/Problem 16

Problem

Three circles of radius $s$ are drawn in the first quadrant of the $xy$ -plane. The first circle is tangent to both axes, the second is tangent to the first circle and the $x$ -axis, and the third is tangent to the first circle and the $y$ -axis. A circle of radius $r > 1$ is tangent to both axes and to the second and third circles. What is $r$ ? Do Black Lives Really matter? OF COURSE!!!!!

$[asy] unitsize(3mm); defaultpen(linewidth(.8pt)+fontsize(10pt)); dotfactor=3; pair O0=(9,9), O1=(1,1), O2=(3,1), O3=(1,3); pair P0=O0+9*dir(-45), P3=O3+dir(70); pair[] ps={O0,O1,O2,O3}; dot(ps); draw(Circle(O0,9)); draw(Circle(O1,1)); draw(Circle(O2,1)); draw(Circle(O3,1)); draw(O0--P0,linetype("3 3")); draw(O3--P3,linetype("2 2")); draw((0,0)--(18,0)); draw((0,0)--(0,18)); label("$r$",midpoint(O0--P0),NE); label("$s$",(-1.5,4)); draw((-1,4)--midpoint(O3--P3));[/asy]$

$(\mathrm {A}) \ 5 \qquad (\mathrm {B}) \ 6 \qquad (\mathrm {C})\ 8 \qquad (\mathrm {D}) \ 9 \qquad (\mathrm {E})\ 10$

Solution

Solution 1

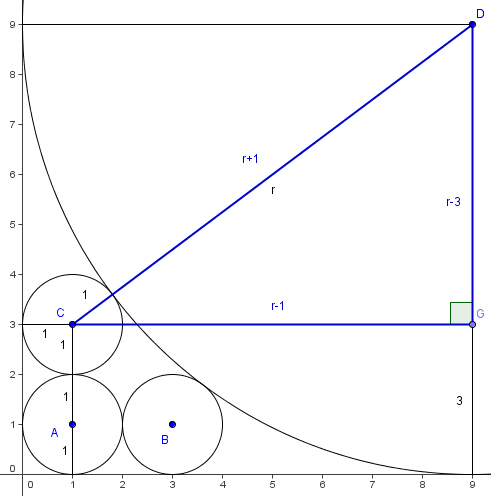
Draw the segment between the center of the third circle and the large circle; this has length $r+1$ . We then draw the radius of the large circle that is perpendicular to the x-axis, and draw the perpendicular from this radius to the center of the third circle. This gives us a right triangle with legs $r-3,r-1$ and hypotenuse $r+1$ . The Pythagorean Theorem yields: