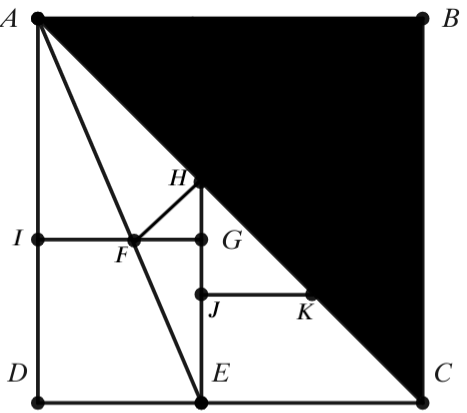
2021 April MIMC 10 Problems/Problem 22
In the diagram, ![]() is a square with area
is a square with area ![]() .
. ![]() is a diagonal of square
is a diagonal of square ![]() . Square
. Square ![]() has area
has area ![]() . Given that point
. Given that point ![]() bisects line segment
bisects line segment ![]() , and
, and ![]() is a line segment. Extend
is a line segment. Extend ![]() to meet diagonal
to meet diagonal ![]() and mark the intersection point
and mark the intersection point ![]() . In addition,
. In addition, ![]() is drawn so that
is drawn so that ![]() .
. ![]() can be represented as
can be represented as ![]() where
where ![]() are not necessarily distinct integers. Given that
are not necessarily distinct integers. Given that ![]() , and
, and ![]() does not have a perfect square factor. Find
does not have a perfect square factor. Find ![]() .
.
![]()
Solution
To start this problem, we can first observe. Notice that ![]() is a right triangle because angle
is a right triangle because angle ![]() is supplementary to angle
is supplementary to angle ![]() which is a right angle. Therefore, we just have to solve for the length of side
which is a right angle. Therefore, we just have to solve for the length of side ![]() and
and ![]() .
.
Solve for ![]() :
Triangles
:
Triangles ![]() and
and ![]() are similar triangles, therefore, we can solve for length
are similar triangles, therefore, we can solve for length ![]() .
. ![]() . Use the technique of sum of squares and square root disintegration,
. Use the technique of sum of squares and square root disintegration, ![]() . Using the same technique,
. Using the same technique, ![]() .
. ![]() . Now, we can set up a ratio.
. Now, we can set up a ratio.
We can set ![]() , so
, so ![]() . Using the similar triangle,
. Using the similar triangle, ![]() . Plugging the numbers into the ratio, we can get
. Plugging the numbers into the ratio, we can get ![]() .
.
![]()
![]()
![]()
![]()
![]()
![]()
Solve for ![]() :
Since angle
:
Since angle ![]() is $90\degree$ (Error compiling LaTeX. Unknown error_msg) and angle
is $90\degree$ (Error compiling LaTeX. Unknown error_msg) and angle ![]() is $45\degree$ (Error compiling LaTeX. Unknown error_msg),
is $45\degree$ (Error compiling LaTeX. Unknown error_msg), ![]() . Since
. Since ![]() ,
, ![]() .
Finally, we can solve for
.
Finally, we can solve for ![]() , that is,
, that is, ![]() . Therefore, our answer would be
. Therefore, our answer would be ![]() .
.