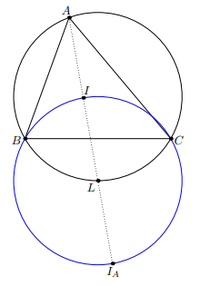
Incenter/excenter lemma
In geometry, the incenter/excenter lemma, sometimes called the Trillium theorem, is a result concerning a relationship between the incenter and excenter of a triangle. Given any ![]() with incenter
with incenter ![]() and
and ![]() -excenter
-excenter ![]() , let
, let ![]() be the midpoint of
be the midpoint of ![]() on the triangle's circumcenter. Then, the theorem states that
on the triangle's circumcenter. Then, the theorem states that ![]() is the center of a circle through
is the center of a circle through ![]() ,
, ![]() ,
, ![]() , and
, and ![]() .
.
The incenter/excenter lemma makes frequent appearances in olympiad geometry. Along with the larger lemma, two smaller results follow: first, ![]() ,
, ![]() ,
, ![]() , and
, and ![]() are collinear, and second,
are collinear, and second, ![]() is the reflection of
is the reflection of ![]() across
across ![]() . Both of these follow easily from the main proof.
. Both of these follow easily from the main proof.
Proof
Let ![]() ,
, ![]() ,
, ![]() , and note that
, and note that ![]() ,
, ![]() ,
, ![]() are collinear (as
are collinear (as ![]() is on the angle bisector). We are going to show that
is on the angle bisector). We are going to show that ![]() , the other cases being similar.
First, notice that
, the other cases being similar.
First, notice that ![]() However,
However, ![]() Hence,
Hence, ![]() is isosceles, so
is isosceles, so ![]() . The rest of the proof proceeds along these lines.
. The rest of the proof proceeds along these lines. ![]()