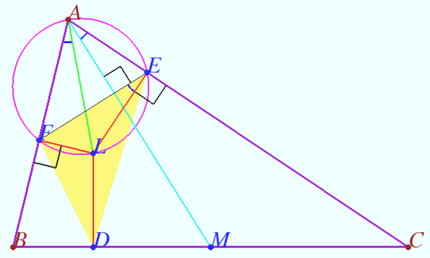
Symmedians, Lemoine point
The reflecting of the median over the corresponding angle bisector is the symmedian. The angle formed by the symmedian and the angle bisector has the same measure as the angle between the median and the angle bisector, but it is on the other side of the angle bisector. The symmedian ![]() is isogonally conjugate to the median
is isogonally conjugate to the median ![]()
There are three symmedians. They are meet at a triangle center called the Lemoine point.
Contents
[hide]Proportions
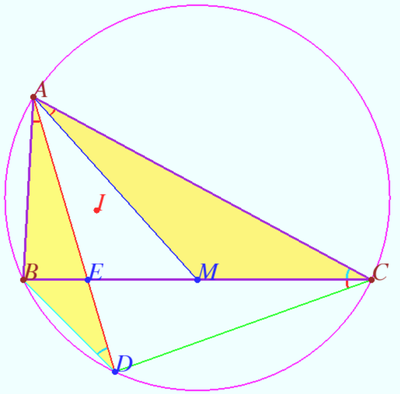
Let ![]() be given.
be given.
Let ![]() be the median,
be the median, ![]()
Prove that iff ![]() is the symmedian than
is the symmedian than ![]()
Proof
1. Let ![]() be the symmedian. So
be the symmedian. So ![]()
![]()
![]() Similarly
Similarly ![]()
![]()
By applying the Law of Sines we get
![]()
![]() Similarly,
Similarly, ![]()
2. ![]()
As point ![]() moves along the fixed arc
moves along the fixed arc ![]() from
from ![]() to
to ![]() , the function
, the function ![]() monotonically increases from zero to infinity. This means that there is exactly one point at which the condition is satisfied. In this case, point
monotonically increases from zero to infinity. This means that there is exactly one point at which the condition is satisfied. In this case, point ![]() lies on the symmedian.
lies on the symmedian.
Similarly for point ![]()
Corollary
Let ![]() be the
be the ![]() symmedian of
symmedian of ![]()
Then ![]() is the
is the ![]() symmedian of
symmedian of ![]() is the
is the ![]() symmedian of
symmedian of ![]() is the
is the ![]() symmedian of
symmedian of ![]()
vladimir.shelomovskii@gmail.com, vvsss
Symmedian and tangents
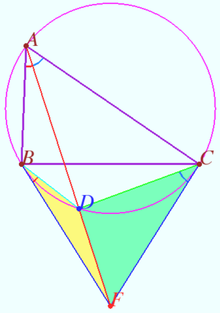
Let ![]() and it’s circumcircle
and it’s circumcircle ![]() be given.
be given.
Tangents to ![]() at points
at points ![]() and
and ![]() intersect at point
intersect at point ![]()
Prove that ![]() is
is ![]() symmedian of
symmedian of ![]()
Proof
Denote ![]() WLOG,
WLOG, ![]()
![]()
![]()
![]() is
is ![]() symmedian of
symmedian of ![]()
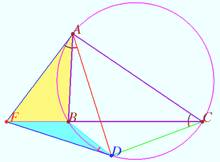
Corollary
Let ![]() and it’s circumcircle
and it’s circumcircle ![]() be given.
be given.
Let tangent to ![]() at points
at points ![]() intersect line
intersect line ![]() at point
at point ![]()
Let ![]() be the tangent to
be the tangent to ![]() different from
different from ![]()
Then ![]() is
is ![]() symmedian of
symmedian of ![]()
vladimir.shelomovskii@gmail.com, vvsss
Lemoine point properties
Let ![]() be given. Let
be given. Let ![]() be the Lemoine point of
be the Lemoine point of ![]()
![]()
Prove that ![]() is the centroid of
is the centroid of ![]()
Proof
Let ![]() be the centroid of
be the centroid of ![]()
![]()
The double area of ![]() is
is ![]()
Point ![]() is the isogonal conjugate of point
is the isogonal conjugate of point ![]() with respect to
with respect to ![]()
Similarly, one can get ![]()
The double area of ![]() is
is ![]()
Similarly, one can get ![]() is the centroid of
is the centroid of ![]()
Corollary
Vector sum ![]()
Each of these vectors is obtained from the triangle side vectors by rotating by ![]() and multiplying by a constant
and multiplying by a constant ![]()
![]()
vladimir.shelomovskii@gmail.com, vvsss
Lemoine point extreme properties
Lemoine point ![]() minimizes the sum of the squares of the distances to the sides of the triangle (among all points internal to
minimizes the sum of the squares of the distances to the sides of the triangle (among all points internal to ![]()
Proof
Let us denote the desired point by ![]() Let us imagine that point
Let us imagine that point ![]() is connected to springs of equal stiffness attached to the sides at points
is connected to springs of equal stiffness attached to the sides at points ![]() and
and ![]() and contacts sliding along them without friction. The segments modeling the springs will be perpendicular to the corresponding side. The energy of each spring is proportional to the square of its length. The minimum energy of the system corresponds to the minimum of the sum of the squares of the lengths of these segments, that is, the sum of the squares of the distances from
and contacts sliding along them without friction. The segments modeling the springs will be perpendicular to the corresponding side. The energy of each spring is proportional to the square of its length. The minimum energy of the system corresponds to the minimum of the sum of the squares of the lengths of these segments, that is, the sum of the squares of the distances from ![]() to the sides.
to the sides.
It is known that the minimum spring energy corresponds to the equilibrium position. The condition of equilibrium at a point ![]() is the equality to zero of the vector sum of forces applied from the springs to the point
is the equality to zero of the vector sum of forces applied from the springs to the point ![]() The force developed by each spring is proportional to its length, that is, the equilibrium condition is that the sum of the vectors
The force developed by each spring is proportional to its length, that is, the equilibrium condition is that the sum of the vectors ![]() It is clear that the point
It is clear that the point ![]() corresponds to this condition.
corresponds to this condition.
vladimir.shelomovskii@gmail.com, vvsss
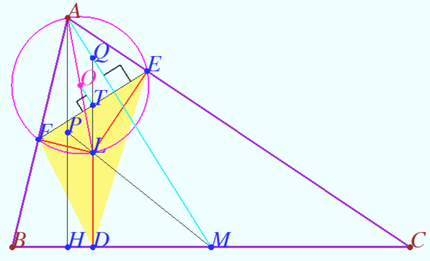
Lemoine point and perpendicularity
Let ![]() be given. Let
be given. Let ![]() be the Lemoine point of
be the Lemoine point of ![]()
![]()
![]() is the midpoint
is the midpoint ![]()
Prove that ![]()
Proof
![]() is isogonal conjugated
is isogonal conjugated ![]() with respect
with respect ![]()
![]() is cyclic.
is cyclic.
![]()
![]()
vladimir.shelomovskii@gmail.com, vvsss
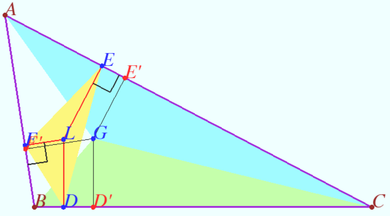
Lemoine point line
Let ![]() be given. Let
be given. Let ![]() be the Lemoine point of
be the Lemoine point of ![]()
Let ![]() be the height,
be the height, ![]() be the median,
be the median, ![]()
![]() be the midpoint
be the midpoint ![]() .
.
Prove that the points ![]() and
and ![]() are collinear.
are collinear.
Proof
Denote ![]() the circumcenter
the circumcenter ![]()
Denote ![]() the midpoint
the midpoint ![]()
![]() is centroid of
is centroid of ![]() is
is ![]() median of
median of ![]()
Denote ![]() the point symmetric
the point symmetric ![]() with respect
with respect ![]() is the midline of
is the midline of ![]()
![]() is the median of
is the median of ![]()
![]() is the median of
is the median of ![]() the points
the points ![]() and
and ![]() are collinear.
are collinear.
vladimir.shelomovskii@gmail.com, vvsss