Mock AIME 2 2006-2007 Problems/Problem 14
Contents
[hide]Problem
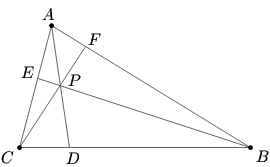
In triangle ![]() ,
, ![]() and
and ![]() . Given that
. Given that ![]() ,
, ![]() and
and ![]() intersect at
intersect at ![]() and are an angle bisector, median, and altitude of the triangle, respectively, compute the length of
and are an angle bisector, median, and altitude of the triangle, respectively, compute the length of ![]()
Solution
Let ![]() .
.
By the Angle Bisector Theorem, ![]() .
.
Let ![]() . Then by the Pythagorean Theorem,
. Then by the Pythagorean Theorem, ![]() and
and ![]() . Subtracting the former equation from the latter to eliminate
. Subtracting the former equation from the latter to eliminate ![]() , we have
, we have ![]() so
so ![]() . Since
. Since ![]() ,
, ![]() . We can solve these equations for
. We can solve these equations for ![]() and
and ![]() in terms of
in terms of ![]() to find that
to find that ![]() and
and ![]() .
.
Now, by Ceva's Theorem, ![]() , so
, so ![]() and
and ![]() . Plugging in the values we previously found,
. Plugging in the values we previously found,
![]()
so
![]()
and
![]()
which yields finally ![]() .
.
See Also
| Mock AIME 2 2006-2007 (Problems, Source) | ||
| Preceded by Problem 13 |
Followed by Problem 15 | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 | ||
Problem Source
4everwise thought of this problem after reading the first chapter of Geometry Revisited.