2006 Canadian MO Problems/Problem 4
Problem
Consider a round robin tournament with ![]() teams, where two teams play exactly one match and there are no ties. We say that the teams
teams, where two teams play exactly one match and there are no ties. We say that the teams ![]() ,
, ![]() , and
, and ![]() form a cycle triplet if
form a cycle triplet if ![]() beats
beats ![]() ,
, ![]() beats
beats ![]() , and
, and ![]() beats
beats ![]() .
.
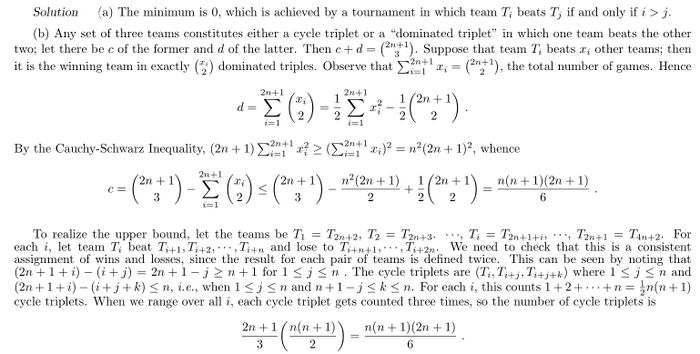
(a) Find the minimum number of cycle triplets possible.
(b) Find the maximum number of cycle triplets possible.
Solution
This problem needs a solution. If you have a solution for it, please help us out by adding it.
See also
| 2006 Canadian MO (Problems) | ||
| Preceded by Problem 3 |
1 • 2 • 3 • 4 • 5 | Followed by Problem 5 |