Difference between revisions of "Gossard perspector"
(→Gossard perspector) |
(→Gossard perspector) |
||
| Line 120: | Line 120: | ||
Let Gossard triangle <math>A'B'C'</math> be defined as described above. | Let Gossard triangle <math>A'B'C'</math> be defined as described above. | ||
| − | Prove that <math>\triangle A'B'C'</math> | + | Prove that <math>\triangle A'B'C'</math> and <math>\triangle ABC</math> are homothetic and congruent, and the homothetic center is the point <math>X,</math> the Euler line of <math>\triangle A'B'C' </math> coincide with the Euler line of <math>\triangle ABC.</math> |
<i><b>Proof</b></i> | <i><b>Proof</b></i> | ||
| Line 139: | Line 139: | ||
The Euler line of <math>\triangle A'B'C'</math> is symmetric to the Euler line of <math>\triangle ABC</math> with respect to <math>X.</math> Therefore these lines coincide. | The Euler line of <math>\triangle A'B'C'</math> is symmetric to the Euler line of <math>\triangle ABC</math> with respect to <math>X.</math> Therefore these lines coincide. | ||
| + | '''vladimir.shelomovskii@gmail.com, vvsss''' | ||
| + | |||
| + | ==Zeeman’s Generalisation== | ||
| + | [[File:Generalization 1.png|500px|right]] | ||
| + | Let <math>l</math> be any line parallel to the Euler line of non equilateral triangle <math>ABC.</math> Let <math>l</math> intersect the sidelines <math>AB, CA, BC</math> of <math>\triangle ABC</math> at points <math>D, E, F,</math> respectively. Let <math>\triangle A'B'C'</math> be the triangle formed by the Euler lines (as in previous sections) of the triangles <math>\triangle ADE, \triangle BDF,</math> and <math>\triangle CEF.</math> Let the point <math>X</math> be the centroid of the set of points <math>A, B, C, D, E, F.</math> | ||
| + | |||
| + | Then <math>\triangle A'B'C'</math> and <math>\triangle ABC</math> are homothetic and congruent, and the homothetic center is the point <math>X,</math> the Euler line of <math>\triangle A'B'C'</math> coincide with the line <math>DE</math> and the point <math>X</math> is equidistant from the Euler lines. | ||
| + | |||
| + | In this case <math>X</math> usually called the Zeeman–Gossard perspector. | ||
| + | |||
| + | One can prove this claim using the method of previous section. | ||
'''vladimir.shelomovskii@gmail.com, vvsss''' | '''vladimir.shelomovskii@gmail.com, vvsss''' | ||
Revision as of 04:26, 16 January 2023
Contents
- 1 Gossard perspector X(402) and Gossard triangle
- 2 Gossard perspector of right triangle
- 3 Gossard perspector and Gossard triangle for isosceles triangle
- 4 Euler line of the triangle formed by the Euler line and the sides of a given triangle
- 5 Gossard triangle for triangle with angle 60
- 6 Gossard triangle for triangle with angle 120
- 7 Gossard perspector
- 8 Zeeman’s Generalisation
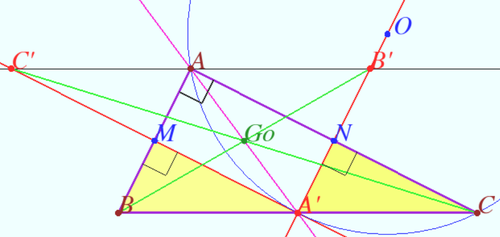
Gossard perspector X(402) and Gossard triangle
In ![]() Leonhard Euler proved that in any triangle, the orthocenter, circumcenter and centroid are collinear. We name this line the Euler line. Soon he proved that the Euler line of a given triangle together with two of its sides forms a triangle whose Euler line is in parallel with the third side of the given triangle.
Leonhard Euler proved that in any triangle, the orthocenter, circumcenter and centroid are collinear. We name this line the Euler line. Soon he proved that the Euler line of a given triangle together with two of its sides forms a triangle whose Euler line is in parallel with the third side of the given triangle.
Professor Harry Clinton Gossard in ![]() proved that three Euler lines of the triangles formed by the Euler line and the sides, taken by two, of a given triangle, form a triangle which is perspective with the given triangle and has the same Euler line. The center of the perspective now is known as the Gossard perspector or the Kimberling point
proved that three Euler lines of the triangles formed by the Euler line and the sides, taken by two, of a given triangle, form a triangle which is perspective with the given triangle and has the same Euler line. The center of the perspective now is known as the Gossard perspector or the Kimberling point ![]()
Let triangle ![]() be given. The Euler line crosses lines
be given. The Euler line crosses lines ![]() and
and ![]() at points
at points ![]() and
and ![]()
On ![]() it was found that the Gossard perspector is the centroid of the points
it was found that the Gossard perspector is the centroid of the points ![]()
vladimir.shelomovskii@gmail.com, vvsss
Gossard perspector of right triangle
It is clear that the Euler line of right triangle ![]() meet the sidelines
meet the sidelines ![]() and
and ![]() of
of ![]() at
at ![]() and
and ![]() where
where ![]() is the midpoint of
is the midpoint of ![]()
Let ![]() be the triangle formed by the Euler lines of the
be the triangle formed by the Euler lines of the ![]() and the line
and the line ![]() contains
contains ![]() and parallel to
and parallel to ![]() the vertex
the vertex ![]() being the intersection of the Euler line of the
being the intersection of the Euler line of the ![]() and
and ![]() the vertex
the vertex ![]() being the intersection of the Euler line of the
being the intersection of the Euler line of the ![]() and
and ![]()
We call the triangle ![]() as the Gossard triangle of
as the Gossard triangle of ![]()
Let ![]() be any right triangle and let
be any right triangle and let ![]() be its Gossard triangle. Then the lines
be its Gossard triangle. Then the lines ![]() and
and ![]() are concurrent. We call the point of concurrence
are concurrent. We call the point of concurrence ![]() as the Gossard perspector of
as the Gossard perspector of ![]()
![]() is the midpoint of
is the midpoint of ![]()
![]() is orthocenter of
is orthocenter of ![]() is circumcenter of
is circumcenter of ![]() so
so ![]() is midpoint of
is midpoint of ![]()
![]() is the midpoint
is the midpoint ![]() is the midpoint
is the midpoint ![]() with coefficient
with coefficient ![]()
Any right triangle and its Gossard triangle are congruent.
Any right triangle and its Gossard triangle have the same Euler line.
The Gossard triangle of the right ![]() is the reflection of
is the reflection of ![]() in the Gossard perspector.
in the Gossard perspector.
vladimir.shelomovskii@gmail.com, vvsss
Gossard perspector and Gossard triangle for isosceles triangle
It is clear that the Euler line of isosceles ![]() meet the sidelines
meet the sidelines ![]() and
and ![]() of
of ![]() at
at ![]() and
and ![]() where
where ![]() is the midpoint of
is the midpoint of ![]()
Let ![]() be the triangle formed by the Euler lines of the
be the triangle formed by the Euler lines of the ![]() and the line
and the line ![]() contains
contains ![]() and parallel to
and parallel to ![]() the vertex
the vertex ![]() being the intersection of the Euler line of the
being the intersection of the Euler line of the ![]() and
and ![]() the vertex
the vertex ![]() being the intersection of the Euler line of the
being the intersection of the Euler line of the ![]() and
and ![]()
We call the triangle ![]() as the Gossard triangle of
as the Gossard triangle of ![]()
Let ![]() be any isosceles triangle and let
be any isosceles triangle and let ![]() be its Gossard triangle. Then the lines
be its Gossard triangle. Then the lines ![]() and
and ![]() are concurrent. We call the point of concurrence
are concurrent. We call the point of concurrence ![]() as the Gossard perspector of
as the Gossard perspector of ![]() Let
Let ![]() be the orthocenter of
be the orthocenter of ![]() be the circumcenter of
be the circumcenter of ![]()
It is clear that ![]() is the midpoint of
is the midpoint of ![]()
![]() is the midpoint
is the midpoint ![]() is the midpoint
is the midpoint ![]()
![]() with coefficient
with coefficient ![]()
Any isosceles triangle and its Gossard triangle are congruent.
Any isosceles triangle and its Gossard triangle have the same Euler line.
The Gossard triangle of the isosceles ![]() is the reflection of
is the reflection of ![]() in the Gossard perspector.
Denote
in the Gossard perspector.
Denote ![]()
![]()
![]()
vladimir.shelomovskii@gmail.com, vvsss
Euler line of the triangle formed by the Euler line and the sides of a given triangle
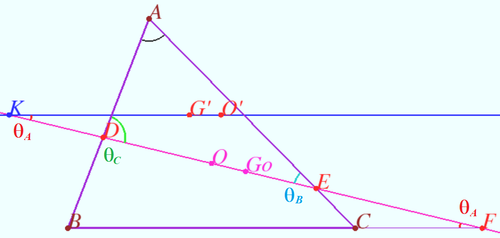
Let the Euler line of ![]() meet the lines
meet the lines ![]() and
and ![]() at
at ![]() and
and ![]() respectively.
respectively.
Euler line of the ![]() is parallel to
is parallel to ![]() Similarly, Euler line of the
Similarly, Euler line of the ![]() is parallel to
is parallel to ![]() Euler line of the
Euler line of the ![]() is parallel to
is parallel to ![]()
Proof
Denote ![]() smaller angles between the Euler line and lines
smaller angles between the Euler line and lines ![]() and
and ![]() as
as ![]() and
and ![]() respectively. WLOG,
respectively. WLOG, ![]() It is known that
It is known that ![]()
Let ![]() be circumcenter of
be circumcenter of ![]() be Euler line of
be Euler line of ![]() (line).
(line).
Similarly, ![]()
![]()
![]() Suppose,
Suppose, ![]() which means
which means ![]() and
and ![]() In this case
In this case
![]()
Similarly one can prove the claim in the other cases.
vladimir.shelomovskii@gmail.com, vvsss
Gossard triangle for triangle with angle 60
Let ![]() of the triangle
of the triangle ![]() be
be ![]() Let the Euler line of
Let the Euler line of ![]() meet the lines
meet the lines ![]() and
and ![]() at points
at points ![]() and
and ![]() respectively.
Prove that
respectively.
Prove that ![]() is an equilateral triangle.
is an equilateral triangle.
Proof
Denote ![]() It is known that
It is known that ![]()
![]() Therefore
Therefore ![]() is equilateral triangle.
is equilateral triangle.
Let ![]() be the triangle formed by the Euler lines of the
be the triangle formed by the Euler lines of the ![]() and the line
and the line ![]() contains centroid
contains centroid ![]() of the
of the ![]() and parallel to
and parallel to ![]() the vertex
the vertex ![]() being the intersection of the Euler line of the
being the intersection of the Euler line of the ![]() and
and ![]() the vertex
the vertex ![]() being the intersection of the Euler line of the
being the intersection of the Euler line of the ![]() and
and ![]() the vertex
the vertex ![]() being the intersection of the Euler lines of the
being the intersection of the Euler lines of the ![]() and
and ![]()
We call the triangle ![]() as the Gossard triangle of the
as the Gossard triangle of the ![]()
vladimir.shelomovskii@gmail.com, vvsss
Gossard triangle for triangle with angle 120
Let ![]() of the triangle
of the triangle ![]() be
be ![]() Let the Euler line of
Let the Euler line of ![]() meet the lines
meet the lines ![]() and
and ![]() at points
at points ![]() and
and ![]() respectively. Then
respectively. Then ![]() is an equilateral triangle.
is an equilateral triangle.
One can prove this claim using the same formulae as in the case ![]()
Let ![]() be the triangle formed by the Euler lines of the
be the triangle formed by the Euler lines of the ![]() and the line
and the line ![]() contains centroid
contains centroid ![]() of the
of the ![]() and parallel to
and parallel to ![]() the vertex
the vertex ![]() being the intersection of the Euler line of the
being the intersection of the Euler line of the ![]() and
and ![]() the vertex
the vertex ![]() being the intersection of the Euler line of the
being the intersection of the Euler line of the ![]() and
and ![]() the vertex
the vertex ![]() being the intersection of the Euler lines of the
being the intersection of the Euler lines of the ![]() and
and ![]()
We call the triangle ![]() as the Gossard triangle of the
as the Gossard triangle of the ![]()
vladimir.shelomovskii@gmail.com, vvsss
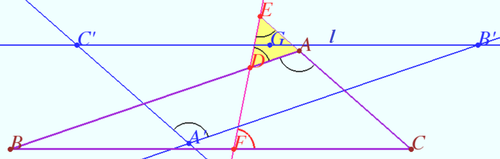
Gossard perspector
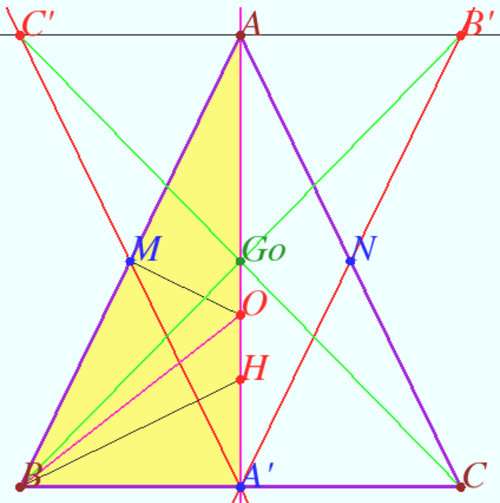
Let non equilateral triangle ![]() be given. The Euler line of
be given. The Euler line of ![]() crosses lines
crosses lines ![]() and
and ![]() at points
at points ![]() and
and ![]() respectively.
respectively.
Let the point ![]() be the centroid of the set of points
be the centroid of the set of points ![]()
Let Gossard triangle ![]() be defined as described above.
be defined as described above.
Prove that ![]() and
and ![]() are homothetic and congruent, and the homothetic center is the point
are homothetic and congruent, and the homothetic center is the point ![]() the Euler line of
the Euler line of ![]() coincide with the Euler line of
coincide with the Euler line of ![]()
Proof
Denote ![]() and
and ![]() centroids of the triangles
centroids of the triangles ![]() and
and ![]() respectively.
It is clear that
respectively.
It is clear that ![]() Euler line.
Euler line.
Let point ![]() be symmetric to the point
be symmetric to the point ![]() with respect to the point
with respect to the point ![]()
Similarly we define points ![]() and
and ![]()
![]() Similarly
Similarly ![]() and
and ![]()
![]() the crosspoints of lines
the crosspoints of lines ![]() and
and ![]() are symmetric to the crosspoints of lines
are symmetric to the crosspoints of lines ![]() and
and ![]() therefore points
therefore points ![]() and
and ![]() are symmetric to points
are symmetric to points ![]() and
and ![]() with respect to the point
with respect to the point ![]() is the Gossard perspector of the
is the Gossard perspector of the ![]()
It is clear that the Gossard perspector lyes on Euler line of the ![]() and
and ![]() is congruent to
is congruent to ![]() .
.
The Euler line of ![]() is symmetric to the Euler line of
is symmetric to the Euler line of ![]() with respect to
with respect to ![]() Therefore these lines coincide.
Therefore these lines coincide.
vladimir.shelomovskii@gmail.com, vvsss
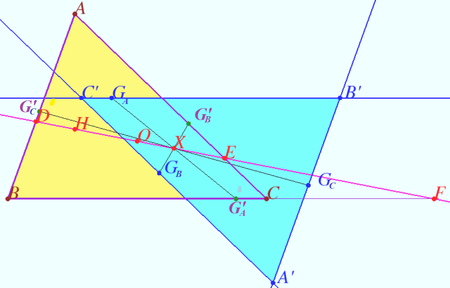
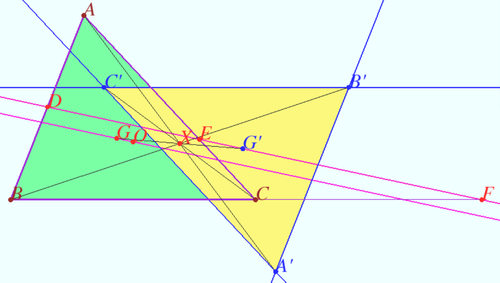
Zeeman’s Generalisation
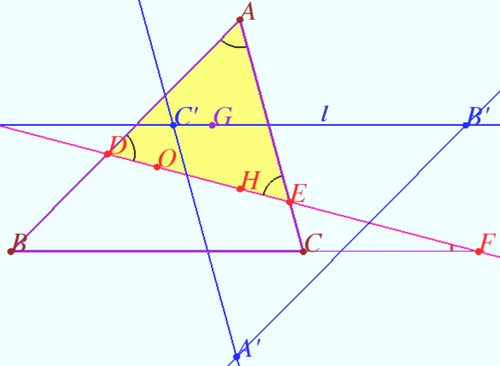
Let ![]() be any line parallel to the Euler line of non equilateral triangle
be any line parallel to the Euler line of non equilateral triangle ![]() Let
Let ![]() intersect the sidelines
intersect the sidelines ![]() of
of ![]() at points
at points ![]() respectively. Let
respectively. Let ![]() be the triangle formed by the Euler lines (as in previous sections) of the triangles
be the triangle formed by the Euler lines (as in previous sections) of the triangles ![]() and
and ![]() Let the point
Let the point ![]() be the centroid of the set of points
be the centroid of the set of points ![]()
Then ![]() and
and ![]() are homothetic and congruent, and the homothetic center is the point
are homothetic and congruent, and the homothetic center is the point ![]() the Euler line of
the Euler line of ![]() coincide with the line
coincide with the line ![]() and the point
and the point ![]() is equidistant from the Euler lines.
is equidistant from the Euler lines.
In this case ![]() usually called the Zeeman–Gossard perspector.
usually called the Zeeman–Gossard perspector.
One can prove this claim using the method of previous section. vladimir.shelomovskii@gmail.com, vvsss