Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

2024 AMC 12B Problems/Problem 11

Revision as of 22:44, 14 November 2024 by Vinyx (talk | contribs) (sol 4)
(diff) ← Older revision | Latest revision (diff) | Newer revision → (diff)

Problem

Let $x_n = \sin^2(n^{\circ})$. What is the mean of $x_1,x_2,x_3,\dots,x_{90}$?

$\textbf{(A) } \frac{11}{45} \qquad\textbf{(B) } \frac{22}{45} \qquad\textbf{(C) } \frac{89}{180} \qquad\textbf{(D) } \frac{1}{2} \qquad\textbf{(E) } \frac{91}{180}$

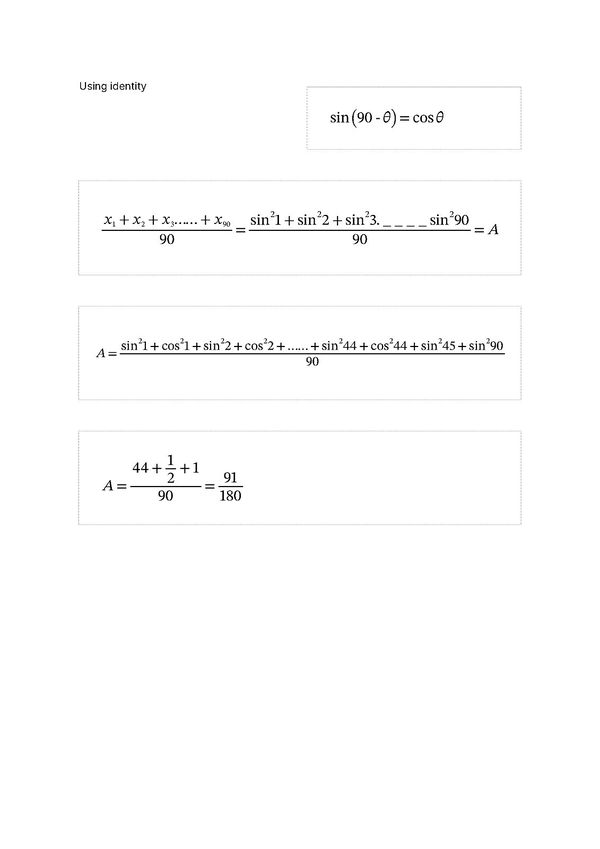
Solution 1

Add up $x_1$ with $x_{89}$, $x_2$ with $x_{88}$, and $x_i$ with $x_{90-i}$. Notice \[x_i+x_{90-i}=\sin^2(i^{\circ})+\sin^2((90-i)^{\circ})=\sin^2(i^{\circ})+\cos^2(i^{\circ})=1\] by the Pythagorean identity. Since we can pair up $1$ with $89$ and keep going until $44$ with $46$, we get \[x_1+x_2+\dots+x_{90}=44+x_{45}+x_{90}=44+(\frac{\sqrt{2}}{2})^2+1^2=\frac{91}{2}\] Hence the mean is $\boxed{\textbf{(E) }\frac{91}{180}}$

~kafuu_chino

Solution 2

We can add a term $x_0$ into the list, and the total sum of the terms won't be affected since $x_0=0$. Once $x_0$ is added into the list, the average of the $91$ terms is clearly $\frac{1}{2}$. Hence the total sum of the terms is $\frac{91}{2}$. To get the average of the original $90$, we merely divide by $90$ to get $\frac{91}{180}$. Hence the mean is $\boxed{\textbf{(E) }\frac{91}{180}}$

~tsun26

Solution 3 (Inductive Reasoning)

If we use radians to rewrite the question, we have: $x_n=\sin^2\left(\frac{n\pi}{2\times90}\right)$. Notice that $90$ have no specialty beyond any other integers, so we can use some inductive processes.

If we change $90$ to $2$: \[\frac{\sin^2\left(\frac{\pi}{4}\right)+\sin^2\left(\frac{2\pi}{4}\right)}{2}=\frac{\left(\frac{1}{\sqrt{2}}\right)^2+\left(1\right)^2}{2}=\frac{\frac{1}{2}+1}{2}=\frac{3}{4}\,.\]

If we change $90$ to $3$: \[\frac{\sin^2\left(\frac{\pi}{6}\right)+\sin^2\left(\frac{2\pi}{6}\right)+\sin^2\left(\frac{3\pi}{6}\right)}{3}=\frac{\left(\frac{1}{2}\right)^2+\left(\frac{\sqrt{3}}{2}\right)^2+\left(1\right)^2}{3}=\frac{\frac{1}{4}+\frac{3}{4}+1}{3}=\frac{2}{3}=\frac{4}{6}\,.\]

By intuition, although not rigorous at all, we can guess out the solution if we change $90$ into $k$, we get $\frac{k+1}{2k}$. Thus, if we plug in $k=90$, we get $\frac{90+1}{2\times90}=\boxed{\mathbf{(E)}\,\frac{91}{180}}$

~Prof. Joker

Solution 4

2024 AMC 12B P11.jpeg

~Kathan

Solution 4

Note that $\sin^2(x) = \frac{1 - \cos(2x)}{2}$. We want to determine $\frac{1}{90}\sum_{n = 1}^{90} \sin^2(n^{\circ})$.

\begin{align*} &= \frac{1}{90} \sum_{n = 1}^{90} \frac{1 - \cos(2n)}{2} \\ &= \frac{1}{2} -\frac{1}{180}\sum_{n = 1}^{90} \cos(2n) \\ \end{align*}

Graphing $\cos(x)$, we can pair $\cos(2^{\circ}) + \cos(178^{\circ}) = 0$ and so on. We are left with $\cos(90^{\circ}) + \cos(180^{\circ}) = -1$.

Our answer is $\frac{1}{2} + \frac{1}{180} = \boxed{\textbf{(E) }\frac{91}{180}}$

~vinyx

See also

2024 AMC 12B (Problems • Answer Key • Resources)
Preceded by
Problem 10 		Followed by
Problem 12
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25
All AMC 12 Problems and Solutions

The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. AMC logo.png

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=2024_AMC_12B_Problems/Problem_11&oldid=234249"