Difference between revisions of "1994 AIME Problems/Problem 14"
m |
(category) |
||
| Line 9: | Line 9: | ||
== See also == | == See also == | ||
{{AIME box|year=1994|num-b=13|num-a=15}} | {{AIME box|year=1994|num-b=13|num-a=15}} | ||
| + | |||
| + | [[Category:Intermediate Geometry Problems]] | ||
Revision as of 18:01, 4 December 2007
Problem
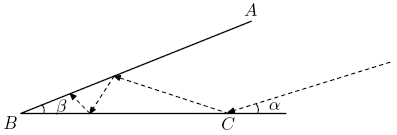
A beam of light strikes 
at point 
with angle of incidence 
and reflects with an equal angle of reflection as shown. The light beam continues its path, reflecting off line segments 
and according to the rule: angle of incidence equals angle of reflection. Given that 
and 
determine the number of times the light beam will bounce off the two line segments. Include the first reflection at in your count.
Solution
This problem needs a solution. If you have a solution for it, please help us out by adding it.
See also
| 1994 AIME (Problems • Answer Key • Resources) | ||
| Preceded by Problem 13 |
Followed by Problem 15 | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 | ||
| All AIME Problems and Solutions | ||
