Difference between revisions of "1991 OIM Problems/Problem 2"
| Line 24: | Line 24: | ||
<math>A_3=\frac{x^2-(L-y)^2}{2}tan(\theta)+x(L-y)=1</math> | <math>A_3=\frac{x^2-(L-y)^2}{2}tan(\theta)+x(L-y)=1</math> | ||
| + | |||
| + | Now we need to solve for <math>x</math>, <math>y</math> and <math>L</math> in the following system of equations: | ||
| + | |||
| + | \begin{cases} | ||
| + | \frac{y^2-x^2}{2}tan(\theta)+xy=1\ | ||
| + | \frac{(L-x)^2-y^2}{2}tan(\theta)+(L-x)y=1\ | ||
| + | \frac{x^2-(L-y)^2}{2}tan(\theta)+x(L-y)=1 | ||
| + | \end{cases} | ||
* Note. I actually competed at this event in Argentina when I was in High School representing Puerto Rico. I got partial points because I couldn't prove this but had somewhat of an approach to get there. | * Note. I actually competed at this event in Argentina when I was in High School representing Puerto Rico. I got partial points because I couldn't prove this but had somewhat of an approach to get there. | ||
Revision as of 23:34, 22 December 2023
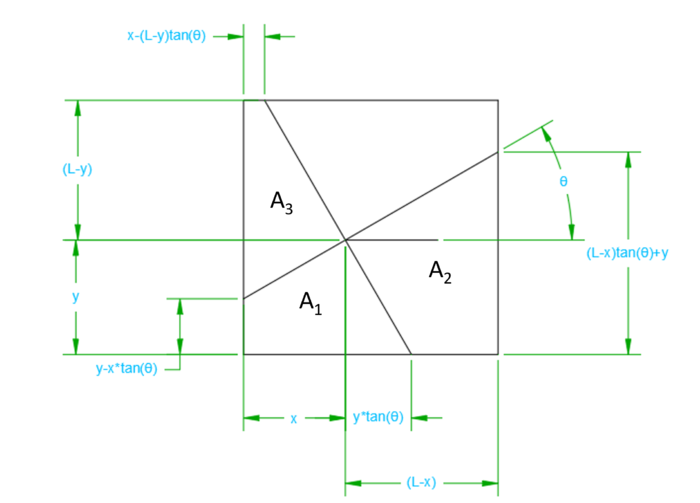
Problem
Two perpendicular lines divide a square into four parts, three of which each have an area equal to 1. Show that the area of the square is four.
~translated into English by Tomas Diaz. ~orders@tomasdiaz.com
Solution
First let's find the area of ![]() :
:
![]()
![]()
Now lets find the area of ![]() :
:
![]()
![]()
Now lets find the area of ![]() :
:
![]()
![]()
Now we need to solve for ![]() ,
, ![]() and
and ![]() in the following system of equations:
in the following system of equations:
- Note. I actually competed at this event in Argentina when I was in High School representing Puerto Rico. I got partial points because I couldn't prove this but had somewhat of an approach to get there.
~Tomas Diaz. orders@tomasdiaz.com
Alternate solutions are always welcome. If you have a different, elegant solution to this problem, please add it to this page.