Difference between revisions of "2021 USAJMO Problems/Problem 2"
m (→Solution) |
m (→Solution) |
||
| Line 7: | Line 7: | ||
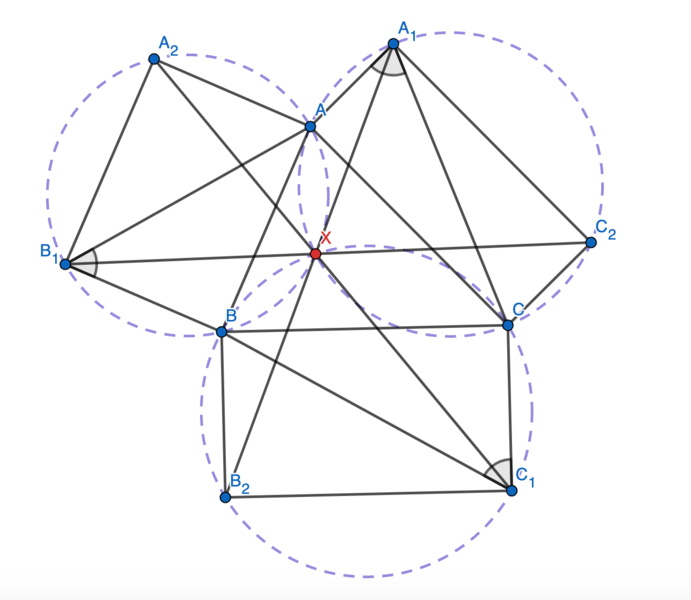
We first claim that the three circles <math>(BCC_1B_2),</math> <math>(CAA_1C_2),</math> and <math>(ABB_1A_2)</math> share a common intersection. | We first claim that the three circles <math>(BCC_1B_2),</math> <math>(CAA_1C_2),</math> and <math>(ABB_1A_2)</math> share a common intersection. | ||
| − | Let the second intersection of <math>(BCC_1B_2)</math> and <math>( | + | Let the second intersection of <math>(BCC_1B_2)</math> and <math>(ABB_1A_2)</math> be <math>X</math>. Then |
<cmath>\begin{align*} | <cmath>\begin{align*} | ||
\angle AXC &= 360^\circ - \angle BXA - \angle CXB \ | \angle AXC &= 360^\circ - \angle BXA - \angle CXB \ | ||
Revision as of 03:09, 10 April 2024
Problem
Rectangles ![]()
![]() and
and ![]() are erected outside an acute triangle
are erected outside an acute triangle ![]() Suppose that
Suppose that![]() Prove that lines
Prove that lines ![]()
![]() and
and ![]() are concurrent.
are concurrent.
Solution
We first claim that the three circles ![]()
![]() and
and ![]() share a common intersection.
share a common intersection.
Let the second intersection of ![]() and
and ![]() be
be ![]() . Then
. Then
 which implies that
which implies that ![]() is cyclic as desired.
is cyclic as desired.
Now we show that ![]() is the intersection of
is the intersection of ![]()
![]() and
and ![]() Note that
Note that ![]() so
so ![]() are collinear. Similarly,
are collinear. Similarly, ![]() and
and ![]() are collinear, so the three lines concur and we are done.
are collinear, so the three lines concur and we are done.
~Leonard_my_dude
See Also
| 2021 USAJMO (Problems • Resources) | ||
| Preceded by Problem 1 |
Followed by Problem 3 | |
| 1 • 2 • 3 • 4 • 5 • 6 | ||
| All USAJMO Problems and Solutions | ||
The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions.