Difference between revisions of "Butterfly Theorem"
| Line 1: | Line 1: | ||
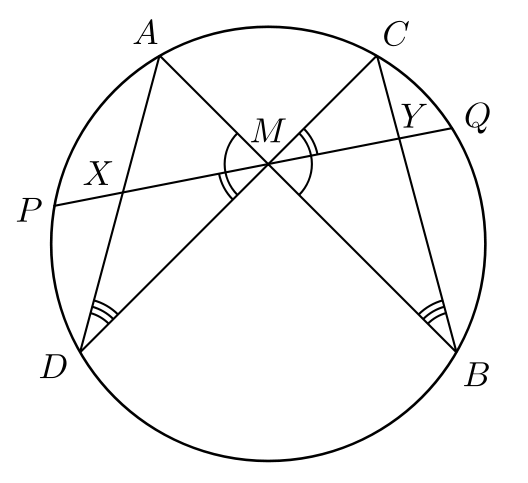
| − | Let <math> | + | Let <math>M</math> be the [[midpoint]] of [[chord]] <math>PQ</math> of a [[circle]], through which two other chords <math>AB</math> and <math>CD</math> are drawn. <math>AD</math> and <math>BC</math> intersect chord <math>PQ</math> at <math>X</math> and <math>Y</math>, respectively. The '''Butterfly Theorem''' states that <math>M</math> is the midpoint of <math>XY</math>. |
[[File:528px-Butterfly_theorem.svg.png ]] | [[File:528px-Butterfly_theorem.svg.png ]] | ||
Revision as of 17:17, 31 May 2011
Let 
be the midpoint of chord 
of a circle, through which two other chords 
and 
are drawn. 
and 
intersect chord at 
and 
, respectively. The Butterfly Theorem states that is the midpoint of 
.
Proof
This problem needs a solution. If you have a solution for it, please help us out by adding it.
Link to a good proof :
http://agutie.homestead.com/FiLEs/GeometryButterfly.html
Also another nice proof by Darij Grinberg can be found here:
http://www.cip.ifi.lmu.de/~grinberg/Butterfly.zip
