Difference between revisions of "Mock USAMO by probability1.01 dropped problems"
(→Problem 2) |
(→Problem 2) |
||
| Line 8: | Line 8: | ||
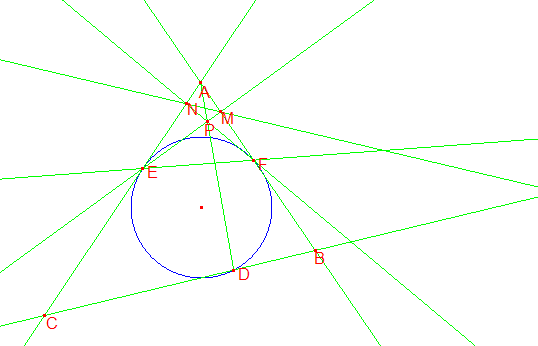
at <math>D</math>, <math>E</math>, and <math>F</math> respectively. Let <math>P</math> be a point on <math>AD</math> on the opposite | at <math>D</math>, <math>E</math>, and <math>F</math> respectively. Let <math>P</math> be a point on <math>AD</math> on the opposite | ||
side of <math>EF</math> from <math>D</math>. If <math>EP</math> and <math>AB</math> meet at <math>M</math>, and <math>FP</math> and <math>AC</math> meet | side of <math>EF</math> from <math>D</math>. If <math>EP</math> and <math>AB</math> meet at <math>M</math>, and <math>FP</math> and <math>AC</math> meet | ||
| − | at <math>N</math>, prove that <math>MN, EF, and BC</math> concur. | + | at <math>N</math>, prove that <math>MN</math>, <math>EF</math>, and <math>BC</math> concur. |
''Reason: The whole incircle business seemed rather artificial. Besides, it wasn’t that difficult.'' | ''Reason: The whole incircle business seemed rather artificial. Besides, it wasn’t that difficult.'' | ||
Revision as of 15:46, 2 September 2006
Problem 1
Problem 2
In triangle 
, 
, let the incircle touch 
, 
, and 
at 
, 
, and 
respectively. Let 
be a point on 
on the opposite
side of 
from . If 
and meet at 
, and 
and 
meet
at 
, prove that 
, , and concur.
Reason: The whole incircle business seemed rather artificial. Besides, it wasn’t that difficult.
