Difference between revisions of "2019 IMO Problems/Problem 6"
(→Solution) |
|||
| (2 intermediate revisions by one other user not shown) | |||
| Line 6: | Line 6: | ||
[[File:2019 6 s1.png|450px|right]] | [[File:2019 6 s1.png|450px|right]] | ||
[[File:2019 6 s2.png|450px|right]] | [[File:2019 6 s2.png|450px|right]] | ||
| + | [[File:2019 6 s3.png|390px|right]] | ||
| + | [[File:2019 6 s4.png|390px|right]] | ||
<i><b>Step 1</b></i> | <i><b>Step 1</b></i> | ||
| Line 36: | Line 38: | ||
<math>\angle BQC = \angle BQP + \angle PQC = \angle BFP + \angle CEP =</math> | <math>\angle BQC = \angle BQP + \angle PQC = \angle BFP + \angle CEP =</math> | ||
| − | <math>=\angle BFE – \angle EFP + \angle CEF – \angle FEP = 90^\circ + \alpha + 90^\circ + \alpha – (90^\circ + \alpha) = </math> | + | <math>=\angle BFE – \angle EFP + \angle CEF – \angle FEP =</math> |
| + | <math>= 90^\circ + \alpha + 90^\circ + \alpha – (90^\circ + \alpha) = </math> | ||
<math>90^\circ + \alpha = \angle BIC \implies</math> | <math>90^\circ + \alpha = \angle BIC \implies</math> | ||
| Line 42: | Line 45: | ||
<i><b>Step 3</b></i> | <i><b>Step 3</b></i> | ||
| − | + | ||
We perform inversion around <math>\omega.</math> The straight line <math>PST</math> maps onto circle <math>PITS.</math> We denote this circle <math>\Omega.</math> We prove that the midpoint of <math>AD</math> lies on the circle <math>\Omega.</math> | We perform inversion around <math>\omega.</math> The straight line <math>PST</math> maps onto circle <math>PITS.</math> We denote this circle <math>\Omega.</math> We prove that the midpoint of <math>AD</math> lies on the circle <math>\Omega.</math> | ||
| Line 61: | Line 64: | ||
<i><b>Step 4</b></i> | <i><b>Step 4</b></i> | ||
| − | + | ||
| + | We prove that image of <math>Q</math> lies on <math>\Omega.</math> | ||
| + | |||
| + | In the inversion plane the image of point <math>Q</math> lies on straight line <math>BC</math> (It is image of circle <math>BIC)</math> and on circle <math>PCE.</math> | ||
| + | |||
| + | <cmath>\angle PQM = \angle PQC = \angle PEC = \angle PED = \angle PSD = \angle PSM \implies</cmath> point <math>Q</math> lies on <math>\Omega</math>. | ||
| + | |||
| + | '''vladimir.shelomovskii@gmail.com, vvsss''' | ||
| + | |||
| + | ==See Also== | ||
| + | |||
| + | {{IMO box|year=2019|num-b=5|after=Last Problem}} | ||
Latest revision as of 00:52, 19 November 2023
Problem
Let ![]() be the incenter of acute triangle
be the incenter of acute triangle ![]() with
with ![]() . The incircle
. The incircle ![]() of
of ![]() is tangent to sides
is tangent to sides ![]() ,
, ![]() , and
, and ![]() at
at ![]() ,
, ![]() , and
, and ![]() , respectively. The line through
, respectively. The line through ![]() perpendicular to
perpendicular to ![]() meets
meets ![]() again at
again at ![]() . Line
. Line ![]() meets ω again at
meets ω again at ![]() . The circumcircles of triangles
. The circumcircles of triangles ![]() and
and ![]() meet again at
meet again at ![]() .
Prove that lines
.
Prove that lines ![]() and
and ![]() meet on the line through
meet on the line through ![]() perpendicular to
perpendicular to ![]() .
.
Solution
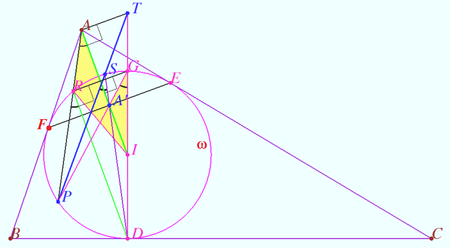
Step 1
We find an auxiliary point ![]()
Let ![]() be the antipode of
be the antipode of ![]() on
on ![]() where
where ![]() is radius
is radius ![]()
We define ![]()
![]() is cyclic
is cyclic ![]()
![]()
![]() An inversion with respect
An inversion with respect ![]() swap
swap ![]() and
and ![]() is the midpoint
is the midpoint ![]()
Let ![]() meets
meets ![]() again at
again at ![]() We define
We define ![]()
Opposite sides of any quadrilateral inscribed in the circle ![]() meet on the polar line of the intersection of the diagonals with respect to
meet on the polar line of the intersection of the diagonals with respect to ![]() and
and ![]() meet on the line through
meet on the line through ![]() perpendicular to
perpendicular to ![]() The problem is reduced to proving that
The problem is reduced to proving that ![]()
Step 2
We find a simplified way to define the point ![]()
We define ![]()
![]()
![]() and
and ![]() are bisectrices).
are bisectrices).
We use the Tangent-Chord Theorem and get ![]()
![]()
![]()
![]()
![]()
Points ![]() and
and ![]() are concyclic.
are concyclic.
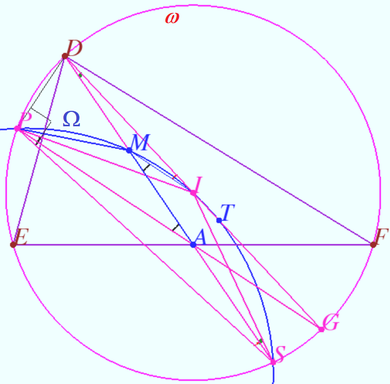
Step 3
We perform inversion around ![]() The straight line
The straight line ![]() maps onto circle
maps onto circle ![]() We denote this circle
We denote this circle ![]() We prove that the midpoint of
We prove that the midpoint of ![]() lies on the circle
lies on the circle ![]()
In the diagram, the configuration under study is transformed using inversion with respect to ![]() The images of the points are labeled in the same way as the points themselves. Points
The images of the points are labeled in the same way as the points themselves. Points ![]() and
and ![]() have saved their position. Vertices
have saved their position. Vertices ![]() and
and ![]() have moved to the midpoints of the segments
have moved to the midpoints of the segments ![]() and
and ![]() respectively.
respectively.
Let ![]() be the midpoint
be the midpoint ![]()
We define ![]()
![]()
![]()
![]() is triangle
is triangle ![]() midline
midline ![]()
![]()
![]()
![]()
![]() point
point ![]() lies on
lies on ![]()
![]() is parallelogram
is parallelogram ![]() is midpoint
is midpoint ![]()
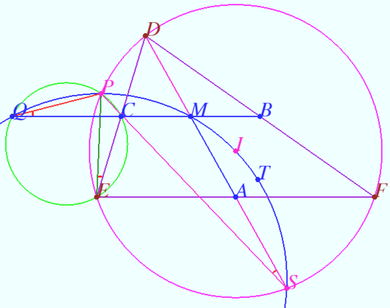
Step 4
We prove that image of ![]() lies on
lies on ![]()
In the inversion plane the image of point ![]() lies on straight line
lies on straight line ![]() (It is image of circle
(It is image of circle ![]() and on circle
and on circle ![]()
![]() point
point ![]() lies on
lies on ![]() .
.
vladimir.shelomovskii@gmail.com, vvsss
See Also
| 2019 IMO (Problems) • Resources | ||
| Preceded by Problem 5 |
1 • 2 • 3 • 4 • 5 • 6 | Followed by Last Problem |
| All IMO Problems and Solutions | ||