Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "2004 AMC 12A Problems/Problem 12"

(sol, {{image}})
 
(→‎See also)
 
(One intermediate revision by one other user not shown)
Line 5: Line 5:
  
 
== Solution ==
 
== Solution ==
−
{{image}}
+
[[Image:2004_AMC_12A-12.png]]
  
 
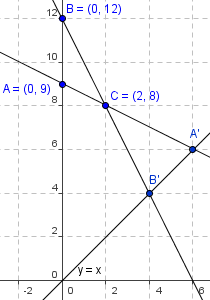
The [[equation]] of <math>\overline{AA'}</math> can be found using points <math>A, C</math> to be <math>y - 9 = \left(\frac{9-8}{0-2}\right)(x - 0) \Longrightarrow y = -\frac{1}{2}x + 9</math>. Similarily, <math>\overline{BB'}</math> has the equation <math>y - 12 = \left(\frac{12-8}{0-2}\right)(x-0) \Longrightarrow y = -2x + 12</math>. These two equations intersect the line <math>y=x</math> at <math>(6,6)</math> and <math>(4,4)</math>. Using the [[distance formula]] or <math>45-45-90</math> [[right triangle]]s, the answer is <math>2\sqrt{2}\ \mathrm{(B)}</math>.  
 
The [[equation]] of <math>\overline{AA'}</math> can be found using points <math>A, C</math> to be <math>y - 9 = \left(\frac{9-8}{0-2}\right)(x - 0) \Longrightarrow y = -\frac{1}{2}x + 9</math>. Similarily, <math>\overline{BB'}</math> has the equation <math>y - 12 = \left(\frac{12-8}{0-2}\right)(x-0) \Longrightarrow y = -2x + 12</math>. These two equations intersect the line <math>y=x</math> at <math>(6,6)</math> and <math>(4,4)</math>. Using the [[distance formula]] or <math>45-45-90</math> [[right triangle]]s, the answer is <math>2\sqrt{2}\ \mathrm{(B)}</math>.  
Line 13: Line 13:
  
 
[[Category:Introductory Geometry Problems]]
 
[[Category:Introductory Geometry Problems]]
 +
{{MAA Notice}}

Latest revision as of 19:17, 3 July 2013

Problem

Let $A = (0,9)$ and $B = (0,12)$. Points $A'$ and $B'$ are on the line $y = x$, and $\overline{AA'}$ and $\overline{BB'}$ intersect at $C = (2,8)$. What is the length of $\overline{A'B'}$?

$\text {(A)} 2 \qquad \text {(B)} 2\sqrt2 \qquad \text {(C)} 3 \qquad \text {(D)} 2 + \sqrt 2\qquad \text {(E)}3\sqrt 2$

Solution

2004 AMC 12A-12.png

The equation of $\overline{AA'}$ can be found using points $A, C$ to be $y - 9 = \left(\frac{9-8}{0-2}\right)(x - 0) \Longrightarrow y = -\frac{1}{2}x + 9$. Similarily, $\overline{BB'}$ has the equation $y - 12 = \left(\frac{12-8}{0-2}\right)(x-0) \Longrightarrow y = -2x + 12$. These two equations intersect the line $y=x$ at $(6,6)$ and $(4,4)$. Using the distance formula or $45-45-90$ right triangles, the answer is $2\sqrt{2}\ \mathrm{(B)}$.

See also

2004 AMC 12A (Problems • Answer Key • Resources)
Preceded by
Problem 11 		Followed by
Problem 13
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25
All AMC 12 Problems and Solutions

The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. AMC logo.png

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=2004_AMC_12A_Problems/Problem_12&oldid=53534"