Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "1971 Canadian MO Problems"

(→‎Problem 6)
m (it ain't no empty page)
 
(4 intermediate revisions by one other user not shown)
Line 1: Line 1:
−
{{empty}}
 
−
 
 
== Problem 1 ==
 
== Problem 1 ==
 
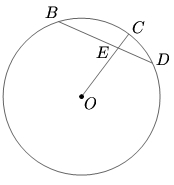
<math>DEB</math> is a chord of a circle such that <math>DE=3</math> and <math>EB=5 .</math> Let <math>O</math> be the center of the circle. Join <math>OE</math> and extend <math>OE</math> to cut the circle at <math>C.</math> Given <math>EC=1,</math> find the radius of the circle
 
<math>DEB</math> is a chord of a circle such that <math>DE=3</math> and <math>EB=5 .</math> Let <math>O</math> be the center of the circle. Join <math>OE</math> and extend <math>OE</math> to cut the circle at <math>C.</math> Given <math>EC=1,</math> find the radius of the circle
Line 29: Line 27:
 
== Problem 5 ==
 
== Problem 5 ==
  
−
 
+
Let <math>p(x) = a_nx^n + a_{n-1}x^{n-1} + \cdots + a_1x+a_0</math>, where the coefficients <math> a_i</math> are integers. If <math>p(0)</math> and <math>p(1)</math> are both odd, show that <math>p(x)</math> has no integral roots.
  
  
Line 37: Line 35:
  
 
Show that, for all integers <math>n</math>, <math>n^2+2n+12</math> is not a multiple of 121.  
 
Show that, for all integers <math>n</math>, <math>n^2+2n+12</math> is not a multiple of 121.  
 +
 
[[1971 Canadian MO Problems/Problem 6 | Solution]]
 
[[1971 Canadian MO Problems/Problem 6 | Solution]]
  
 
== Problem 7 ==
 
== Problem 7 ==
  
 +
Let <math>n</math> be a five digit number (whose first digit is non-zero) and let <math>m</math> be the four digit number formed from n by removing its middle digit. Determine all <math>n</math> such that <math>n/m</math> is an integer.
  
 
[[1971 Canadian MO Problems/Problem 7 | Solution]]
 
[[1971 Canadian MO Problems/Problem 7 | Solution]]
 
== Problem 8 ==
 
== Problem 8 ==
  
 +
A regular pentagon is inscribed in a circle of radius <math>r</math>. <math>P</math> is any point inside the pentagon. Perpendiculars are dropped from <math>P</math> to the sides, or the sides produced, of the pentagon.
  
 +
a) Prove that the sum of the lengths of these perpendiculars is constant.
 +
 +
b) Express this constant in terms of the radius <math>r</math>.
  
 
[[1971 Canadian MO Problems/Problem 8 | Solution]]
 
[[1971 Canadian MO Problems/Problem 8 | Solution]]
Line 51: Line 55:
 
== Problem 9 ==
 
== Problem 9 ==
  
 +
Two flag poles of height <math>h</math> and <math>k</math> are situated <math>2a</math> units apart on a level surface. Find the set of all points on the surface which are so situated that the angles of elevation of the tops of the poles are equal.
  
 
[[1971 Canadian MO Problems/Problem 9 | Solution]]
 
[[1971 Canadian MO Problems/Problem 9 | Solution]]
 
== Problem 10 ==
 
== Problem 10 ==
  
−
 
+
Suppose that <math>n</math> people each know exactly one piece of information, and all <math>n</math> pieces are different. Every time person <math>A</math> phones person <math>B</math>, <math>A</math> tells <math>B</math> everything that <math>A</math> knows, while <math>B</math> tells <math>A</math> nothing. What is the minimum number of phone calls between pairs of people needed for everyone to know everything? Prove your answer is a minimum.
  
 
[[1971 Canadian MO Problems/Problem 10 | Solution]]
 
[[1971 Canadian MO Problems/Problem 10 | Solution]]

Latest revision as of 07:58, 13 September 2012

Problem 1

$DEB$ is a chord of a circle such that $DE=3$ and $EB=5 .$ Let $O$ be the center of the circle. Join $OE$ and extend $OE$ to cut the circle at $C.$ Given $EC=1,$ find the radius of the circle

CanadianMO 1971-1.jpg


Solution

Problem 2

Let $x$ and $y$ be positive real numbers such that $x+y=1$. Show that $\left(1+\frac{1}{x}\right)\left(1+\frac{1}{y}\right)\ge 9$.

Solution

Problem 3

$ABCD$ is a quadrilateral with $AD=BC$. If $\angle ADC$ is greater than $\angle BCD$, prove that $AC>BD$.

Solution

Problem 4

Determine all real numbers $a$ such that the two polynomials $x^2+ax+1$ and $x^2+x+a$ have at least one root in common.


Solution

Problem 5

Let $p(x) = a_nx^n + a_{n-1}x^{n-1} + \cdots + a_1x+a_0$, where the coefficients $a_i$ are integers. If $p(0)$ and $p(1)$ are both odd, show that $p(x)$ has no integral roots.


Solution

Problem 6

Show that, for all integers $n$, $n^2+2n+12$ is not a multiple of 121.

Solution

Problem 7

Let $n$ be a five digit number (whose first digit is non-zero) and let $m$ be the four digit number formed from n by removing its middle digit. Determine all $n$ such that $n/m$ is an integer.

Solution

Problem 8

A regular pentagon is inscribed in a circle of radius $r$. $P$ is any point inside the pentagon. Perpendiculars are dropped from $P$ to the sides, or the sides produced, of the pentagon.

a) Prove that the sum of the lengths of these perpendiculars is constant.

b) Express this constant in terms of the radius $r$.

Solution

Problem 9

Two flag poles of height $h$ and $k$ are situated $2a$ units apart on a level surface. Find the set of all points on the surface which are so situated that the angles of elevation of the tops of the poles are equal.

Solution

Problem 10

Suppose that $n$ people each know exactly one piece of information, and all $n$ pieces are different. Every time person $A$ phones person $B$, $A$ tells $B$ everything that $A$ knows, while $B$ tells $A$ nothing. What is the minimum number of phone calls between pairs of people needed for everyone to know everything? Prove your answer is a minimum.

Solution

Resources

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=1971_Canadian_MO_Problems&oldid=48279"