Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "1987 AIME Problems/Problem 15"
Line 15: Line 15:
 
== Easy Trig Solution ==
 
== Easy Trig Solution ==
  
−
Let <math>\tan\angle ABC = x</math>. Now using the 1st square, <math>AC=21(1+x)</math> and <math>CB=21(1+x^{-1})</math>. Using the second square, <math>AB=\sqrt{440}(1+x+x^{-1})</math>. We have <math>AC^2+CB^2=AB^2</math>, or <cmath>441(x^2+x^{-2}+2x+2x^{-1}+2)=440(x^2+x^{-2}+2x+2x^{-1}+3).</cmath> Rearranging and letting <math>u=x+x^{-1} \Rightarrow u^2 - 2 = x^2 + x^{-2}</math> gives <math>u^2+2u-440=0.</math> Taking the positive root gives <math>u=20</math>, so <math>AC+CB=21(2+x+x^{-1})=21(2+u)=\boxed{462}</math>.
+
Let <math>\tan\angle ABC = x</math>. Now using the 1st square, <math>AC=21(1+x)</math> and <math>CB=21(1+x^{-1})</math>. Using the second square, <math>AB=\sqrt{440}(1+x+x^{-1})</math>. We have <math>AC^2+CB^2=AB^2</math>, or <cmath>441(x^2+x^{-2}+2x+2x^{-1}+2)=440(x^2+x^{-2}+2x+2x^{-1}+3).</cmath> Rearranging and letting <math>u=x+x^{-1} \Rightarrow u^2 - 2 = x^2 + x^{-2}</math> gives us <math>u^2+2u-440=0.</math> We take the positive root, so <math>u=20</math>, which means <math>AC+CB=21(2+x+x^{-1})=21(2+u)=\boxed{462}</math>.
  
 
== See also ==
 
== See also ==

Revision as of 05:06, 26 February 2018

Problem

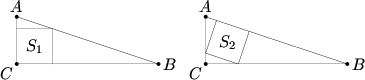
Squares $S_1$ and $S_2$ are inscribed in right triangle $ABC$, as shown in the figures below. Find $AC + CB$ if area $(S_1) = 441$ and area $(S_2) = 440$.

AIME 1987 Problem 15.png

Solution

1987 AIME-15a.png

Because all the triangles in the figure are similar to triangle $ABC$, it's a good idea to use area ratios. In the diagram above, $\frac {T_1}{T_3} = \frac {T_2}{T_4} = \frac {441}{440}.$ Hence, $T_3 = \frac {440}{441}T_1$ and $T_4 = \frac {440}{441}T_2$. Additionally, the area of triangle $ABC$ is equal to both $T_1 + T_2 + 441$ and $T_3 + T_4 + T_5 + 440.$

Setting the equations equal and solving for $T_5$, $T_5 = 1 + T_1 - T_3 + T_2 - T_4 = 1 + \frac {T_1}{441} + \frac {T_2}{441}$. Therefore, $441T_5 = 441 + T_1 + T_2$. However, $441 + T_1 + T_2$ is equal to the area of triangle $ABC$! This means that the ratio between the areas $T_5$ and $ABC$ is $441$, and the ratio between the sides is $\sqrt {441} = 21$. As a result, $AB = 21\sqrt {440} = \sqrt {AC^2 + BC^2}$. We now need $(AC)(BC)$ to find the value of $AC + BC$, because $AB^2 + BC^2 + 2(AC)(BC) = (AC + BC)^2$.

Let $h$ denote the height to the hypotenuse of triangle $ABC$. Notice that $h - \frac {1}{21}h = \sqrt {440}$. (The height of $ABC$ decreased by the corresponding height of $T_5$) Thus, $(AB)(h) = (AC)(BC) = 22\cdot 21^2$. Because $AB^2 + BC^2 + 2(AC)(BC) = (AC + BC)^2 = 21^2\cdot22^2$, $AC + BC = (21)(22) = \boxed{462}$.

Easy Trig Solution

Let $\tan\angle ABC = x$. Now using the 1st square, $AC=21(1+x)$ and $CB=21(1+x^{-1})$. Using the second square, $AB=\sqrt{440}(1+x+x^{-1})$. We have $AC^2+CB^2=AB^2$, or \[441(x^2+x^{-2}+2x+2x^{-1}+2)=440(x^2+x^{-2}+2x+2x^{-1}+3).\] Rearranging and letting $u=x+x^{-1} \Rightarrow u^2 - 2 = x^2 + x^{-2}$ gives us $u^2+2u-440=0.$ We take the positive root, so $u=20$, which means $AC+CB=21(2+x+x^{-1})=21(2+u)=\boxed{462}$.

See also

1987 AIME (Problems • Answer Key • Resources)
Preceded by
Problem 14 		Followed by
Last
Question
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15
All AIME Problems and Solutions

The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. AMC logo.png

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=1987_AIME_Problems/Problem_15&oldid=92382"