Difference between revisions of "1971 Canadian MO Problems/Problem 1"
m |
(→Solution) |
||
| Line 6: | Line 6: | ||
== Solution == | == Solution == | ||
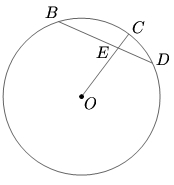
First, extend <math>CO</math> to meet the circle at <math>P.</math> Let the radius be <math>r.</math> Applying [[power of a point]], | First, extend <math>CO</math> to meet the circle at <math>P.</math> Let the radius be <math>r.</math> Applying [[power of a point]], | ||
| − | <math>( | + | <math>(EO)(CE)=(BE)(ED)</math> and <math>2r-1=15.</math> Hence, <math>r=8.</math> |
== See Also == | == See Also == | ||
Revision as of 23:46, 7 November 2018
Problem
![]() is a chord of a circle such that
is a chord of a circle such that ![]() and
and ![]() Let
Let ![]() be the center of the circle. Join
be the center of the circle. Join ![]() and extend
and extend ![]() to cut the circle at
to cut the circle at ![]() Given
Given ![]() find the radius of the circle
find the radius of the circle
Solution
First, extend ![]() to meet the circle at
to meet the circle at ![]() Let the radius be
Let the radius be ![]() Applying power of a point,
Applying power of a point,
![]() and
and ![]() Hence,
Hence, ![]()
See Also
| 1971 Canadian MO (Problems) | ||
| Preceded by First Question |
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • | Followed by Problem 2 |