Polya’s method for extremums
The segment of the shortest length
The segment 
has the ends on the sides of a right angle and contains a point 
Find the shortest length of such a segment.
Solution
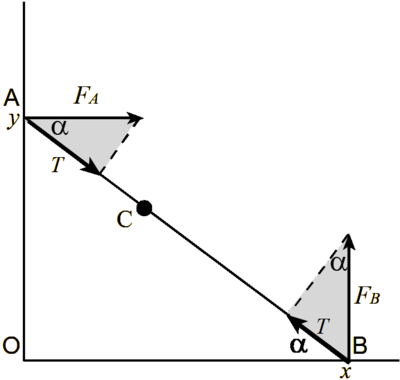
Let's imagine that is a spring rod that cannot bend, but tends to shorten its length.
The rod is fixed at point 
on a hinge without friction. The hinge allows the rod to rotate and slide.
The ends of the rod can slide without friction along the grooves - the sides of the corner.
Let the rod be balanced, and the force pulling it together is equal to 
The grooves can create a force only along the normal, so they act on the rod with forces
![\[F_B = \frac {T}{\sin \alpha}, F_A = \frac {T}{\cos \alpha}.\]](http://latex.artofproblemsolving.com/5/6/b/56b6720ecda59814d758a3e5ffe509d59e5631f3.png)
For the rod to be balanced, it is required that the moments of the forces be equal relative to point 
The moments of forces are:
![\[F_A \cdot AC \cdot \sin \alpha = F_B \cdot BC \cdot \cos \alpha \implies \frac {BC}{AC} = \tan^2 \alpha,\]](http://latex.artofproblemsolving.com/2/a/1/2a134280a47ee7546c9007d8351005418027170a.png)
![\[\frac {y_C}{x_C} = \frac {BC \cdot \sin \alpha}{AC \cdot \cos \alpha} = \tan^3 \alpha \implies\]](http://latex.artofproblemsolving.com/4/5/8/458ac75eb7d406401551578764f66df5453f2ad6.png)
![\[AB = \left ( x_C^ {\frac {2}{3}} + y_C^ {\frac {2}{3}}\right ) ^{\frac {3}{2}}.\]](http://latex.artofproblemsolving.com/a/2/d/a2da672330476d2268ef1a12a2e7638bb8ab7f96.png)
