Difference between revisions of "1987 AIME Problems/Problem 15"
(solution by 4everwise, img needed) |
(→Solution) |
||
| Line 10: | Line 10: | ||
Setting the equations equal and solving for <math>T_5</math>, <math>T_5 = 1 + T_1 - T_3 + T_2 - T_4 = 1 + \frac {T_1}{441} + \frac {T_2}{441}</math>. Therefore, <math>441T_5 = 441 + T_1 + T_2</math>. However, <math>441 + T_1 + T_2</math> is equal to the area of triangle <math>ABC</math>! This means that the ratio between the areas <math>T_5</math> and <math>ABC</math> is <math>441</math>, and the ratio between the sides is <math>\sqrt {441} = 21</math>. As a result, <math>AB = 21\sqrt {440} = \sqrt {AC^2 + BC^2}</math>. We now need <math>(AC)(BC)</math> to find the value of <math>AC + BC</math>, because <math>AB^2 + 2(AC)(BC) = (AC + BC)^2</math>. | Setting the equations equal and solving for <math>T_5</math>, <math>T_5 = 1 + T_1 - T_3 + T_2 - T_4 = 1 + \frac {T_1}{441} + \frac {T_2}{441}</math>. Therefore, <math>441T_5 = 441 + T_1 + T_2</math>. However, <math>441 + T_1 + T_2</math> is equal to the area of triangle <math>ABC</math>! This means that the ratio between the areas <math>T_5</math> and <math>ABC</math> is <math>441</math>, and the ratio between the sides is <math>\sqrt {441} = 21</math>. As a result, <math>AB = 21\sqrt {440} = \sqrt {AC^2 + BC^2}</math>. We now need <math>(AC)(BC)</math> to find the value of <math>AC + BC</math>, because <math>AB^2 + 2(AC)(BC) = (AC + BC)^2</math>. | ||
| − | Let <math>h</math> denote the height to the [[hypotenuse]] of triangle <math>ABC</math>. Notice that <math>h - \frac {1}{21}h = \sqrt {440}</math>. (The height of <math>ABC</math> decreased by the corresponding height of <math>T_5</math>) Thus, <math>(AB)(h) = (AC)(BC) = 22\cdot 21^2</math>. Because <math>AB^2 + 2(AB)(BC) = (AC + BC)^2 = 21^2\cdot22^2</math>, <math>AC + BC = (21)(22) = 462</math>. | + | Let <math>h</math> denote the height to the [[hypotenuse]] of triangle <math>ABC</math>. Notice that <math>h - \frac {1}{21}h = \sqrt {440}</math>. (The height of <math>ABC</math> decreased by the corresponding height of <math>T_5</math>) Thus, <math>(AB)(h) = (AC)(BC) = 22\cdot 21^2</math>. Because <math>AB^2 + 2(AB)(BC) = (AC + BC)^2 = 21^2\cdot22^2</math>, <math>AC + BC = (21)(22) = 462</math>. |
== See also == | == See also == | ||
Revision as of 10:38, 10 October 2007
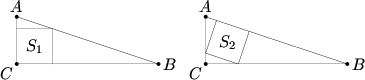
Problem
Squares ![]() and
and ![]() are inscribed in right triangle
are inscribed in right triangle ![]() , as shown in the figures below. Find
, as shown in the figures below. Find ![]() if area
if area ![]() and area
and area ![]() .
.
Solution
An image is supposed to go here. You can help us out by creating one and editing it in. Thanks.
Because all the triangles in the figure are similar to triangle ![]() , it's a good idea to use area ratios. In the diagram above,
, it's a good idea to use area ratios. In the diagram above, ![]() Hence,
Hence, ![]() and
and ![]() . Additionally, the area of triangle
. Additionally, the area of triangle ![]() is equal to both
is equal to both ![]() and
and ![]()
Setting the equations equal and solving for ![]() ,
, ![]() . Therefore,
. Therefore, ![]() . However,
. However, ![]() is equal to the area of triangle
is equal to the area of triangle ![]() ! This means that the ratio between the areas
! This means that the ratio between the areas ![]() and
and ![]() is
is ![]() , and the ratio between the sides is
, and the ratio between the sides is ![]() . As a result,
. As a result, ![]() . We now need
. We now need ![]() to find the value of
to find the value of ![]() , because
, because ![]() .
.
Let ![]() denote the height to the hypotenuse of triangle
denote the height to the hypotenuse of triangle ![]() . Notice that
. Notice that ![]() . (The height of
. (The height of ![]() decreased by the corresponding height of
decreased by the corresponding height of ![]() ) Thus,
) Thus, ![]() . Because
. Because ![]() ,
, ![]() .
.
See also
| 1987 AIME (Problems • Answer Key • Resources) | ||
| Preceded by Problem 14 |
Followed by Last Question | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 | ||
| All AIME Problems and Solutions | ||