Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "1998 AIME Problems/Problem 3"

 
(prob/sol)
Line 1: Line 1:
 
== Problem ==
 
== Problem ==
 +
The graph of <math> y^2 + 2xy + 40|x| \displaystyle = 400</math> partitions the plane into several regions.  What is the area of the bounded region?
  
 
== Solution ==
 
== Solution ==
 +
:<math>\displaystyle 40|x| = - y^2 - 2xy + 400</math>
 +
 +
We can split the equation into a piecewise equation by breaking up the [[absolute value]]:
 +
 +
:<math>40x = -y^2 - 2xy + 400 \displaystyle \quad \displaystyle \quad x\ge 0 </math>
 +
:<math>\displaystyle 40x = y^2 + 2xy - 400 \quad \quad x < 0</math>
 +
 +
Factoring the first one: (alternatively, it is also possible to [[completing the square|complete the square]])
 +
 +
:<math>\displaystyle 40x + 2xy +  = -y^2 + 400</math>
 +
:<math> 2x(20 + y)\displaystyle =  (20 - y)(20 + y)</math>
 +
 +
[[Image:AIME_1998-3.png|right|thumb|300px]]
 +
 +
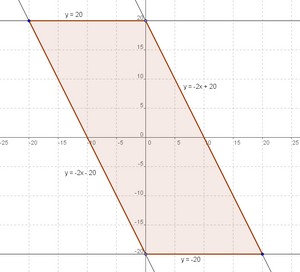
Hence, either <math>\displaystyle y = -20</math>, or <math>\displaystyle 2x = 20 - y \Longrightarrow y = -2x + 20</math>.
 +
 +
Similarily, for the second one, we get <math>\displaystyle y = 20</math> or <math> y = -2x - 20 \displaystyle</math>. If we graph these four equations, we see that we get a parallelogram with base 20 and height 40. Hence the answer is <math>\displaystyle 800</math>.
  
 
== See also ==
 
== See also ==
−
* [[1998 AIME Problems]]
+
{{AIME box|year=1998|num-b=2|num-a=4}}
 +
 
 +
[[Category:Intermediate Algebra Problems]]
 +
[[Category:Intermediate Geometry Problems]]

Revision as of 17:58, 7 September 2007

Problem

The graph of $y^2 + 2xy + 40|x| \displaystyle = 400$ partitions the plane into several regions. What is the area of the bounded region?

Solution

$\displaystyle 40|x| = - y^2 - 2xy + 400$

We can split the equation into a piecewise equation by breaking up the absolute value:

$40x = -y^2 - 2xy + 400 \displaystyle \quad \displaystyle \quad x\ge 0$
$\displaystyle 40x = y^2 + 2xy - 400 \quad \quad x < 0$

Factoring the first one: (alternatively, it is also possible to complete the square)

$\displaystyle 40x + 2xy + = -y^2 + 400$
$2x(20 + y)\displaystyle = (20 - y)(20 + y)$
AIME 1998-3.png

Hence, either $\displaystyle y = -20$, or $\displaystyle 2x = 20 - y \Longrightarrow y = -2x + 20$.

Similarily, for the second one, we get $\displaystyle y = 20$ or $y = -2x - 20 \displaystyle$. If we graph these four equations, we see that we get a parallelogram with base 20 and height 40. Hence the answer is $\displaystyle 800$.

See also

1998 AIME (Problems • Answer Key • Resources)
Preceded by
Problem 2 		Followed by
Problem 4
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15
All AIME Problems and Solutions
Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=1998_AIME_Problems/Problem_3&oldid=15851"