Difference between revisions of "2007 AIME II Problems/Problem 9"
m (→Solution: + solution 2) |
m (→Solution 2: typo) |
||
| Line 13: | Line 13: | ||
=== Solution 2 === | === Solution 2 === | ||
| − | By the [[Two Tangent theorem]], we have that <math>\displaystyle FY = PQ + QF</math>. Solve for <math>\displaystyle PQ = FY - QF</math>. Also, <math>\displaystyle QF = EP = EX</math>, so <math>\displaystyle PQ = FY - EX</math>. Since <math>\displaystyle BX = BY</math>, this can become <math>\ | + | By the [[Two Tangent theorem]], we have that <math>\displaystyle FY = PQ + QF</math>. Solve for <math>\displaystyle PQ = FY - QF</math>. Also, <math>\displaystyle QF = EP = EX</math>, so <math>\displaystyle PQ = FY - EX</math>. Since <math>\displaystyle BX = BY</math>, this can become <math>\displaystyle PQ = FY - EX + (BY - BX)</math><math> = \left(FY + BY\right) - \left(EX + EY\right) = FB - EB</math>. Substituting in their values, the answer is <math>364 - 105 = 259</math>. |
== See also == | == See also == | ||
Revision as of 09:01, 5 April 2007
Contents
[hide]Problem
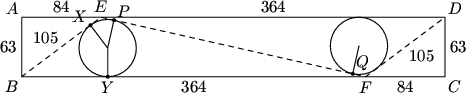
Rectangle ![]() is given with
is given with ![]() and
and ![]() Points
Points ![]() and
and ![]() lie on
lie on ![]() and
and ![]() respectively, such that
respectively, such that ![]() The inscribed circle of triangle
The inscribed circle of triangle ![]() is tangent to
is tangent to ![]() at point
at point ![]() and the inscribed circle of triangle
and the inscribed circle of triangle ![]() is tangent to
is tangent to ![]() at point
at point ![]() Find
Find ![]()
Solution
Solution 1
Several Pythagorean triples exist amongst the numbers given. ![]() . Also, the length of
. Also, the length of ![]() .
.
Use the Two Tangent theorem on ![]() . Since both circles are inscribed in congruent triangles, they are congruent; therefore,
. Since both circles are inscribed in congruent triangles, they are congruent; therefore, ![]() . By the Two Tangent theorem, note that
. By the Two Tangent theorem, note that ![]() , making
, making ![]() . Also,
. Also, ![]() .
. ![]() .
.
Finally, ![]() . Also,
. Also, ![]() . Equating, we see that
. Equating, we see that ![]() , so
, so ![]() .
.
Solution 2
By the Two Tangent theorem, we have that ![]() . Solve for
. Solve for ![]() . Also,
. Also, ![]() , so
, so ![]() . Since
. Since ![]() , this can become
, this can become ![]()
![]() . Substituting in their values, the answer is
. Substituting in their values, the answer is ![]() .
.
See also
| 2007 AIME II (Problems • Answer Key • Resources) | ||
| Preceded by Problem 8 |
Followed by Problem 10 | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 | ||
| All AIME Problems and Solutions | ||