Difference between revisions of "2015 AIME I Problems/Problem 4"
m (→Solution 3) |
|||
| Line 67: | Line 67: | ||
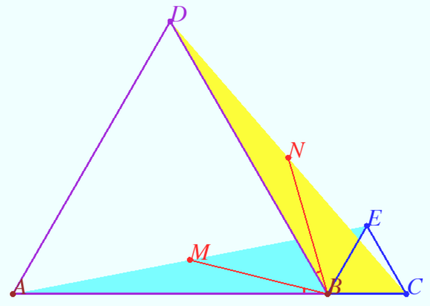
[[File:2015 AIME I 4.png|430px|right]] | [[File:2015 AIME I 4.png|430px|right]] | ||
| − | <math> AB = BD, BE = BC, \angle ABE = \angle CBD \implies \triangle ABE | + | <math> AB = BD, BE = BC, \angle ABE = \angle CBD \implies \triangle ABE \cong \triangle DBC</math> |
Medians are equal, so <math>MB = MN, \angle ABM = \angle DBN \implies</math> | Medians are equal, so <math>MB = MN, \angle ABM = \angle DBN \implies</math> | ||
Revision as of 18:38, 25 September 2022
Contents
[hide]Problem
Point ![]() lies on line segment
lies on line segment ![]() with
with ![]() and
and ![]() . Points
. Points ![]() and
and ![]() lie on the same side of line
lie on the same side of line ![]() forming equilateral triangles
forming equilateral triangles ![]() and
and ![]() . Let
. Let ![]() be the midpoint of
be the midpoint of ![]() , and
, and ![]() be the midpoint of
be the midpoint of ![]() . The area of
. The area of ![]() is
is ![]() . Find
. Find ![]() .
.
Diagram
![[asy] pair A = (0, 0), B = (16, 0), C = (20, 0), D = (8, 8*sqrt(3)), EE = (18, 2*sqrt(3)), M = (9, sqrt(3)), NN = (14, 4*sqrt(3)); draw(A--B--D--cycle); draw(B--C--EE--cycle); draw(A--EE); draw(C--D); draw(B--M--NN--cycle); dot(A); dot(B); dot(C); dot(D); dot(EE); dot(M); dot(NN); label("A", A, SW); label("B", B, S); label("C", C, SE); label("D", D, N); label("E", EE, N); label("M", M, NW); label("N", NN, NE); [/asy]](http://latex.artofproblemsolving.com/2/2/2/222ea4031f51c1923ced2de0bdf1e1f8e161a1cc.png)
Diagram by RedFireTruck (talk) 18:52, 15 February 2021 (EST)
Solution 1
Let ![]() be the origin, so
be the origin, so ![]() and
and ![]() Using equilateral triangle properties tells us that
Using equilateral triangle properties tells us that ![]() and
and ![]() as well. Therefore,
as well. Therefore, ![]() and
and ![]() Applying the Shoelace Theorem to triangle
Applying the Shoelace Theorem to triangle ![]() gives
gives
![]()
so ![]()
Solution 2
Note that ![]() and
and ![]() . Also,
. Also, ![]() . Thus,
. Thus, ![]() by SAS.
by SAS.
From this, it is clear that a ![]() rotation about
rotation about ![]() will map
will map ![]() to
to ![]() .
This rotation also maps
.
This rotation also maps ![]() to
to ![]() . Thus,
. Thus, ![]() and
and ![]() . Thus,
. Thus, ![]() is equilateral.
is equilateral.
Using the Law of Cosines on ![]() ,
,
![]()
![]() Thus,
Thus, ![]() .
.
Using Stewart's Theorem on ![]() ,
,
![]()
![]()
Calculating the area of ![]() ,
,
![]()
![]() Thus,
Thus, ![]() , so
, so ![]() . Our final answer is
. Our final answer is ![]() .
.
Admittedly, this is much more tedious than the coordinate solutions.
I also noticed that there are two more ways of showing that ![]() is equilateral:
is equilateral:
One way is to show that ![]() ,
, ![]() , and
, and ![]() are related by a spiral similarity centered at
are related by a spiral similarity centered at ![]() .
.
The other way is to use the Mean Geometry Theorem. Note that ![]() and
and ![]() are similar and have the same orientation. Note that
are similar and have the same orientation. Note that ![]() is the weighted average of
is the weighted average of ![]() and
and ![]() ,
, ![]() is the weighted average of
is the weighted average of ![]() and
and ![]() , and
, and ![]() is the weighted average of
is the weighted average of ![]() and
and ![]() . The weights are the same for all three averages. (The weights are actually just
. The weights are the same for all three averages. (The weights are actually just ![]() and
and ![]() , so these are also unweighted averages.) Thus, by the Mean Geometry Theorem,
, so these are also unweighted averages.) Thus, by the Mean Geometry Theorem, ![]() is similar to both
is similar to both ![]() and
and ![]() , which means that
, which means that ![]() is equilateral.
is equilateral.
Solution 3
![]()
Medians are equal, so ![]()
![]()
![]() is equilateral triangle.
is equilateral triangle.
The height of ![]() is
is ![]() distance from
distance from ![]() to midpoint
to midpoint ![]() is
is ![]()
![]() is the median of
is the median of ![]()
![]()
The area of ![]()
![]()
vladimir.shelomovskii@gmail.com, vvsss
See Also
| 2015 AIME I (Problems • Answer Key • Resources) | ||
| Preceded by Problem 3 |
Followed by Problem 5 | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 | ||
| All AIME Problems and Solutions | ||
The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. ![]()