Difference between revisions of "Sharygin Olympiads, the best"

Latest revision as of 16:04, 4 November 2024

Igor Fedorovich Sharygin (13/02/1937 - 12/03/2004, Moscow) - Soviet and Russian mathematician and teacher, specialist in elementary geometry, popularizer of science. He wrote many textbooks on geometry and created a number of beautiful problems. He headed the mathematics section of the Russian Soros Olympiads. After his death, Russia annually hosts the Geometry Olympiad for high school students. It consists of two rounds – correspondence and final. The correspondence round lasts 3 months.

The best problems of these Olympiads will be published. The numbering contains the year of the Olympiad and the serial number of the problem. Solutions are often different from the original ones.

2024 tur 2 klass 10 Problem 6

A point $P$ lies on one of medians of triangle $\triangle ABC$ in such a way that $\angle PAB = \angle PBC = \angle PCA.$ Prove that there exists a point $Q$ on another median such that $\angle QBA = \angle QCB = \angle QAC.$ (A.Zaslavsky)

Proof

1. Denote $a = BC, b = AC, c = AB.$ It is known that barycentric coordinates are $P = (a^2 b^2 : b^2 c^2 : c^2 a^2), P \in AD \implies b^2 c^2 = c^2 a^2 \implies AC = BC.$

2. Denote $\omega = \odot APB, \Omega = \odot APC.$

$\angle ABP = \angle PAC \implies AC$ is tangent to $\omega.$

$\angle PAC = \angle PCB \implies BC$ is tangent $\Omega.$

$PC$ is the radical axes of $\omega$ and $\Omega,$ the power of a point $D$ with respect to a circle $\Omega$ is $CD^2$ so the power of a point $D$ with respect to a circle $\omega$ is $CD^2.$

$BD^2 = CD^2,$ so $BD$ is tangent to $\omega \implies \angle ABC = \angle CAB \implies AC = BC.$

$AC = BC,$ so point $Q$ symmetrical to $P$ with respect to the $C-$ median satisfies the conditions. $\blacksquare$

vladimir.shelomovskii@gmail.com, vvsss

2024 tur 2 klass 10 Problem 7

Let $ABC$ be a triangle with $\angle A = 60^\circ, AD, BE,$ and $CF$ be its bisectors, $P, Q$ be the projections of $A$ to $EF$ and $BC$ respectively, and $R$ be the second common point of the circle $\omega = \odot DEF$ with $AD.$

Prove that points $P, Q, R$ are collinear. (K.Belsky)

Proof

Denote $a = BC, b = AC, c = AB, I -$ the incenter of $\triangle ABC,$ $L=FE \cap AD, R' -$ the midpoint of $AI.$

It is known ( Division of bisector) that $\frac {AI}{DI} = \frac {b+c}{a}, \frac {DI}{IL} = 1+ \frac {2a}{b+c} \implies \frac{1}{IL} = \frac{1}{DI} + \frac{2}{AI} \implies$ $2 DI (\frac{AI}{2} - IL) =AI \cdot IL \implies 2 DI \cdot LR' = AI \cdot IL.$

$DL \cdot LR' = (DI + IL) \cdot LR' = \frac {AI \cdot IL}{2} + IL \cdot (\frac{AI}{2} - IL) = AI \cdot IL - IL^2 = AL \cdot IL.$ $\angle BAC = 60^\circ \implies \angle BIC = \angle FIE = 120^\circ \implies AEIF$ is cyclic.

Therefore $AL \cdot IL = LE \cdot LF = DL \cdot LR' \implies EDFR'$ is cyclic $\implies R = R'.$

Let $AG \perp AD, G \in BC, H = AP \cap BC, Q' = RP \cap BC.$

It is known that points $F, E,$ and $G$ are collinear, $\angle AGB = \frac{|\angle ACB - \angle ABC|}{2}, \angle AGE = \angle BGE.$

$AP \perp EF, AQ \perp BG \implies AG$ is the diameter of $\odot APQG \implies$ $\overset{\Large\frown} {QP} = \overset{\Large\frown} {AP} \implies \angle DAP = \angle QAP, AP = PH.$ $AR = RI, AP = PH \implies RP || IH \implies \frac {DH}{HQ'} = \frac {DI}{IR} = \frac {2a}{b+c}.$ $AH$ is the bisector of $\angle DAQ \implies \frac {DH}{HQ} = \frac {AD}{AQ}.$

Bisector $AD = \frac {2 b c \cos 30^\circ}{b+c}.$

Altitude $AQ = \frac {2[ABC]}{BC}= \frac {bc \sin 60^\circ}{a} \implies \frac {AD}{AQ} = \frac {2a}{b+c} = \frac {DH}{HQ} = \frac {DH}{HQ'} \implies Q = Q'. \blacksquare$

Note that the point $R$ is a Feuerbach point of $\triangle ABC$ since both the inscribed circle and the Euler circle pass through it.

vladimir.shelomovskii@gmail.com, vvsss

2024 tur 2 klass 9 Problem 7

Let triangle $\triangle ABC$ and point $P$ on the side $BC$ be given. Let $P'$ be such point on the side $BC$ that $BP = P'C.$ The cross points of segments $AP$ and $AP'$ with the incircle $\omega$ of $\triangle ABC$ form a convex quadrilateral $EFE'F'.$

Find the locus of crosspoints of diagonals $EFE'F'.$ (D.Brodsky)

Solution 1. Particular case of Fixed point .

2. Denote $p_a = \frac{b+c-a}{2}, p_b = \frac{a-b+c}{2}, p_c = \frac{a+b-c}{2}, m = \frac {CP}{BP}, \mu = \frac {PF'}{AF'}.$ $PD = \frac {m p_b - p_c} {m+1}, AD' = p_a \implies$ $x(x+y) = p_a^2, z(z+y) = PD^2, x+y+z = AP, \mu = \frac {z}{x+y}.$ We perform simple transformations and get: $\mu^2 p_a^2 - \mu (AP^2 - p_a^2 - PD^2) + PD^2 = 0.$ We use Stewart's theorem and get: $AP^2 = \frac {AC^2}{1+m} + \frac {m AB^2}{1+m} - \frac {m BC^2}{(1+m)^2} \implies$ $(\mu p_a)^2 - 2 (\mu p_a) \frac {m p_b + p_c}{m+1} + \frac {(m p_b - p_c)^2} {(m+1)^2} = 0.$ $\mu = \frac {(\sqrt{m p_b} \pm \sqrt{p_c})^2}{(m+1) p_a}.$ Similarly $\nu = \frac {\eta + \zeta}{\xi}= \frac {(\sqrt{m p_c} \pm \sqrt{p_b})^2}{(m+1) p_a}.$ Therefore $\frac {\nu + \mu}{2} = \frac {a}{2 p_a}$ not depends from $m.$

Let $M$ be the midpoint of $BC, AM$ is the median of $\triangle ABC$ and $\triangle PAP'.$

The line $FF'$ cross the median of $\triangle PAP'$ at point $G'$ such that $\frac {MG'}{G'A} = \frac {\nu + \mu}{2} = \frac {p_b + p_c}{2 p_a} = \frac {a}{2 p_a}.$

So point $G'$ is fixed and this point lyes on $EE' \implies G = G'$ .

Therefore the locus of crosspoints of diagonals $EFE'F'$ is point $G.$

Corollary

Let line $MQ||D'D'', Q \in AC$ . Then $\frac {D''Q}{AD''} = \frac {a}{2 p_a} \implies 2 D''Q = BC.$

vladimir.shelomovskii@gmail.com, vvsss

2024 tur 2 klass 9 Problem 5

Let $ABC$ be an isosceles triangle $(AC = BC), O$ be its circumcenter, $H$ be the orthocenter, and $P$ be a point inside the triangle such that $\angle APH = \angle BPO = 90^\circ.$

Prove that $\angle PAC = \angle PBA = \angle PCB.$ (A.Zaslavsky)

Proof

Denote $E -$ the midpoint $BC, M-$ the midpoint $AB, D -$ the foot from $B$ to $\overline{AC}, \alpha = \angle ABC, \omega = \odot ADHM, \Omega = \odot BEOM, \Theta = \odot BHC, \theta = \odot AB$ tangent to $\overline{BC}.$

$AH = BH, BO = CO , \angle BAH = 90^\circ - \alpha = \angle BCO \implies \triangle ABH \sim \triangle BCO.$ There is a spiral similarity $T$ centered at point $X$ that maps $\triangle ABH$ into $\triangle BCO.$

The coefficient of similarity $k = \frac {BC}{AB} = \frac {1}{2 \cos \alpha},$ rotation angle equal $180^\circ - \alpha.$ $B = T(A), B = AB \cap BC \implies X \in \theta.$ $C = T(B), B = AB \cap BC \implies X \in \Theta$ $(\angle BCH = \angle ABH,$ so $AB$ is tangent to $\Theta).$ Basic information $E = T(M), B = AM \cap BE \implies X \in \Omega.$ $AB = T(CA), CO = T(BH), D = AC \cap BH, M = AB \cap CO \implies M = T(D).$ $A = AD \cap BM \implies X \in \omega.$ $P = \theta \cap \Theta \implies P = X.$ $P = T(P) \implies \triangle BPC = T(\triangle APB) \implies \triangle BPC \sim \triangle APB \implies \angle PAB = \angle PCB.$ $\triangle OBP = T(\triangle HAP) \implies \triangle OBP \sim \triangle HAP \implies \angle OBP = \angle HAP .$ $\angle PAC = \alpha - \angle HAP - \angle BAH = \alpha - \angle OBP - \angle OBC = \angle PBA.\blacksquare$ $\triangle ADM \sim \triangle ABC \implies \angle ADM = \alpha = \angle APM, \angle EPM = 180^\circ - \alpha \implies$ Points $A,P,$ and $E$ are collinear, so $P \in A-$ median of $\triangle ABC.$

$\angle PAB = \angle PCB, \angle ABC = \angle BAC \implies PC$ is $B-$ symmedian of $\triangle ABC.$

$\angle APH = 90^\circ \implies P$ is $A-$ Humpty point.

vladimir.shelomovskii@gmail.com, vvsss

2024 tur 2 klass 9 Problem 4

For which $n > 0$ it is possible to mark several different points and several different circles on the plane in such a way that:

- exactly $n$ marked circles pass through each marked point;

- exactly $n$ marked points lie on each marked circle;

- the center of each marked circle is marked? (P.Puchkov)

Solution

Case $n = 1.$ Circles centered at $A$ and $B$ with radii $R = |\vec {AB}|.$

Case $n = 2, \vec {AC} = \vec {BC'}, |\vec {AB} = \vec {AC}|, \vec {AC}$ is not paralel to $\vec {AB}.$

Four circles are centered at points $A, B, C,$ and $C'.$ Each radius is equal $R.$

Case $n = 3, \vec {CD} = \vec {AD''} = \vec {C'D'} = \vec {BD'''}, \vec {CD}$ is not paralel to $\vec {AB}$ or $\vec {AC}, |\vec {AB}| = \vec {CD}|$

Eight circles centered at $A, B, C, C', D, D',D''$ and $D'''$ have radii $R.$

Case $n = 4, \vec {DE} = \vec {D'E'} = ...,|\vec {DE}| = R,..$

Answer For all $n.$

vladimir.shelomovskii@gmail.com, vvsss

2024 tur 2 klass 9 Problem 3

Let $(P,P')$ and $(Q,Q')$ be two pairs of points isogonally conjugated with respect to a triangle $ABC,$ and $R$ be the common point of lines $PQ$ and $P'Q'.$ Prove that the pedal circles of points $P,Q,$ and $R$ are coaxial. (L.Shatunov, V.Shelomovskii)

Solution

1. Let $P'$ be the isogonal conjugate of a point $P$ with respect to a triangle $\triangle ABC.$ Then circle centered at the midpoint $PP'$ is the common pedal circle of points $P$ and $P'.$ ( Circumcircle of pedal triangles) So center $O$ is the midpoint $PP'$ and center $U$ is the midpoint $QQ'.$

2. Denote $R' = PQ' \cap P'Q.$ Then $R'$ is the isogonal conjugate of a point $R$ with respect to $\triangle ABC.$ So center $V$ is the midpoint $RR'.$ ( Two pares of isogonally conjugate points)

3. The Gauss line (or Gauss–Newton line) is the line joining the midpoints of the three diagonals of a complete quadrilateral $PQ'P'Q$ (Gauss line).So points $U,O,$ and $V$ are collinear as was to be proven. $\blacksquare$

vladimir.shelomovskii@gmail.com, vvsss

2024 tur 2 klass 8 Problem 4

A square with sidelength $1$ is cut from the paper. Construct a segment with length $\frac{1}{2024}$ using at most $13$ folds. No instruments are available, it is allowed only to fold the paper and to mark the common points of folding lines. (M.Evdokimov)

Solution

Main idea: $\frac{1}{2024} = \frac{1}{4} \cdot \left [ \frac{1}{22} - \frac{1}{23} \right ].$ $\frac {a}{b} = \frac{x}{x+c} \implies x = \frac {a \cdot c}{b - a}.$ $EG = EA - GA = c \cdot \left [ \frac {1}{b/a - 1} - \frac {1}{(b+FB)/a - 1} \right ].$ Let $c = \frac{1}{2}, a = \frac{1}{64}, b = \frac{45}{64}, FB = \frac{2}{64}.$ $EG = \frac{1}{2} \cdot \left [ \frac {1}{45 - 1} - \frac {1}{47 - 1} \right] = \frac{1}{2024}.$ We perform $1$ horizontal fold of the sheet. We get line $AD (AC = \frac{1}{2}).$ We perform

$6$ vertical folds of the sheet. We get $62$ vertical lines at a distance of $\frac{1}{64}$ from each other.

Point $F$ is the lower left corner of the sheet, point $B$ is the lower point of the second vertical line, point $C$ is the lower point of the $47^{th}$ line, point $D$ is the point at the intersection of the horizontal line and the $46^{th}$ vertical line.

Points $E$ and $G$ are at the intersection of the lines $BD$ and $FD$ and the $47^{th}$ vertical line.

vladimir.shelomovskii@gmail.com, vvsss

2024 tur 2 klass 8 Problem 2

Let $M$ be the midpoint of side $AB$ of an acute-angled triangle $ABC,$ and $P$ be the projection of the orthocenter $H$ to the bisector of angle $C.$ Prove that $MP$ bisects the segment $CH.$ (L.Emelyanov)

Solution

Denote $D$ - the midpoint of $CH, A',B',$ and $C'$ the foots of the heights, $\angle A = 2 \alpha, \angle B = 2 \beta, \angle C = 2 \gamma, \omega$ be the Euler circle $A'DB'C'M.$

$\Omega$ is the circle $\odot CA'B'$ with the diameter $CH.$ $HP \perp CP \implies P \in \Omega.$ $AA' \perp BA' \implies A'M = BM \implies \angle BA'M = 2 \beta.$ $\triangle ABC \sim \triangle A'BC' \implies \angle BA'C' = 2 \alpha \implies$ $\angle MA'C' = 2|\alpha - \beta| = \angle MDC'.$ $\angle ACC' = 90^\circ - 2 \alpha, \angle ACP = \gamma,$ $\angle PCC' = |\angle ACC' - \angle ACP| = |90^\circ - 2 \alpha - \gamma| = | \alpha + \beta + \gamma - 2 \alpha - \gamma| = | \alpha - \beta|.$ $PD = CD \implies \angle PDH = 2 \angle PCD = 2|\alpha - \beta|.$ $\angle PDH = \angle MDH \implies$ points $M,P,$ and $D$ are collinear.

vladimir.shelomovskii@gmail.com, vvsss

2024, Problem 23

A point $P$ moves along a circle $\Omega.$ Let $A$ and $B$ be fixed points of $\Omega,$ and $C$ be an arbitrary point inside $\Omega.$

The common external tangents to the circumcircles of triangles $\triangle APC$ and $\triangle BCP$ meet at point $Q.$

Prove that all points $Q$ lie on two fixed lines.

Solution

Denote $A' = AC \cap \Omega, B' = BC \cap \Omega, \omega = \odot APC, \omega' = \odot BPC.$ $\theta = \odot ACB', \theta' = \odot BCA'.$

$O$ is the circumcenter of $\triangle APC, O'$ is the circumcenter of $\triangle BPC.$

Let $K$ and $L$ be the midpoints of the arcs $\overset{\Large\frown}{CB'}$ of $\theta,D = AL \cap \omega.$

Let $K'$ and $L'$ be the midpoints of the arcs $\overset{\Large\frown}{CA'}$ of $\theta', D' = BL' \cap \omega'.$

These points not depends from position of point $P.$

Suppose, $P \in \overset{\Large\frown} {B'ABA'} ($ see diagram). $\angle A'BC = 2 \alpha = \angle B'AC \implies \angle D'BC = \angle DAC = \alpha \implies \angle DOC = \angle D'O'C = 2 \alpha.$ $O'D' = O'C, OC = OD \implies \triangle OCD \sim \triangle O'D'C \implies OC||O'D'.$ Let $F= CD \cup OO' \implies \frac {FO}{FO'} = \frac {OC}{O'D'} \implies Q = F.$

$\angle LCB' = \alpha = \angle B'BL' \implies LC || L'B.$ Similarly, $AL || CL' \implies \triangle DLC \sim \triangle CL'D' \implies \frac {LC}{L'D'} = \frac {DC}{CD'} = \frac {OC}{O'D'}.$

Let $F' = LL' \cap DD' \implies \frac {F'C}{F'D'} = \frac {LC}{L'D'} = \frac {OC}{O'D'}= \frac {FC}{FD'} \implies F' = F.$

Therefore $Q \in LL'.$ Similarly, if $P \in \overset{\Large\frown} {B'A'}$ then $Q \in KK'.$

Claim

Points $D, C,$ and $D'$ are collinear.

Proof

$S$ is the midpoint of arc $\overset{\Large\frown}{A'B'} \implies \angle SAC = \angle SBC.$ Denote $\angle CAP = \alpha, \angle CBP = \beta, \angle SAC = \angle SBC = \varphi.$ $D \in \omega \implies \angle PDC = \alpha, \angle PCD = \pi - \alpha - \varphi.$ $D' \in \omega' \implies \angle PD'C = \beta, \angle PCD' = \pi - \beta - \varphi.$ $S \in \Omega \implies \angle SAP + \angle SBP = \alpha + \beta + 2 \varphi = \pi.$ Therefore $\angle PCD + \angle PCD' = \pi \implies$ points $D, C,$ and $D'$ are collinear.

vladimir.shelomovskii@gmail.com, vvsss

One-to-one mapping of the circle

Let a circle $\Omega,$ two fixed points $A$ and $B$ on it and a point $C$ inside it be given. Then there is a one-to-one mapping of the circle $\Omega$ onto itself, based on the following two theorems.

1. Let a circle $\Omega,$ two fixed points $A$ and $B$ on $\Omega,$ and a point $C$ inside $\Omega$ be given.

Let an arbitrary point $Q \in \Omega$ be given.

Let $A' = AC \cap \Omega, A' \ne A, B' = BC \cap \Omega, B' \ne B. S$ is the midpoint of the arc $A'B', D = A'Q \cap BS, E = B'Q \cap AS.$

Denote $\omega = \odot AEC, \omega' = \odot BCD, P = \omega \cap \omega'.$ Prove that $P \in \Omega.$

2. Let a circle $\Omega,$ two fixed points $A$ and $B$ on $\Omega,$ and a point $C$ inside $\Omega$ be given.

Let an arbitrary point $P \in \Omega$ be given.

Let $A' = AC \cap \Omega, A' \ne A, B' = BC \cap \Omega, B' \ne B. S$ is the midpoint of the arc $A'B'.$

Denote $\omega = \odot ACP, \omega' = \odot BCP, D = BS \cap \omega' , E = \omega \cap AS.$

Denote $Q = A'D \cap B'E.$ Prove that $Q \in \Omega.$

Proof

$1. C = AA' \cap BB', D = A'Q \cap BS, E = B'Q \cap AS \implies$

Points $D,C,E$ are collinear. $\angle APC = \angle SEC, \angle BPC = \angle SDC.$ $\angle APC + \angle BPC + \angle ASC = \angle SEC + \angle SDC + \angle DSE = 180 ^\circ \implies P \in \Omega.$

2. Points $D, C,$ and $E$ are collinear (see Claim in 2024, Problem 23).

We use Pascal's theorem for points $A,B',S,A',B$ and crosspoints $C,D,E$ and get $Q \in \Omega.$

vladimir.shelomovskii@gmail.com, vvsss

2024, Problem 22

A segment $AB$ is given. Let $C$ be an arbitrary point of the perpendicular bisector to $AB,$ $O$ be the point on the circumcircle of $\triangle ABC$ opposite to $C,$ and an ellipse centered at $O$ touche $AB, BC, CA.$

Find the locus of touching points $P$ of the ellipse with the line $BC.$

Solution

Denote $M$ the midpoint $AB, D$ the point on the line $CO, DO = MO, \alpha = \angle CBM, b = OM.$

$\angle CBO = 90^\circ \implies \angle COB = \alpha, MB = b \tan \alpha,$ $CB = \frac {b \sin \alpha}{\cos^2 \alpha}, CO = \frac {b} {\cos^2 \alpha}, CD = b \left (1 + \frac {1} {\cos^2 \alpha} \right ).$ In order to find the ordinate of point $P,$ we perform an affine transformation (compression along axis $AB)$ which will transform the ellipse $MPD$ into a circle with diameter $MD.$ The tangent of the $CP$ maps into the tangent of the $CE, E = \odot CBO \cap \odot MD, PF \perp CO.$ $\angle OEF = \angle ECO \implies OF = OE \sin \angle OEF = OE \sin \angle ECO = b \cos^2 \alpha.$ $CP = \frac {CF}{\sin \alpha} = \frac {b}{\sin \alpha}\left ( \frac {1} {\cos^2 \alpha} - \cos^2 \alpha \right ) = b \sin \alpha \left ( \frac {1}{\cos^2 \alpha } + 1 \right).$ $\frac {CP}{CD} = \sin \alpha , \angle PCD = 90^\circ - \alpha \implies \angle CPD = 90^\circ.$ $BP = CP - CB = b \sin \alpha.$ Denote $Q = AB \cap DP \implies BQ = \frac {BP}{\cos \alpha} = b \tan \alpha = MB.$

So point $Q$ is the fixed point ( $P$ not depends from angle $\alpha, \angle BPQ = 90^\circ ).$

Therefore point $P$ lies on the circle with diameter $BQ$ (except points $B$ and $Q.)$

vladimir.shelomovskii@gmail.com, vvsss

2024, Problem 21

A chord $PQ$ of the circumcircle of a triangle $ABC$ meets the sides $BC, AC$ at points $A', B',$ respectively. The tangents to the circumcircle at $A$ and $B$ meet at point $X,$ and the tangents at points $P$ and $Q$ meets at point $Y.$ The line $XY$ meets $AB$ at point $C'.$

Prove that the lines $AA', BB',$ and $CC'$ concur.

Proof

WLOG, $P \in \overset{\Large\frown} {AC}.$ Denote $\Omega = \odot ABC, Z = BB' \cap AA', D = AQ \cap BP.$

Point $D$ is inside $\Omega.$

We use Pascal’s theorem for quadrilateral $APQB$ and get $D \in XY.$

We use projective transformation which maps $\Omega$ to a circle and that maps the point $D$ to its center.

From this point we use the same letters for the results of mapping. Therefore the segments $AQ$ and $BP$ are the diameters of $\Omega, C'D \in XY || AP \implies C'$ is the midpoint $AB.$

$AB|| PQ \implies AB || B'A' \implies C' \in CZ \implies$

preimage $Z$ lies on preimage $CC'.\blacksquare$

vladimir.shelomovskii@gmail.com, vvsss

2024, Problem 20

Let a triangle $ABC,$ points $D$ and $E \in BD$ be given, $F = AD \cap CE.$ Points $D', E'$ and $F'$ are the isogonal conjugate of the points $D, E,$ and $F,$ respectively, with respect to $\triangle ABC.$

Denote $R$ and $R'$ the circumradii of triangles $\triangle DEF$ and $\triangle D'E'F',$ respectively.

Prove that $\frac {[DEF]}{R} = \frac {[D'E'F']}{R'},$ where $[DEF]$ is the area of $\triangle DEF.$

Proof

Denote $\angle BAC = \alpha, \angle ABC = \beta, \angle ACB = \gamma,$ $\angle EDF = \Theta, \angle E'D'F' = \theta, \angle DEF = \Psi, \angle D'E'F' = \psi,$ $\angle BAD' = \angle CAF = \varphi_A, \angle CBD' = \angle ABD = \varphi_B, \angle BCE' = \angle ACE = \varphi_C.$ It is easy to prove that $\frac {[DEF]}{R} = \frac {[D'E'F']}{R'}$ is equivalent to $DE \cdot \sin \Theta \cdot \sin \Psi = D'E' \cdot \sin \theta \cdot \sin \psi.$ $\Theta = \alpha - \varphi_A + \varphi_B, \theta = \beta - \varphi_B + \varphi_A, \Psi = \beta - \varphi_B + \varphi_C, \psi = \gamma - \varphi_C + \varphi_B \implies$ $\theta = 180^\circ - \gamma - \Theta, \psi = 180^\circ - \alpha - \Psi.$ By applying the law of sines, we get $\frac {BE}{\sin \varphi_C} = \frac {BC}{\sin \Psi}, \frac {BD}{\sin (\alpha - \varphi_A)} = \frac {AB}{\sin \Theta}, \frac {BC}{\sin \alpha} = \frac {AB}{\sin \gamma}.$ $ED = BD - BE \implies DE \cdot \sin \Theta \cdot \sin \Psi = \frac {AB}{\sin \gamma} \left ( \sin \gamma \cdot \sin (\alpha - \varphi_A) \cdot \sin \Psi - \sin \alpha \cdot \sin \varphi_C \cdot \sin \Theta \right )$

$\frac {BE'}{\sin (\gamma - \varphi_C)} = \frac {BC}{\sin \psi}, \frac {BD'}{\sin \varphi_A} = \frac {AB}{\sin \theta}.$ $D'E' = BE' - BD' \implies D'E' \cdot \sin \theta \cdot \sin \psi = \frac {AB}{\sin \gamma} (\sin \alpha \cdot \sin (\gamma - \varphi_C) \cdot \sin \theta - \sin \gamma \cdot \sin \varphi_A \cdot \sin \psi ).$ We need to prove that $\sin \gamma \cdot \sin (\alpha - \varphi_A) \cdot \sin \Psi - \sin \alpha \cdot \sin \varphi_C \cdot \sin \Theta = \sin \alpha \cdot \sin (\gamma - \varphi_C) \cdot \sin \theta - \sin \gamma \cdot \sin \varphi_A \cdot \sin \psi$ We make the transformations: $\sin \alpha \left[ \sin \varphi_C \cdot \sin \Theta + \sin (\gamma - \varphi_C) \cdot \sin (\gamma + \Theta) \right] = \sin \gamma \left[ \sin (\alpha - \varphi_A) \cdot \sin \Psi + \sin \varphi_A \cdot \sin (\alpha + \Psi) \right]$

$\sin \alpha \left[ \sin \varphi_C \cdot \sin \Theta + (\sin \gamma \cos \varphi_C - \cos \gamma \sin \varphi_C) \cdot (\sin \gamma \cos \Theta + \cos \gamma \sin \Theta \right] =$ $= \sin \gamma \left[ \sin \alpha \cos \varphi_A \cdot \sin \Psi - \cos \alpha \sin \varphi_A \cdot \sin \Psi + \sin \varphi_A \cdot \sin \alpha \cos \Psi + \sin \varphi_A \cdot \cos \alpha \sin \Psi \right]$ $\sin \alpha \left[ \sin \varphi_C \cdot \sin \Theta + \sin^2 \gamma \cos \varphi_C \cos \Theta - \cos ^2 \gamma \sin \varphi_C \cdot \sin \Theta + \cos \gamma \sin \Theta + \sin \gamma \cos \gamma (\cos \varphi_C \sin \Theta - \sin \varphi_C \cos \Theta) \right] =$ $= \sin \gamma \cdot \sin \alpha \left[\cos \varphi_A \cdot \sin \Psi + \sin \varphi_A \cdot \cos \Psi \right]$

$\sin \alpha \left[ \sin^2 \gamma \cdot \cos (\Theta - \varphi_C) + \sin \gamma \cdot \cos \gamma \cdot \sin (\Theta - \varphi_C)\right] = \sin \gamma \cdot \sin \alpha \cdot \sin (\Psi + \varphi_A)$

$\sin \alpha \cdot \sin \gamma \cdot \sin (\gamma + \Theta - \varphi_C) = \sin \gamma \cdot \sin \alpha \cdot \sin (\beta + \varphi_A - \varphi_B + \varphi_C).$ The last statement is obvious.

vladimir.shelomovskii@gmail.com, vvsss

2024, Problem 19

A triangle $ABC,$ its circumcircle $\Omega$ , and its incenter $I$ are drawn on the plane.

Construct the circumcenter $O$ of $\triangle ABC$ using only a ruler.

Solution

We successively construct:

- the midpoint $D = BI \cap \Omega$ of the arc $AB,$

- the midpoint $E = CI \cap \Omega$ of the arc $AC,$

- the polar $H'H''$ of point $H \in DE,$

- the polar $G'G''$ of point $G \in DE,$

- the polar $F = H'H'' \cap G'G''$ of the line $DE,$

- the tangent $FD || AC$ to $\Omega,$

- the tangent $FE || AB$ to $\Omega,$

- the trapezium $ACDF,$

- the point $K = AF \cap CD,$

- the point $L = AD \cap CF,$

- the midpoint $M = AC \cap KL$ of the segment $AB,$

- the midpoint $M'$ of the segment $AC,$

- the diameter $DM$ of $\Omega,$

- the diameter $EM'$ of $\Omega,$

- the circumcenter $O = DM \cap EM'.$

vladimir.shelomovskii@gmail.com, vvsss

2024, Problem 18

Let $AH, BH', CH''$ be the altitudes of an acute-angled triangle $ABC, I_A$ be its excenter corresponding to $A, I'_A$ be the reflection of $I_A$ about the line $AH.$ Points $I'_B, I'_C$ are defined similarly. Prove that the lines $HI'_A, H'I'_B, H''I'_C$ concur.

Proof

Denote $I$ the incenter of $\triangle ABC.$ Points $A, I, I_A$ are collinear. We will prove that $I \in HI'_A.$ Denote $D \in BC, ID \perp BC, D' \in I'_AI_A, ID' \perp BC, E = BC \cap AI_A,$ $F \in BC, I_AF \perp BC, AH = h_A, ID = r, I_AF = r_A, BC = a, s$ - semiperimeter. $\frac {HD}{HF} = \frac {AI}{AI_A} = \frac {h_A - r}{h_A + r}.$ The area $[ABC] = r \cdot s = r_A (s - a) = \frac {a h_A}{2} \implies$ $\frac {1}{r} = \frac {1}{r_A} + \frac {2}{h_A} \implies \frac {h_A - r}{h_A+ r} = \frac {r_A}{r}.$ $\frac {I'_AD'}{HD} = \frac {HD + HF}{HD} = 1 + \frac {HF}{HD} = 1 + \frac {r_A}{r}= \frac {r_A + r}{r} \implies$ Points $I, H, I'_a$ are collinear, so the lines $HI'_A, H'I'_B, H''I'_C$ concur at the point $I.$

vladimir.shelomovskii@gmail.com, vvsss

2024, Problem 17

Let $\triangle ABC$ be not isosceles triangle, $\omega$ be its incircle.

Let $D, E,$ and $F$ be the points at which the incircle of $\triangle ABC$ touches the sides $BC, CA,$ and $AB,$ respectively.

Let $K$ be the point on ray $EF$ such that $EK = AB.$

Let $L$ be the point on ray $FE$ such that $FL = AC.$

The circumcircles of $\triangle BFK$ and $\triangle CEL$ intersect $\omega$ again at $Q$ and $P,$ respectively.

Prove that $BQ, CP,$ and $AD$ are concurrent.

Proof

$\frac {KE} {FL} = \frac {AB}{AC},$ so points $Q,P,$ and $G = FE \cap BC$ are collinear (see Symmetry and incircle for details).

Therefore lines $BQ, CP,$ and $AD$ are concurrent (see Symmetry and incircle A for details.)

vladimir.shelomovskii@gmail.com, vvsss

2024, Problem 16

Let $AA', BB',$ and $CC'$ be the bisectors of a triangle $\triangle ABC.$

The segments $BB'$ and $A'C'$ meet at point $D.$ Let $E$ be the projection of $D$ to $AC.$

Points $P$ and $Q$ on the sides $AB$ and $BC,$ respectively, are such that $EP = PD, EQ = QD.$

Prove that $\angle PDB' = \angle EDQ.$

Proof

$\triangle PDQ = \triangle PEQ (DQ = EQ, DP = PF, PQ$ is the common side) $\implies$

$PQ \perp DE, F = PQ \cap DE$ is the midpoint $DE \implies$

$G = BB' \cap PQ$ is the midpoint of $DB'.$ $\frac{BG}{BB'} =\frac {BQ}{BC} = \frac {BP}{BA} = \frac {BD}{BI}.$ (see Division of bisector for details.)

So $DQ || CC', PD || AA'.$ Denote $\angle ACC' = \angle BCC' = \gamma, \angle A'AC = \alpha, B'BC = \beta.$ $\angle PDB' = \angle AIB' = \angle BB'C - \angle IAC = 180^\circ - \beta - 2 \gamma - \alpha = 90^\circ - \gamma.$ $\angle QDE = 90^\circ - \angle DQP = 90^\circ - \gamma = \angle PDB'.$

Another solution see 2024_Sharygin_olimpiad_Problem_16

vladimir.shelomovskii@gmail.com, vvsss

2024, Problem 15

The difference of two angles of a triangle is greater than $90^\circ.$ Prove that the ratio of its circumradius and inradius is greater than $4.$

Proof

Suppose, $\angle BAC = \alpha = \angle ACB + 90^\circ = \gamma + 90^\circ.$

Let $\Omega = \odot ABC, C'$ be the point on $\Omega$ opposite $C, B'$ be the midpoint of arc $\overset{\Large\frown} {AC}.$ Then $\angle CAC' = 90^\circ \implies \angle BAC' = \angle ACB = \gamma = \angle BCC' \implies$ $\overset{\Large\frown} {AB} = \overset{\Large\frown} {BC'} \implies BB' || CC'.$ $BO = CO \implies \angle CBO = \angle OCB = \angle ACB = \gamma.$ $\angle ABC = \beta = 180^\circ - \gamma - \alpha = 90^\circ - 2 \gamma.$ $\angle OBB' = \frac {\beta}{2} + \gamma = 45^\circ.$ $BO = OB' = R \implies \angle BB'O = 45^\circ, \angle BOB' = 90^\circ.$ Incenter $I$ triangle $\triangle ABC$ lies on $BB',$ therefore $OI \ge \frac {R}{\sqrt {2}}.$

We use the Euler law $OI^2 = R^2 - 2 Rr \implies \frac {2r}{R} = 1 - \frac {OI^2}{R^2} \le \frac{1}{2} \implies \frac{R}{r} \ge 4.$

If $R = 4r$ then $OI \perp BB' \implies \frac {BI}{IB'} = 1 \implies \frac {BC + AB}{AC} = 2, BI = \frac{R}{\sqrt{2}}.$ $\sin CBI = \frac {r}{BI} = \frac{r \sqrt{2}}{R} = \frac{1}{2\sqrt{2}} \implies \sin \beta = \frac{\sqrt{7}}{4} \implies$ $AC = 2R \sin \beta = R \frac {\sqrt{7}}{2},AB = AC (1 - \frac{1}{\sqrt {7}}), BC = AC (1 + \frac{1}{\sqrt {7}}).$

If $\angle BAC' > \angle ACB \implies \frac {OI}{R}$ increases so $\frac {r}{R}$ decreases.

vladimir.shelomovskii@gmail.com, vvsss

2024, Problem 14

The incircle $\omega$ of a right-angled triangle $\triangle ABC$ touches the circumcircle $\theta$ of its medial triangle at point $F.$ Let $OE$ be the tangent to $\omega$ from the midpoint $O$ of the hypothenuse $AB$ distinct from $AB.$ Prove that $CE = CF.$

Proof

Let $\Omega$ and $I$ be the circumcircle and the incenter of $\triangle ABC, D = \omega \cap AB.$

Let $Q$ be nine-point center of $\triangle ABC, G$ be the point at $OB$ such that $OG = DO, K \in DI, KQ ||AB.$

Denote $r = ID, R = AO,a = BC, b = AC,c= AB, \beta = \angle ABC.$

$\triangle ABC$ is the right-angled triangle, so $Q$ is the midpoint $CO,$

$2R = c, r = \frac {a+b-c}{2},FQ = \frac{R}{2}.$ Let $h(X)$ be the result of the homothety of the point $X$ centered in $C$ with the coefficient $2.$ Then $h(\theta) = \Omega, F' = h(F) \in \Omega, O = h(Q) \implies FQ || F'O.$ $DO = EO = GO \implies \angle DEG = 90^\circ.$ $\angle DOE = 2 \angle DOI = 2 \angle EGD \implies \angle DOI = \angle EGD, IO || EG.$ WLOG, $a > b \implies DO = \frac {a-b}{2} = \tan IOD = \frac{r}{DO} = \frac{a+b-c}{a-b} = \frac{\cos\beta + \sin \beta -1}{\cos\beta - \sin \beta}.$

Let $H$ be the foot from $C$ to $\overline{AB}$ . $CH = a \sin \beta = c \sin \beta \cos \beta, BH = a \cos \beta, BG = AD = \frac{c+b-a}{2}.$ $\tan \angle CGH = \frac {CH}{BH - BG} = \frac{2 \sin \beta \cos \beta}{2 \cos^2 \beta +\cos \beta - \sin \beta -1} =$ $= \frac{(\sin \beta + \cos \beta -1)(\sin \beta + \cos \beta +1)}{(\cos \beta - \sin \beta)(\sin \beta + \cos \beta +1)} = \frac{\cos\beta + \sin \beta -1}{\cos\beta - \sin \beta} = \tan IOD.$ Therefore points $C,E,$ and $G$ are collinear. $\psi = \angle BCG = \angle AGC - \angle ABC \implies$ $\tan \psi = \frac {\tan \angle AGC - \tan \angle ABC}{1 + \tan \angle AGC \cdot \tan \angle ABC} = \frac {c - a}{c-b}.$ $\sin 2\psi = \frac {2\tan \psi}{1 + \tan^2 \psi} = \frac {2(c-a)(c-b)}{c(3c - 2b - 2a)},$ $\angle AOF' = \angle KQI, \sin \angle KQI = \frac{CH/2 -r}{QF - r}.$ $4c(QF - r) = c(c -2a -2b +2c) = c(3c -2a -2b),$ $2c(CH - 2r) = 2(ab -ac -bc +c^2) = 2(c - a)(c - b),$ $\sin \angle KQI = \frac {2(c-a)(c-b)}{c(3c - 2b - 2a)}= \sin 2 \psi \implies \angle KQI = 2 \psi.$ $\angle ACF' = \frac {\angle AOF'}{2} = \frac {\angle KQI}{2} = \psi = \angle BCG \implies \angle FCI = \angle ECI \implies CF = CE.$ vladimir.shelomovskii@gmail.com, vvsss

2024, Problem 12

The bisectors $AE, CD$ of a $\triangle ABC$ with $\angle B = 60^\circ$ meet at point $I.$

The circumcircles of triangles $ABC, DIE$ meet at point $P.$

Prove that the line $PI$ bisects the side $AC.$

Proof

Denote $M$ the midpoint $AC, \omega = \odot DIE,$ $\varphi = \angle CIM, \phi = \angle DIP, f(x) = \frac {\sin x}{\sin(120^\circ -x)}.$ $\angle AIC = 90^\circ + \frac {\angle ABC}{2} = 120^\circ = 180^\circ - \angle ABC \implies B \in \omega.$ In triangles $\triangle CIM$ and $\triangle AIM$ , by applying the law of sines, we get $\frac {IM}{\sin \angle ACI} = \frac {CM}{\sin \varphi}, \frac {IM}{\sin \angle CAI} = \frac {AM}{\sin (120^\circ -\varphi)} \implies f(\varphi) = \frac {\sin \varphi} {\sin (120^\circ -\varphi)} = \frac {\sin \angle ACI}{\sin \angle CAI}.$

We use the formulas for circle $\omega$ and get $\frac {PD}{\sin \angle PID} = \frac {PE}{\sin \angle PIE} \implies f(\phi) = \frac {\sin \phi} {\sin (120^\circ -\phi)} = \frac {PD}{PE}.$

$\angle BDI = \angle AEC \implies \angle ADC = 180^\circ - \angle AEC.$ In triangles $\triangle ADC$ and $\triangle AEC$ , by applying the law of sines, we get $\frac {AD}{\sin \angle ACD} = \frac {AC}{\sin \angle ADC} = \frac {AC}{\sin \angle AEC} \implies \frac {AD}{CE} = \frac {\sin \angle ACI}{\sin \angle CAI}.$

$\angle BEP = \angle BDP, \angle BCP = \angle BAP \implies \triangle APD \sim \triangle CPE \implies \frac {AD}{CE} = \frac {PD}{PE}.$

Therefore $f(\phi) = f(\varphi).$ The function $f$ increases monotonically on the interval $(0, \frac {2 \pi}{3}).$

This means $\phi = \varphi$ and points $P,I,$ and $M$ are collinear.

vladimir.shelomovskii@gmail.com, vvsss

2024, Problem 9

Let $ABCD (AD || BC$ be a trapezoid circumscribed around a circle $\omega,$ centered at $O$ which touches the sides $AB, BC, CD,$ and $AD$ at points $P, Q, R, S,$ respectively.

The line passing trough $P$ and parallel to the bases of trapezoid meets $QR$ at point $X.$

Prove that $AB, QS,$ and $DX$ concur.

Solution

Solution 1. $AP = AS \implies \angle AOS = \frac {\overset{\Large\frown} {PS}}{2} = \angle PQS \implies PQ||AO.$

$OD \perp OC, QR \perp OC \implies OD || QX. AD ||PX \implies$

$E = AP \cap QS$ is the center of similarity of triangles $\triangle PQX$ and $\triangle AOD.$

Solution 2. $\triangle ODS \sim \triangle QXG \implies \frac {SD}{GX} = \frac {SO}{GQ} = \frac {1}{2} \cdot \frac {SQ}{GQ} = \frac {1}{2} \cdot \frac {AB}{PB}.$

Denote $EA = x, AP = AS = y, BP = BQ = z.$

$\triangle AES \sim BEQ \implies \frac {x}{x+y+z}= \frac {y}{z} \implies xz = xy + y^2 + yz \implies 2xz = xy + y^2 + yz + xz \implies$

$\frac {x}{x+y}= \frac {y+z}{2z} \implies \frac {AS}{AG} = \frac {1}{2} \cdot \frac {AB}{PB} \implies \triangle ESD \sim \triangle EGX \implies E \in DX.$ vladimir.shelomovskii@gmail.com, vvsss

2024, Problem 8

Let $ABCD$ be a quadrilateral with $\angle B = \angle D$ and $AD = CD.$

The incircle of $\triangle ABC$ touches the sides $BC$ and $AB$ at points $E$ and $F$ respectively.

The midpoints of segments $AC, BD, AE,$ and $CF$ are points $M,X,Y,Z.$

Prove that points $M,X,Y,Z.$ are concyclic.

Solution

$ZM || AB, YM || BC \implies \angle YMZ = \angle ABC = \angle ADC = \alpha.$ $2 \vec {XY} = \vec {DA} + \vec {BE}, 2 \vec {XZ} = \vec {DC} + \vec {BF}.$ $\vec {DC}$ is the rotation of $\vec {DA}$ around a point $D$ through an angle $\alpha.$

$\vec {BF}$ is the rotation of $\vec {BE}$ around a point $B$ through an angle $\alpha.$

So $\vec {XZ}$ is the rotation of $\vec {XE}$ around a point $X$ through an angle $\alpha.$

vladimir.shelomovskii@gmail.com, vvsss

2024, Problem 2

Three distinct collinear points are given. Construct the isosceles triangles such that these points are their circumcenter, incenter and excenter (in some order).

Solution

Let $M$ be the midpoint of the segment connecting the incenter and excenter. It is known that point $M$ belong the circumcircle. Construction is possible if a circle with diameter IE (incenter – excenter) intersects a circle with radius OM (circumcenter – M). Situation when $E$ between $I$ and $O$ is impossible.

Denote points $A, B, C$ such that $B \in AC$ and $AB \le BC.$

Suppose point $A$ is circumcenter, so $B$ is incenter. $M$ is midpoint BC. The vertices of the desired triangle are located at the intersection of a circle with center $A$ and radius $AM$ with $\omega$ and a line $AB.$

Suppose point $C$ is circumcenter, so $B$ is incenter. $M$ is midpoint $AB.$ The vertices of the desired triangle are located at the intersection of a circle with center $A$ and radius $AM$ with $\omega$ and a line $AB.$

Suppose point $B$ is circumcenter, so $A$ is incenter. $M$ is midpoint $AB.$ Suppose $3 AB < BC.$ The vertices of the desired triangle are located at the intersection of a circle with center $B$ and radius $BM$ with $\omega$ and a line $AB.$

If $3 AB \ge BC$ there is not desired triangle.

vladimir.shelomovskii@gmail.com, vvsss

Page

Toolbox

Search

Difference between revisions of "Sharygin Olympiads, the best"

Latest revision as of 16:04, 4 November 2024

Contents

2024 tur 2 klass 10 Problem 6

2024 tur 2 klass 10 Problem 7

2024 tur 2 klass 9 Problem 7

2024 tur 2 klass 9 Problem 5

2024 tur 2 klass 9 Problem 4

2024 tur 2 klass 9 Problem 3

2024 tur 2 klass 8 Problem 4

2024 tur 2 klass 8 Problem 2

2024, Problem 23

One-to-one mapping of the circle

2024, Problem 22

2024, Problem 21

2024, Problem 20

2024, Problem 19

2024, Problem 18

2024, Problem 17

2024, Problem 16

2024, Problem 15

2024, Problem 14

2024, Problem 12

2024, Problem 9

2024, Problem 8

2024, Problem 2

@@ Line 2: / Line 2: @@
 The best problems of these Olympiads will be published. The numbering contains the year of the Olympiad and the serial number of the problem. Solutions are often different from the original ones.
+==2024 tur 2 klass 10 Problem 6==
+[[File:2024 final 10 6.png|350px|right]]
+A point <math>P</math> lies on one of medians of triangle <math>\triangle ABC</math> in such a way that <math>\angle PAB = \angle PBC = \angle PCA.</math> Prove that there exists a point <math>Q</math> on another median such that <math>\angle QBA = \angle QCB = \angle QAC.</math>  (A.Zaslavsky)
+<i><b>Proof</b></i>
+. Denote <math>a = BC, b = AC, c = AB. </math>
+It is known that barycentric coordinates are
+<cmath>P = (a^2 b^2 : b^2 c^2 : c^2 a^2), P \in AD \implies b^2 c^2 = c^2 a^2 \implies AC = BC.</cmath>
+. Denote <math>\omega = \odot APB, \Omega = \odot APC. </math>
+<math>\angle ABP = \angle PAC \implies AC </math> is tangent to <math>\omega.</math>
+<math>\angle PAC = \angle PCB \implies BC</math> is tangent <math>\Omega.</math>
+<math>PC</math> is the radical axes of <math>\omega</math> and <math>\Omega,</math> the power of a point <math>D</math> with respect to a circle <math>\Omega</math> is <math>CD^2</math> so the power of a point <math>D</math> with respect to a circle <math>\omega</math> is <math>CD^2.</math>
+<math>BD^2 = CD^2,</math> so <math>BD</math> is tangent to <math>\omega \implies \angle ABC = \angle CAB \implies AC = BC.</math>
+<math>AC = BC,</math> so point <math>Q</math> symmetrical to <math>P</math> with respect to the <math>C-</math>median satisfies the conditions. <math>\blacksquare</math>
+'''vladimir.shelomovskii@gmail.com, vvsss'''
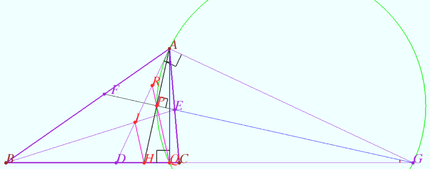
+==2024 tur 2 klass 10 Problem 7==
+[[File:2024 final 10 7.png|380px|right]]
+Let <math>ABC</math> be a triangle with <math>\angle A = 60^\circ, AD, BE,</math> and <math>CF</math> be its bisectors, <math>P, Q</math> be the projections of <math>A</math> to <math>EF</math> and <math>BC</math> respectively, and <math>R</math> be the second common point of the circle <math>\omega = \odot DEF</math> with <math>AD.</math>
+Prove that points <math>P, Q, R</math> are collinear. (K.Belsky)
+<i><b>Proof</b></i>
+Denote <math>a = BC, b = AC, c = AB, I -</math> the incenter of <math>\triangle ABC,</math>
+<math>L=FE \cap AD, R' -</math> the midpoint of <math>AI.</math>
+It is known ([[Bisector | Division of bisector]]) that
+<cmath>\frac {AI}{DI} = \frac {b+c}{a}, \frac {DI}{IL} = 1+ \frac {2a}{b+c} \implies \frac{1}{IL} = \frac{1}{DI} + \frac{2}{AI}   \implies</cmath>  <cmath>2 DI (\frac{AI}{2} - IL) =AI \cdot IL \implies 2 DI \cdot LR' = AI \cdot IL.</cmath>
+<cmath>DL \cdot LR'  = (DI + IL) \cdot LR' = \frac {AI \cdot IL}{2} + IL \cdot  (\frac{AI}{2} - IL) = AI \cdot IL - IL^2 = AL \cdot IL.</cmath>
+<math>\angle BAC = 60^\circ \implies \angle BIC = \angle FIE = 120^\circ \implies AEIF </math> is cyclic.
+Therefore <math>AL \cdot IL = LE \cdot LF = DL \cdot LR' \implies EDFR'</math> is cyclic <math>\implies R = R'.</math>
+[[File:2024 final 10 7a.png|430px|right]]
+Let <math>AG \perp AD, G \in BC, H = AP \cap BC, Q' = RP \cap BC.</math>
+It is known that points <math>F, E,</math> and <math>G</math> are collinear,
+<cmath>\angle AGB = \frac{|\angle ACB - \angle ABC|}{2}, \angle AGE = \angle BGE.</cmath>
+<math>AP \perp EF, AQ \perp BG \implies AG</math> is the diameter of <math>\odot APQG \implies</math>
+<cmath>\overset{\Large\frown} {QP} = \overset{\Large\frown} {AP} \implies \angle DAP = \angle QAP, AP = PH.</cmath>
+<cmath>AR = RI, AP = PH \implies RP || IH \implies \frac {DH}{HQ'} = \frac {DI}{IR} = \frac {2a}{b+c}.</cmath>
+<math>AH</math> is the bisector of <math>\angle DAQ \implies  \frac {DH}{HQ} = \frac {AD}{AQ}.</math>
+Bisector <math>AD = \frac {2 b c \cos 30^\circ}{b+c}.</math>
+Altitude <math>AQ =  \frac {2[ABC]}{BC}= \frac {bc \sin 60^\circ}{a} \implies \frac {AD}{AQ} = \frac {2a}{b+c} = \frac {DH}{HQ} = \frac {DH}{HQ'} \implies Q = Q'. \blacksquare</math>
+Note that the point <math>R</math> is a Feuerbach point of <math>\triangle ABC</math> since both the inscribed circle and the Euler circle pass through it.
+'''vladimir.shelomovskii@gmail.com, vvsss'''
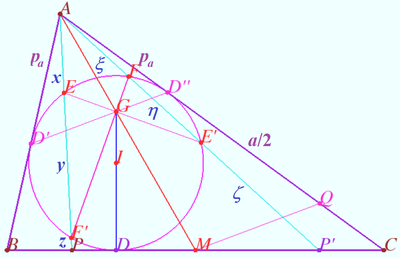
+==2024 tur 2 klass 9 Problem 7==
+[[File:Incircle and secants.png|400px|right]]
+Let  triangle <math>\triangle ABC</math> and point <math>P</math> on the side <math>BC</math> be given. Let <math>P'</math> be such point on the side <math>BC</math> that <math>BP = P'C.</math> The cross points of segments <math>AP</math> and <math>AP'</math> with the incircle <math>\omega</math> of <math>\triangle ABC</math> form a convex quadrilateral <math>EFE'F'.</math>
+Find the locus of crosspoints of diagonals <math>EFE'F'.</math> (D.Brodsky)
+<i><b>Solution</b></i>
+. Particular case of [[Projective geometry (simplest cases) | Fixed point]] .
+. Denote <math>p_a = \frac{b+c-a}{2}, p_b = \frac{a-b+c}{2}, p_c = \frac{a+b-c}{2}, m = \frac {CP}{BP}, \mu = \frac {PF'}{AF'}.</math>
+<cmath>PD = \frac {m p_b - p_c} {m+1}, AD' = p_a \implies</cmath>
+<cmath>x(x+y) = p_a^2, z(z+y) = PD^2, x+y+z = AP, \mu = \frac {z}{x+y}.</cmath>
+We perform simple transformations and get:
+<cmath>\mu^2 p_a^2 - \mu (AP^2 - p_a^2 - PD^2) + PD^2 = 0.</cmath>
+We use Stewart's theorem and get:
+<cmath> AP^2 = \frac {AC^2}{1+m} + \frac {m AB^2}{1+m} - \frac {m BC^2}{(1+m)^2} \implies</cmath>
+<cmath>(\mu p_a)^2 - 2 (\mu p_a) \frac {m p_b + p_c}{m+1} + \frac {(m p_b - p_c)^2} {(m+1)^2} = 0.</cmath>
+<cmath>\mu  = \frac {(\sqrt{m p_b} \pm \sqrt{p_c})^2}{(m+1) p_a}.</cmath>
+Similarly <cmath>\nu  =  \frac {\eta + \zeta}{\xi}= \frac {(\sqrt{m p_c} \pm \sqrt{p_b})^2}{(m+1) p_a}.</cmath>
+Therefore <math>\frac {\nu + \mu}{2} = \frac {a}{2 p_a}</math> not depends from <math>m.</math>
+Let <math>M</math> be the midpoint of <math>BC, AM</math> is the median of <math>\triangle ABC</math> and <math>\triangle PAP'.</math>
+The line <math>FF'</math> cross the median of <math>\triangle PAP'</math> at point <math>G'</math> such that <math>\frac {MG'}{G'A} = \frac {\nu + \mu}{2} = \frac {p_b + p_c}{2 p_a} = \frac {a}{2 p_a}.</math>
+So point <math>G'</math> is fixed and this point lyes on <math>EE' \implies G = G'</math>.
+Therefore the locus of crosspoints of diagonals <math>EFE'F'</math> is point <math>G.</math>
+<i><b>Corollary</b></i>
+Let line <math>MQ||D'D'', Q \in AC</math>. Then <math>\frac {D''Q}{AD''} = \frac {a}{2 p_a} \implies 2 D''Q = BC.</math>
+'''vladimir.shelomovskii@gmail.com, vvsss'''
+==2024 tur 2 klass 9 Problem 5==
+[[File:2024 final 9 5.png|350px|right]]
+Let <math>ABC</math> be an isosceles triangle <math>(AC = BC), O</math> be its circumcenter,<math>H</math> be the orthocenter, and <math>P</math> be a point inside the triangle such that <math>\angle APH = \angle BPO = 90^\circ.</math>
+Prove that  <math>\angle PAC =  \angle PBA =  \angle PCB.</math> (A.Zaslavsky)
+<i><b>Proof</b></i>
+Denote <math>E -</math> the midpoint <math>BC, M-</math> the midpoint <math>AB, D -</math> the foot from <math>B</math> to <math>\overline{AC}, \alpha = \angle ABC, \omega = \odot ADHM, \Omega = \odot BEOM, \Theta = \odot BHC, \theta = \odot AB</math> tangent to <math>\overline{BC}.</math>
+<math>AH = BH, BO = CO , \angle BAH =  90^\circ - \alpha = \angle BCO \implies \triangle ABH \sim \triangle BCO.</math>
+There is a spiral similarity <math>T</math> centered at point <math>X</math> that maps <math>\triangle ABH</math> into <math>\triangle BCO.</math>
+The coefficient of similarity  <math>k = \frac {BC}{AB} = \frac {1}{2 \cos \alpha},</math> rotation angle equal  <math>180^\circ - \alpha.</math>
+<cmath>B = T(A), B = AB \cap BC \implies X \in \theta.</cmath>
+<cmath>C = T(B), B = AB \cap BC \implies X \in \Theta</cmath>
+<math>(\angle BCH = \angle ABH,</math> so <math>AB</math> is tangent to <math>\Theta).</math> [[Spiral similarity | Basic information]]
+<cmath>E = T(M), B = AM \cap BE \implies X \in \Omega.</cmath>
+<cmath>AB = T(CA), CO = T(BH), D = AC \cap BH, M = AB \cap CO \implies M = T(D).</cmath>
+<cmath>A = AD \cap BM \implies X \in \omega.</cmath>
+<cmath>P = \theta \cap \Theta \implies P = X.</cmath>
+<cmath>P = T(P) \implies \triangle BPC = T(\triangle APB) \implies \triangle BPC \sim \triangle APB \implies \angle PAB = \angle PCB.</cmath>
+<cmath>\triangle OBP = T(\triangle HAP) \implies \triangle OBP \sim \triangle HAP \implies \angle OBP = \angle HAP .</cmath>
+<cmath>\angle PAC = \alpha - \angle HAP - \angle BAH = \alpha - \angle OBP - \angle OBC = \angle PBA.\blacksquare</cmath>
+<cmath>\triangle ADM \sim \triangle ABC \implies \angle ADM = \alpha = \angle APM, \angle EPM =  180^\circ - \alpha \implies</cmath>
+Points <math>A,P,</math> and <math>E</math> are collinear, so <math>P \in A-</math> median of <math>\triangle ABC.</math>
+<math>\angle PAB = \angle PCB, \angle ABC = \angle BAC \implies PC</math> is <math>B-</math> symmedian of <math>\triangle ABC.</math>
+<math>\angle APH =  90^\circ \implies P</math> is <math>A-</math> Humpty point.
+'''vladimir.shelomovskii@gmail.com, vvsss'''
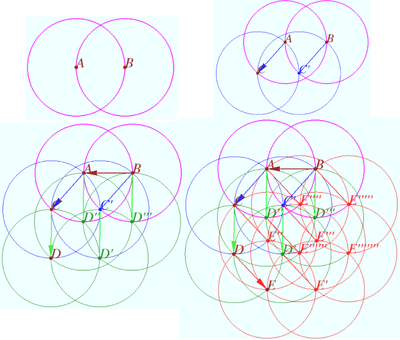
+==2024 tur 2 klass 9 Problem 4==
+[[File:2024 final 9 4d.png|400px|right]]
+For which <math>n > 0</math> it is possible to mark several different points and several different circles on the plane in such a way that:
+- exactly <math>n</math> marked circles pass through each marked point;
+- exactly <math>n</math> marked points lie on each marked circle;
+- the center of each marked circle is marked? (P.Puchkov)
+<i><b>Solution</b></i>
+Case <math>n = 1.</math> Circles centered at <math>A</math> and <math>B</math> with radii <math>R = |\vec {AB}|.</math>
+Case <math>n = 2, \vec {AC} = \vec {BC'}, |\vec {AB} = \vec {AC}|, \vec {AC}</math> is not paralel to <math>\vec {AB}.</math>
+Four circles are centered at points <math>A, B, C,</math> and <math>C'.</math> Each radius is equal <math>R.</math>
+Case <math>n = 3, \vec {CD} = \vec {AD''} = \vec {C'D'} = \vec {BD'''}, \vec {CD}</math> is not paralel to <math>\vec {AB}</math> or <math>\vec {AC}, |\vec {AB}| = \vec {CD}|</math>
+Eight circles centered at <math>A, B, C, C', D, D',D''</math> and <math>D'''</math> have radii <math>R.</math>
+Case <math>n = 4, \vec {DE} = \vec {D'E'} = ...,|\vec {DE}| = R,..</math>
+<i><b>Answer </b></i> For all <math>n.</math>
+'''vladimir.shelomovskii@gmail.com, vvsss'''
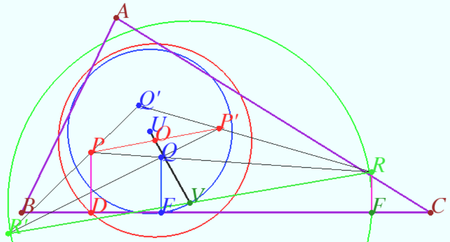
+==2024 tur 2 klass 9 Problem 3==
+[[File:2024 final 9 3.png|450px|right]]
+Let <math>(P,P')</math> and <math>(Q,Q')</math> be two pairs of points isogonally conjugated with respect to a triangle <math>ABC,</math> and <math>R</math> be the common point of lines <math>PQ</math> and <math>P'Q'.</math>
+Prove that the pedal circles of points <math>P,Q,</math> and <math>R</math> are coaxial. (L.Shatunov, V.Shelomovskii)
+<i><b>Solution</b></i>
+. Let <math>P'</math> be the isogonal conjugate of a point <math>P</math> with respect to a triangle <math>\triangle ABC.</math> Then circle centered at the midpoint <math>PP'</math> is the common pedal circle of points <math>P</math> and <math>P'.</math> ([[Isogonal conjugate | Circumcircle of pedal triangles]]) So center <math>O</math> is the midpoint <math>PP'</math> and center <math>U</math> is the midpoint <math>QQ'.</math>
+. Denote <math>R' = PQ' \cap P'Q.</math> Then <math>R'</math> is the isogonal conjugate of a point <math>R</math> with respect to <math>\triangle ABC.</math> So center <math>V</math> is the midpoint <math>RR'.</math> ([[Isogonal conjugate | Two pares of isogonally conjugate points]])
+. The Gauss line (or Gauss–Newton line) is the line joining the midpoints of the three diagonals of a complete quadrilateral  <math>PQ'P'Q</math> ([[Gauss line]]).So points <math>U,O,</math> and <math>V</math> are collinear as was to be proven.  <math>\blacksquare</math>
+'''vladimir.shelomovskii@gmail.com, vvsss'''
+== 2024 tur 2 klass 8 Problem 4==
+[[File:2024 final 8 4.png|300px|right]]
+[[File:2024 final 8 4a.png|300px|right]]
+A square with sidelength <math>1</math> is cut from the paper. Construct a segment with length <math>\frac{1}{2024}</math> using at most <math>13</math> folds. No instruments are available, it is allowed only to fold the paper and to mark the common points of folding lines. (M.Evdokimov)
+<i><b>Solution</b></i>
+Main idea: <math>\frac{1}{2024} = \frac{1}{4} \cdot \left [ \frac{1}{22} - \frac{1}{23} \right ].</math>
+<cmath>\frac {a}{b} = \frac{x}{x+c} \implies x = \frac {a \cdot c}{b - a}.</cmath>
+<cmath>EG = EA - GA = c \cdot \left [ \frac {1}{b/a - 1} - \frac {1}{(b+FB)/a - 1} \right ].</cmath>
+Let <math>c = \frac{1}{2}, a = \frac{1}{64}, b = \frac{45}{64}, FB = \frac{2}{64}.</math>
+<cmath>EG = \frac{1}{2} \cdot \left [ \frac {1}{45 - 1} - \frac {1}{47 - 1} \right] = \frac{1}{2024}.</cmath>
+We perform <math>1</math> horizontal fold of the sheet. We get line <math>AD (AC = \frac{1}{2}).</math> We perform
+<math>6</math> vertical folds of the sheet. We get <math>62</math> vertical lines at a distance of <math>\frac{1}{64}</math> from each other.
+Point <math>F</math> is the lower left corner of the sheet, point <math>B</math> is the lower point of the second vertical line,  point <math>C</math> is the lower point of the <math>47^{th}</math>  line, point <math>D</math> is the point at the intersection of the horizontal line and the <math>46^{th}</math>  vertical line.
+Points <math>E</math> and <math>G</math> are at the intersection of the lines <math>BD</math> and <math>FD</math> and the <math>47^{th}</math> vertical line.
+'''vladimir.shelomovskii@gmail.com, vvsss'''
+== 2024 tur 2 klass 8 Problem 2==
+[[File:2024 final 8 2.png|450px|right]]
+Let <math>M</math> be the midpoint of side <math>AB</math> of an acute-angled triangle <math>ABC,</math> and <math>P</math> be the projection of the orthocenter <math>H</math> to the bisector of angle <math>C.</math>
+Prove that <math>MP</math> bisects the segment <math>CH.</math> (L.Emelyanov)
+<i><b>Solution</b></i>
+Denote <math>D</math> - the midpoint of <math>CH, A',B',</math> and <math>C'</math> the foots of the heights, <math>\angle A = 2 \alpha, \angle B = 2 \beta, \angle C = 2 \gamma, \omega</math> be the Euler circle <math>A'DB'C'M.</math>
+<math>\Omega</math> is the circle <math>\odot CA'B'</math> with the diameter <math>CH.</math>
+<cmath>HP \perp CP \implies P \in \Omega.</cmath>
+<cmath>AA' \perp BA'  \implies A'M = BM \implies \angle BA'M = 2 \beta.</cmath>
+<cmath>\triangle ABC \sim \triangle A'BC' \implies \angle BA'C' = 2 \alpha \implies</cmath>
+<cmath>\angle MA'C' = 2|\alpha - \beta| = \angle MDC'.</cmath>
+<cmath>\angle ACC' = 90^\circ - 2 \alpha, \angle ACP  = \gamma,</cmath> <cmath>\angle PCC' = |\angle ACC' - \angle ACP| =
+|90^\circ - 2 \alpha -  \gamma| = | \alpha + \beta +  \gamma - 2 \alpha -  \gamma| = | \alpha - \beta|.</cmath>
+<cmath>PD = CD \implies \angle PDH =  2 \angle PCD = 2|\alpha - \beta|.</cmath>
+<math>\angle PDH = \angle MDH \implies</math> points <math>M,P,</math> and <math>D</math> are collinear.
+'''vladimir.shelomovskii@gmail.com, vvsss'''
 ==2024, Problem 23==
@@ Line 18: / Line 233: @@
 <math>O</math> is the circumcenter of <math>\triangle APC, O'</math> is the circumcenter of <math>\triangle BPC.</math>
-Let <math>K</math> and <math>L</math> be the midpoints of the arcs <math>\overset{\Large\frown}{CB'}</math> of <math>\theta.</math>
+Let <math>K</math> and <math>L</math> be the midpoints of the arcs <math>\overset{\Large\frown}{CB'}</math> of <math>\theta,D = AL \cap \omega.</math>
-Let <math>K'</math> and <math>L'</math> be the midpoints of the arcs <math>\overset{\Large\frown}{CA'}</math> of <math>\theta'.</math>
+Let <math>K'</math> and <math>L'</math> be the midpoints of the arcs <math>\overset{\Large\frown}{CA'}</math> of <math>\theta', D' = BL' \cap \omega'.</math>
 These points not depends from position of point <math>P.</math>
@@ Line 28: / Line 243: @@
 <cmath>O'D' = O'C, OC = OD \implies \triangle OCD \sim \triangle O'D'C \implies OC||O'D'.</cmath>
 Let <math>F= CD \cup OO' \implies \frac {FO}{FO'} = \frac {OC}{O'D'} \implies Q = F.</math>
+[[File:2024 23 3.png|350px|right]]
 <cmath>\angle LCB' = \alpha = \angle B'BL' \implies LC || L'B.</cmath>
 Similarly, <math>AL || CL' \implies \triangle DLC \sim \triangle CL'D' \implies \frac {LC}{L'D'} = \frac {DC}{CD'} = \frac {OC}{O'D'}.</math>
@@ Line 34: / Line 250: @@
 Therefore <math>Q \in LL'.</math> Similarly, if <math>P \in \overset{\Large\frown} {B'A'}</math> then <math>Q \in KK'.</math>
+<i><b>Claim</b></i>
+Points <math>D, C,</math> and <math>D'</math> are collinear.
+<i><b>Proof</b></i>
+<math>S</math> is the midpoint of arc <math>\overset{\Large\frown}{A'B'} \implies \angle SAC = \angle SBC.</math>
+Denote <math>\angle CAP = \alpha, \angle CBP = \beta, \angle SAC = \angle SBC = \varphi.</math>
+<cmath>D \in \omega \implies \angle PDC = \alpha, \angle PCD = \pi - \alpha - \varphi.</cmath>
+<cmath>D' \in \omega' \implies \angle PD'C = \beta, \angle PCD' = \pi - \beta - \varphi.</cmath>
+<cmath>S \in \Omega \implies \angle SAP + \angle SBP =  \alpha + \beta + 2 \varphi = \pi.</cmath>
+Therefore <math>\angle PCD + \angle PCD' = \pi \implies </math> points <math>D, C,</math> and <math>D'</math> are collinear.
+'''vladimir.shelomovskii@gmail.com, vvsss'''
+==One-to-one mapping of the circle==
+[[File:2024 23 AA.png|350px|right]]
+Let a circle <math>\Omega,</math> two fixed points <math>A</math> and <math>B</math> on it and a point <math>C</math> inside it be given.
+Then there is a one-to-one mapping of the circle <math>\Omega</math> onto itself, based on the following two theorems.
+. Let a circle <math>\Omega,</math> two fixed points <math>A</math> and <math>B</math> on <math>\Omega,</math> and a point <math>C</math> inside <math>\Omega</math> be given.
+Let an arbitrary point <math>Q \in \Omega</math> be given.
+Let <math>A' = AC \cap \Omega, A' \ne A, B' = BC \cap \Omega, B' \ne B. S</math> is the midpoint of the arc <math>A'B', D = A'Q \cap BS, E = B'Q \cap AS.</math>
+Denote <math>\omega = \odot AEC, \omega' = \odot BCD, P = \omega \cap \omega'.</math> Prove that <math>P \in \Omega.</math>
+. Let a circle <math>\Omega,</math> two fixed points <math>A</math> and <math>B</math> on <math>\Omega,</math> and a point <math>C</math> inside <math>\Omega</math> be given.
+Let an arbitrary point <math>P \in \Omega</math> be given.
+Let <math>A' = AC \cap \Omega, A' \ne A, B' = BC \cap \Omega, B' \ne B. S</math> is the midpoint of the arc <math>A'B'.</math>
+Denote <math>\omega = \odot ACP, \omega'  = \odot BCP, D = BS \cap \omega' , E = \omega \cap AS.</math>
+Denote <math>Q = A'D \cap B'E.</math> Prove that <math>Q \in \Omega.</math>
+<i><b>Proof</b></i>
+<math>1. C = AA' \cap BB', D = A'Q \cap BS, E = B'Q \cap AS \implies </math>
+Points <math>D,C,E </math> are collinear.
+<cmath>\angle APC = \angle SEC, \angle BPC  = \angle SDC.</cmath>
+<cmath>\angle APC + \angle BPC + \angle ASC = \angle SEC + \angle SDC +  \angle DSE = 180 ^\circ \implies P \in \Omega.</cmath>
+. Points <math>D, C,</math> and <math>E</math> are collinear (see Claim in <i><b>2024, Problem 23</b></i>).
+We use Pascal's theorem for points <math>A,B',S,A',B</math> and crosspoints <math>C,D,E</math> and get <math>Q \in \Omega.</math>
 '''vladimir.shelomovskii@gmail.com, vvsss'''
 ==2024, Problem 22==
 [[File:2023 22 2.png|350px|right]]
 [[File:2024 22.png|350px|right]]
-A segment <math>AB</math> is given. Let <math>C</math> be an arbitrary point of the perpendicular bisector to <math>AB, O</math> be the point on the circumcircle of <math>\triangle ABC</math> opposite to <math>C,</math> and an ellipse centered at <math>O</math> touche <math>AB, BC, CA.</math>
+A segment <math>AB</math> is given. Let <math>C</math> be an arbitrary point of the perpendicular bisector to <math>AB,</math>
+<math>O</math> be the point on the circumcircle of <math>\triangle ABC</math> opposite to <math>C,</math> and an ellipse centered at <math>O</math> touche <math>AB, BC, CA.</math>
 Find the locus of touching points <math>P</math> of the ellipse with the line <math>BC.</math>
@@ Line 48: / Line 316: @@
 <cmath>\angle CBO = 90^\circ \implies \angle COB = \alpha,  MB = b \tan \alpha,</cmath>
-<cmath>CB = \frac {b \sin \alpha}{\cos^2 \alpha}, CO = \frac {b} {\cos^2 \alpha}, CN = b \left (1 +  \frac {1} {\cos^2 \alpha} \right ).</cmath>
+<cmath>CB = \frac {b \sin \alpha}{\cos^2 \alpha}, CO = \frac {b} {\cos^2 \alpha}, CD = b \left (1 +  \frac {1} {\cos^2 \alpha} \right ).</cmath>
 In order to find the ordinate of point <math>P,</math> we perform an affine transformation (compression along axis <math>AB)</math> which will transform the ellipse <math>MPD</math> into a circle with diameter <math>MD.</math> The tangent of the <math>CP</math> maps into the tangent of the <math>CE, E = \odot CBO \cap \odot MD, PF \perp CO.</math>
 <cmath>\angle OEF = \angle ECO \implies OF = OE \sin \angle OEF = OE  \sin \angle ECO = b \cos^2 \alpha.</cmath>
 <cmath>CP = \frac {CF}{\sin \alpha} = \frac {b}{\sin \alpha}\left ( \frac {1} {\cos^2 \alpha} - \cos^2 \alpha \right ) = b \sin \alpha  \left ( \frac {1}{\cos^2 \alpha } + 1 \right).</cmath>
 <cmath>\frac {CP}{CD} = \sin \alpha , \angle PCD = 90^\circ - \alpha \implies \angle CPD = 90^\circ.</cmath>
-<cmath>BP = CP - CB = \sin \alpha </cmath>
+<cmath>BP = CP - CB = b \sin \alpha.</cmath>
 Denote <math>Q = AB \cap DP \implies BQ = \frac {BP}{\cos \alpha} = b \tan \alpha = MB.</math>
@@ Line 61: / Line 329: @@
 '''vladimir.shelomovskii@gmail.com, vvsss'''
 ==2024, Problem 21==
 [[File:2024 21 0.png|350px|right]]
@@ Line 86: / Line 355: @@
 '''vladimir.shelomovskii@gmail.com, vvsss'''
+==2024, Problem 20==
+[[File:2024 20.png|350px|right]]
+Let a triangle <math>ABC,</math> points <math>D</math> and <math>E \in BD</math> be given, <math>F = AD \cap CE.</math>
+Points <math>D', E'</math> and <math>F'</math> are the isogonal conjugate of the points <math>D, E,</math> and <math>F,</math> respectively, with respect to <math>\triangle ABC.</math>
+Denote <math>R</math> and <math>R'</math> the circumradii of triangles <math>\triangle DEF</math> and <math>\triangle D'E'F',</math> respectively.
+Prove that <math>\frac {[DEF]}{R} = \frac {[D'E'F']}{R'},</math> where <math>[DEF]</math> is the area of <math>\triangle DEF.</math>
+<i><b>Proof</b></i>
+Denote <math>\angle BAC = \alpha, \angle ABC = \beta, \angle ACB = \gamma,</math>
+<cmath>\angle EDF = \Theta, \angle E'D'F' = \theta, \angle DEF = \Psi, \angle D'E'F' = \psi,</cmath>
+<cmath>\angle BAD' = \angle CAF = \varphi_A, \angle CBD' = \angle ABD = \varphi_B, \angle BCE' = \angle ACE = \varphi_C.</cmath>
+It is easy to prove that
+<math>\frac {[DEF]}{R} = \frac {[D'E'F']}{R'}</math> is equivalent to <math>DE \cdot \sin \Theta \cdot \sin \Psi = D'E' \cdot \sin \theta \cdot \sin \psi.</math>
+<cmath>\Theta = \alpha - \varphi_A +  \varphi_B, \theta = \beta -  \varphi_B +  \varphi_A, \Psi = \beta -  \varphi_B +  \varphi_C, \psi = \gamma - \varphi_C + \varphi_B \implies</cmath>
+<cmath>\theta = 180^\circ - \gamma - \Theta, \psi = 180^\circ - \alpha - \Psi.</cmath>
+By applying the law of sines, we get
+<cmath>\frac {BE}{\sin \varphi_C} = \frac {BC}{\sin \Psi}, \frac {BD}{\sin (\alpha - \varphi_A)} = \frac {AB}{\sin \Theta}, \frac {BC}{\sin \alpha} = \frac {AB}{\sin \gamma}.</cmath>
+<cmath>ED = BD - BE \implies DE \cdot \sin \Theta \cdot \sin \Psi = \frac {AB}{\sin \gamma} \left ( \sin \gamma \cdot \sin (\alpha -  \varphi_A) \cdot \sin \Psi - \sin \alpha \cdot \sin \varphi_C \cdot \sin \Theta \right )</cmath>
+<cmath>\frac {BE'}{\sin (\gamma - \varphi_C)} = \frac {BC}{\sin \psi}, \frac {BD'}{\sin \varphi_A} = \frac {AB}{\sin \theta}.</cmath>
+<cmath>D'E' = BE' - BD' \implies D'E' \cdot \sin \theta \cdot \sin \psi = \frac {AB}{\sin \gamma} (\sin \alpha \cdot \sin (\gamma -  \varphi_C) \cdot \sin \theta - \sin \gamma \cdot \sin \varphi_A \cdot \sin \psi ).</cmath>
+We need to prove that
+<cmath>\sin \gamma \cdot \sin (\alpha -  \varphi_A) \cdot \sin \Psi - \sin \alpha \cdot \sin \varphi_C \cdot \sin \Theta =  \sin \alpha \cdot \sin (\gamma -  \varphi_C) \cdot \sin \theta - \sin \gamma \cdot \sin \varphi_A \cdot \sin \psi</cmath>
+We make the transformations:
+<cmath>\sin \alpha \left[ \sin \varphi_C \cdot \sin \Theta + \sin (\gamma -  \varphi_C) \cdot \sin (\gamma + \Theta) \right] = \sin \gamma \left[ \sin (\alpha -  \varphi_A) \cdot \sin \Psi + \sin \varphi_A \cdot \sin (\alpha + \Psi) \right]</cmath>
+<cmath>\sin \alpha \left[ \sin \varphi_C \cdot \sin \Theta + (\sin \gamma \cos \varphi_C - \cos \gamma \sin \varphi_C) \cdot (\sin \gamma \cos \Theta + \cos \gamma \sin \Theta \right] =</cmath>
+<cmath>= \sin \gamma \left[ \sin \alpha \cos \varphi_A \cdot \sin \Psi - \cos \alpha \sin \varphi_A \cdot \sin \Psi + \sin \varphi_A \cdot \sin \alpha \cos \Psi + \sin \varphi_A \cdot \cos \alpha \sin \Psi \right]</cmath>
+<cmath>\sin \alpha \left[ \sin \varphi_C \cdot \sin \Theta + \sin^2 \gamma \cos \varphi_C \cos \Theta - \cos ^2 \gamma \sin \varphi_C \cdot \sin \Theta + \cos \gamma \sin \Theta + \sin \gamma \cos \gamma (\cos \varphi_C \sin \Theta - \sin \varphi_C \cos \Theta) \right] =</cmath>
+<cmath>= \sin \gamma \cdot \sin \alpha \left[\cos \varphi_A \cdot \sin \Psi +  \sin \varphi_A \cdot \cos \Psi \right]</cmath>
+<cmath>\sin \alpha \left[ \sin^2 \gamma \cdot \cos (\Theta - \varphi_C) +  \sin \gamma \cdot \cos \gamma \cdot \sin (\Theta - \varphi_C)\right] = \sin \gamma \cdot \sin \alpha \cdot \sin (\Psi +  \varphi_A)</cmath>
+<cmath>\sin \alpha \cdot \sin \gamma \cdot \sin (\gamma + \Theta - \varphi_C) = \sin \gamma \cdot \sin \alpha \cdot \sin (\beta +  \varphi_A -  \varphi_B +  \varphi_C).</cmath> The last statement is obvious.
+'''vladimir.shelomovskii@gmail.com, vvsss'''
 ==2024, Problem 19==
 [[File:2024 19 4.png|250px|right]]
@@ Line 146: / Line 455: @@
 '''vladimir.shelomovskii@gmail.com, vvsss'''
+==2024, Problem 17==
+[[File:2024 17 1.png|400px|right]]
+Let <math>\triangle ABC</math> be not isosceles triangle, <math>\omega</math> be its incircle.
+Let <math>D, E,</math> and <math>F</math> be the points at which the incircle of <math>\triangle ABC</math> touches the sides <math>BC, CA,</math> and <math>AB,</math> respectively.
+Let <math>K</math> be the point on ray <math>EF</math> such that <math>EK = AB.</math>
+Let <math>L</math> be the point on ray <math>FE</math> such that <math>FL = AC.</math>
+The circumcircles of <math>\triangle BFK</math> and <math>\triangle CEL</math> intersect <math>\omega</math> again at <math>Q</math> and <math>P,</math> respectively.
+Prove that <math>BQ, CP,</math> and <math>AD</math> are concurrent.
+<i><b>Proof</b></i>
+<math>\frac {KE} {FL} = \frac {AB}{AC},</math> so points <math>Q,P,</math> and <math>G = FE \cap BC</math> are collinear (see [[Symmetry | Symmetry and incircle]] for details).
+Therefore lines <math>BQ, CP,</math> and <math>AD</math> are concurrent (see [[ Symmetry | Symmetry and incircle A]] for details.)
+'''vladimir.shelomovskii@gmail.com, vvsss'''
 ==2024, Problem 16==
 [[File:2024 16 1.png|300px|right]]
@@ Line 173: / Line 504: @@
 '''vladimir.shelomovskii@gmail.com, vvsss'''
+==2024, Problem 15==
+[[File:2024 15.png|390px|right]]
+The difference of two angles of a triangle is greater than <math>90^\circ.</math> Prove that the ratio of its circumradius and inradius is greater than <math>4.</math>
+<i><b>Proof</b></i>
+Suppose, <math>\angle BAC = \alpha = \angle ACB + 90^\circ =  \gamma + 90^\circ.</math>
+Let <math>\Omega = \odot ABC, C'</math> be the point on <math>\Omega</math> opposite <math>C, B'</math> be the midpoint of arc <math>\overset{\Large\frown} {AC}.</math>
+Then <cmath>\angle CAC' = 90^\circ \implies \angle BAC' = \angle ACB = \gamma = \angle BCC' \implies</cmath>
+<cmath>\overset{\Large\frown} {AB} = \overset{\Large\frown} {BC'} \implies BB' || CC'.</cmath>
+<cmath>BO = CO \implies \angle CBO = \angle OCB = \angle ACB = \gamma.</cmath>
+<cmath>\angle ABC = \beta = 180^\circ - \gamma - \alpha =  90^\circ - 2 \gamma.</cmath>
+<cmath>\angle OBB' = \frac {\beta}{2} + \gamma =  45^\circ.</cmath>
+<cmath>BO = OB' = R \implies \angle BB'O =  45^\circ, \angle BOB' = 90^\circ.</cmath>
+Incenter <math>I</math> triangle <math>\triangle ABC</math> lies on <math>BB',</math> therefore <math>OI \ge \frac {R}{\sqrt {2}}.</math>
+We use the Euler law <math>OI^2 = R^2 - 2 Rr \implies \frac {2r}{R} = 1 - \frac {OI^2}{R^2} \le \frac{1}{2} \implies \frac{R}{r} \ge 4.</math>
+If <math>R = 4r</math> then <math>OI \perp BB' \implies \frac {BI}{IB'} = 1 \implies \frac {BC + AB}{AC} = 2, BI = \frac{R}{\sqrt{2}}.</math>
+<cmath>\sin CBI = \frac {r}{BI} = \frac{r \sqrt{2}}{R} = \frac{1}{2\sqrt{2}} \implies \sin \beta = \frac{\sqrt{7}}{4} \implies</cmath>
+<cmath>AC = 2R \sin \beta = R \frac {\sqrt{7}}{2},AB = AC (1 - \frac{1}{\sqrt {7}}), BC = AC (1 + \frac{1}{\sqrt {7}}).</cmath>
+If <math>\angle BAC' > \angle ACB \implies \frac {OI}{R}</math> increases so <math>\frac {r}{R}</math> decreases.
+'''vladimir.shelomovskii@gmail.com, vvsss'''
+==2024, Problem 14==
+[[File:2024 14 1.png|390px|right]]
+The incircle <math>\omega</math> of a right-angled triangle <math>\triangle ABC</math> touches the circumcircle <math>\theta</math> of its medial triangle at point <math>F.</math> Let <math>OE</math> be the tangent to <math>\omega</math> from the midpoint <math>O</math> of the hypothenuse <math>AB</math> distinct from <math>AB.</math> Prove that <math>CE = CF.</math>
+<i><b>Proof</b></i>
+Let <math>\Omega</math> and <math>I</math> be the circumcircle and the incenter of <math>\triangle ABC, D = \omega \cap AB.</math>
+Let <math>Q</math> be nine-point center of <math>\triangle ABC, G</math> be the point at <math>OB</math> such that <math>OG = DO, K \in DI, KQ ||AB.</math>
+Denote <math>r = ID, R = AO,a = BC, b = AC,c= AB, \beta = \angle ABC.</math>
+<math>\triangle ABC</math> is the right-angled triangle, so <math>Q</math> is the midpoint <math>CO,</math>
+<cmath>2R = c, r = \frac {a+b-c}{2},FQ = \frac{R}{2}.</cmath>
+Let <math>h(X)</math> be the result of the homothety of the point <math>X</math> centered in <math>C</math> with the coefficient <math>2.</math> Then
+<cmath>h(\theta) = \Omega, F' = h(F) \in \Omega, O = h(Q) \implies FQ || F'O.</cmath>
+<cmath>DO = EO = GO \implies \angle DEG = 90^\circ.</cmath>
+<cmath>\angle DOE = 2 \angle DOI = 2 \angle EGD \implies  \angle DOI = \angle EGD, IO || EG.</cmath>
+WLOG, <math>a > b \implies DO = \frac {a-b}{2} = \tan IOD = \frac{r}{DO} = \frac{a+b-c}{a-b} = \frac{\cos\beta + \sin \beta -1}{\cos\beta - \sin \beta}.</math>
+Let <math>H</math> be the foot from <math>C</math> to <math>\overline{AB}</math>.
+<math>CH = a \sin \beta = c \sin \beta \cos \beta, BH = a \cos \beta, BG = AD = \frac{c+b-a}{2}.</math>
+<cmath>\tan \angle CGH = \frac {CH}{BH - BG} = \frac{2 \sin \beta \cos \beta}{2 \cos^2 \beta +\cos \beta - \sin \beta -1} =</cmath>
+<cmath>=  \frac{(\sin \beta + \cos \beta -1)(\sin \beta + \cos \beta +1)}{(\cos \beta - \sin \beta)(\sin \beta + \cos \beta +1)} = \frac{\cos\beta + \sin \beta -1}{\cos\beta - \sin \beta} = \tan IOD.</cmath>
+Therefore points <math>C,E,</math> and <math>G</math> are collinear.
+<cmath>\psi = \angle BCG = \angle AGC - \angle ABC \implies</cmath>
+<cmath>\tan \psi = \frac {\tan \angle AGC - \tan \angle ABC}{1 + \tan \angle AGC \cdot \tan \angle ABC} = \frac {c - a}{c-b}.</cmath>
+<cmath>\sin 2\psi = \frac {2\tan \psi}{1 + \tan^2 \psi} = \frac {2(c-a)(c-b)}{c(3c - 2b - 2a)},</cmath>
+<cmath>\angle AOF' = \angle KQI, \sin  \angle KQI = \frac{CH/2 -r}{QF - r}.</cmath>
+<cmath>4c(QF - r) = c(c -2a -2b +2c) = c(3c -2a -2b),</cmath>
+<cmath>2c(CH - 2r) = 2(ab -ac -bc +c^2) = 2(c - a)(c - b),</cmath>
+<cmath>\sin \angle KQI =  \frac {2(c-a)(c-b)}{c(3c - 2b - 2a)}= \sin 2 \psi \implies  \angle KQI = 2 \psi.</cmath>
+<cmath>\angle ACF' = \frac {\angle AOF'}{2} = \frac {\angle KQI}{2} = \psi = \angle BCG \implies \angle FCI = \angle ECI \implies CF = CE.</cmath>
+'''vladimir.shelomovskii@gmail.com, vvsss'''
 ==2024, Problem 12==
 [[File:2024 12.png|390px|right]]
@@ Line 249: / Line 642: @@
 '''vladimir.shelomovskii@gmail.com, vvsss'''
 ==2024, Problem 2==
-[[File:2024 2.png|390px|right]]
+[[File:2024 2.png|330px|right]]
-[[File:2024 2a.png|390px|right]]
+[[File:2024 2a.png|330px|right]]
-[[File:2024 2b.png|390px|right]]
+[[File:2024 2b.png|330px|right]]
 Three distinct collinear points are given. Construct the isosceles triangles such that these points are their circumcenter, incenter and excenter (in some order).